1. Better than squareroot cancellation for multiplicative functions - Adam Harper [2017]

Adam Harper (University of Warwick)

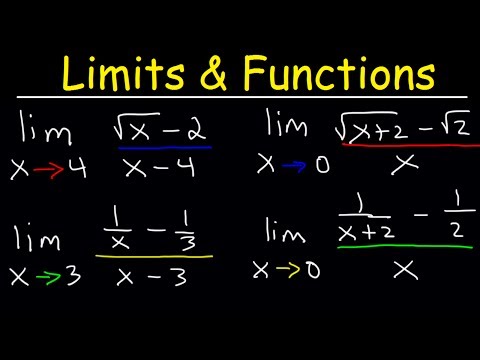
It is a standard heuristic that sums of oscillating number theoretic functions, like the M\"obius function or Dirichlet characters, should exhibit squareroot cancellation. It is often very difficult to prove anything as strong as that, and we generally expect that if we could prove squareroot cancellation it would be the best possible bound. I will discuss recent results showing that, in fact, certain averages of multiplicative functions exhibit a bit more than squareroot cancellation

MAY 01, 2017
MONDAY