filmov
tv
Absolute Value Definition of a Bounded Sequence | Real Analysis
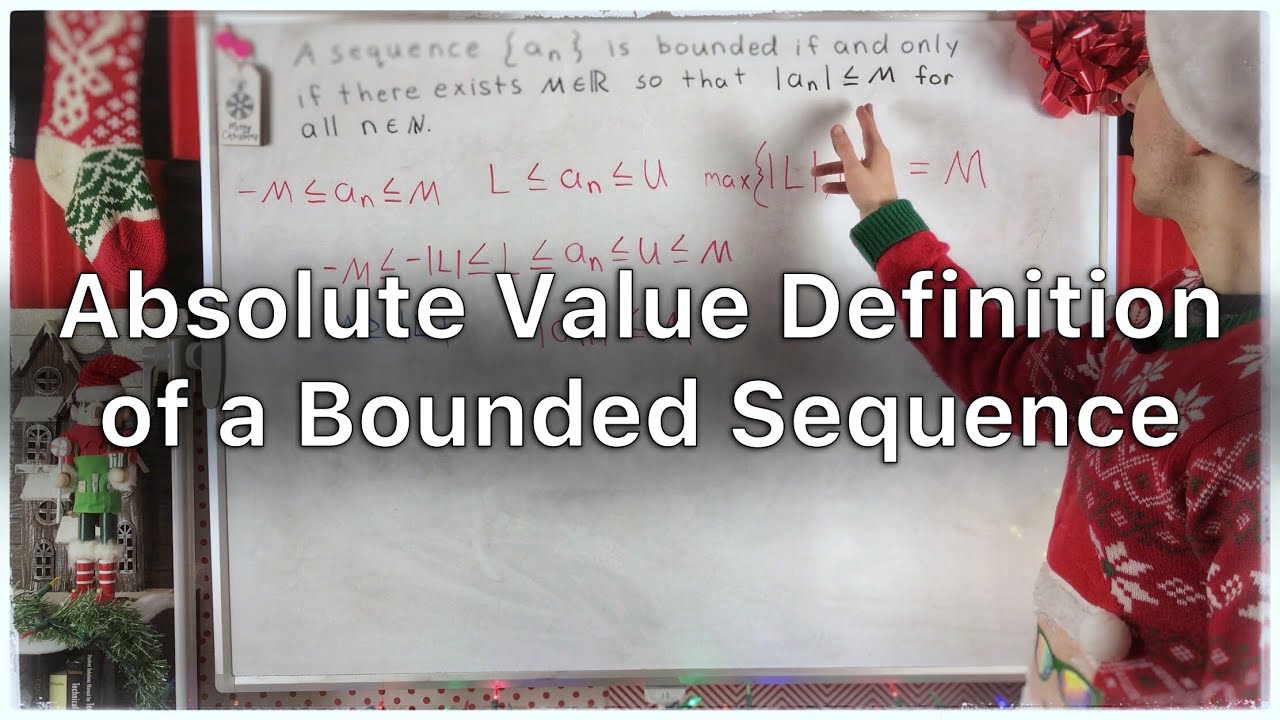
Показать описание
The definition of a bounded sequence is a very important one, and it relies on a sequence having a lower an upper bound. However, we can also state the definition of a bounded sequence with only a single bound - namely an upper bound on the absolute value of the terms of the sequence. If there exists a real number that is greater than or equal to the absolute value of every term in a sequence, then the sequence is bounded, and the converse is true as well. As in: if a sequence is bounded then there exists a real number greater than or equal to the absolute value of every term in the sequence. We prove this equivalent definition of a bounded sequence in today's real analysis video lesson!
★DONATE★
Thanks to Robert Rennie, Barbara Sharrock, and Lyndon for their generous support on Patreon!
Follow Wrath of Math on...
★DONATE★
Thanks to Robert Rennie, Barbara Sharrock, and Lyndon for their generous support on Patreon!
Follow Wrath of Math on...
Meaning of absolute value
What is Absolute Value? | Absolute Value Examples | Math with Mr. J
What is Absolute Value? (1 of 3: The Simplest Definition)
How To Solve Absolute Value Equations, Basic Introduction, Algebra
Math Antics - Absolute Value
What is Absolute Value? (Concept) | Don't Memorise
Definition of Absolute Value Intervals : Math Definitions & More
Absolute Values: Defining, Calculating, and Graphing
Matrix Multiplication, Transposition, Matrix Representation of a Vector, Vector Rotation
What is the definition of absolute value
Absolute value definition
What is Absolute Value of a Number / Absolute Value of Integers
What is Absolute Value? | Math with Mr. J #Shorts
Absolute Values in a Nutshell: GRE / GMAT Quant Explained (with official questions)
What is Absolute Value? (3 of 3: The Geometric Definition)
Absolute Value - Understand In 10 Minutes
Integers and Absolute Values
2.4 Distance and absolute values
01 - What is Absolute Value and Negative Numbers?
Absolute Value Video Lesson
Concept of Absolute Value by Waqas Nasir
What is Absolute Value? | Don't Memorise
What is the Absolute Value of a Number?
Absolute Values Made Easy! #mathtutor #absolutevalue #puzzle
Комментарии
 0:03:40
0:03:40
 0:02:51
0:02:51
 0:04:49
0:04:49
 0:04:21
0:04:21
 0:13:15
0:13:15
 0:04:45
0:04:45
 0:01:54
0:01:54
 0:05:10
0:05:10
 0:25:01
0:25:01
 0:03:49
0:03:49
 0:02:28
0:02:28
 0:04:12
0:04:12
 0:00:53
0:00:53
 0:29:38
0:29:38
 0:04:38
0:04:38
 0:11:44
0:11:44
 0:08:13
0:08:13
 0:03:31
0:03:31
 0:11:02
0:11:02
 0:03:54
0:03:54
 0:06:47
0:06:47
 0:02:19
0:02:19
 0:00:54
0:00:54
 0:00:30
0:00:30