filmov
tv
What Is the Laplacian?

Показать описание
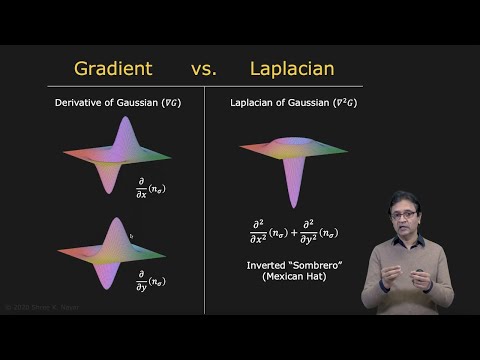
Laplacian intuition
What Is the Laplacian?
Laplacian of a scalar or vector field | Lecture 20 | Vector Calculus for Engineers
What does the Laplace Transform really tell us? A visual explanation (plus applications)
Laplacian intuition
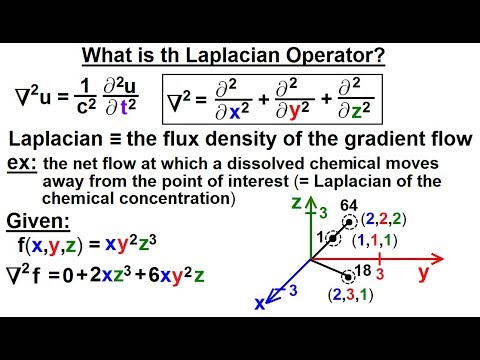
Laplacian computation example
Why the Laplace Transform?
Intro to the Laplace Transform & Three Examples
Calculus 3: Divergence and Curl (22 of 32) [ERROR IN THIS VIDEO] What is the Laplace Operator?
Edge Detection Using Laplacian | Edge Detection
Laplace Transform Explained and Visualized Intuitively
The Laplace Transform - A Graphical Approach
Calculus 3: Divergence and Curl (23 of 32) The Laplace Operator: Ex. 1
Laplacian of a vector | Laplacian operator | Laplacian of a Rectangular Cylindrical Spherical
How to solve differential equations
Laplace Equation
Laplace's Equation and Poisson's Equation
Laplacian operator:Concept, example,properties.Connection with Gradient operator(see pinned comment)
Physics - Advanced E&M: Ch 1 Math Concepts (13 of 55) What is the Laplacian of a Scalar (Field)?
Math: Partial Differential Eqn. - Ch.1: Introduction (14 of 42) Understanding the Laplacian Operator
what is laplacian filterlaplacian matrix
Math: Partial Differential Eqn. - Ch.1: Introduction (13 of 42) What is the Laplacian Operator?
DIP#20 The Laplacian filter- Use of second order derivatives for enhancement || EC Academy
What is...the Laplace matrix?
Комментарии
 0:05:31
0:05:31
 0:20:37
0:20:37
 0:06:51
0:06:51
 0:20:25
0:20:25
 0:05:31
0:05:31
 0:05:21
0:05:21
 0:02:59
0:02:59
 0:12:05
0:12:05
 0:02:36
0:02:36
 0:12:39
0:12:39
 0:19:55
0:19:55
 0:13:24
0:13:24
 0:02:34
0:02:34
 0:10:20
0:10:20
 0:00:46
0:00:46
 0:13:17
0:13:17
 0:17:55
0:17:55
 0:12:20
0:12:20
 0:08:18
0:08:18
 0:06:06
0:06:06
 0:04:16
0:04:16
 0:07:54
0:07:54
 0:05:36
0:05:36
 0:11:34
0:11:34