Math: Partial Differential Eqn. - Ch.1: Introduction (14 of 42) Understanding the Laplacian Operator

Показать описание

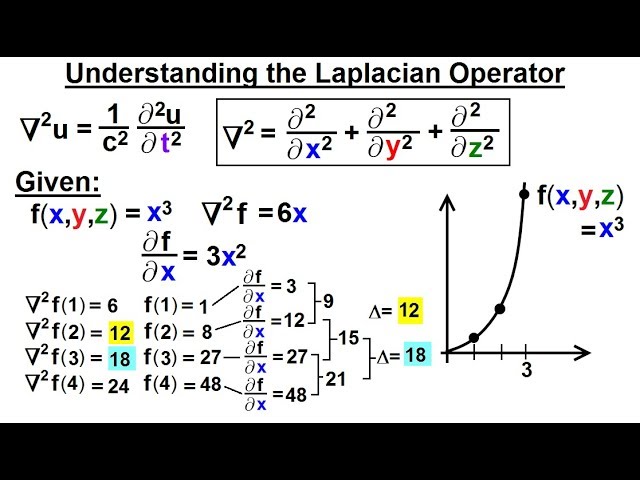
In this video I will explain what is a Laplacian operator by simplifying the function to a single dimension where f(x,y,z)=x^3.

Next video in this series can be seen at:

Рекомендации по теме

Комментарии

Excellent explanation. Thank you Professor.

valeriereid

Michel, It would be really nice if you could do GRE and if possible MCAT videos. Furthermore if you ever are able to have time, one key thing that would be nice is to incorporate interactivity somehow so perhaps someone could work out problems related to each lecture video or at the end of each subject. There is a lot of value in learning from doing. You are changing lives for the better, I thank you again.

Jarrod_C

I thought the second derivative actually tells us the curvature of the function. In 3D it means that out gradient field is curved up or down. So we can understand where things are accumulating and where things are draining. Right?

masoudjafary

Hard to wrap your head around this, but you did a very good job of explaining it.

Would your consider it similar to first and second derivatives of position, which are velocity and acceleration?

schifoso

What is the use of knowing the average of change in the slope. Where v use this function

sainathreddyvarikuti

So, I conclude that the Laplacian is kind of acceleration.

davidkwon

Math: Partial Differential Eqn. - Ch.1: Introduction (14 of 42) Understanding the Laplacian Operator

But what is a partial differential equation? | DE2

Math: Partial Differential Eqn. - Ch.1: Introduction (19 of 42) First Order PDE: Example 1

Partial Differential Equations Overview

How to find Partial Derivative of a function

Math: Partial Differential Eqn. - Ch.1: Introduction (1 of 42) What is a Partial Differential Eqn?

Oxford Calculus: Solving Simple PDEs

Advice for Learning Partial Differential Equations

Learning Partial Differential Equations

Math ➖ IV (BAS303/BAS403), Unit -I, lecture 18, partial differential equations,#math4, #bas303

Math: Partial Differential Eqn. - Ch.1: Introduction (7 of 42) Is the Function a Solution of PDE?

🔵01 - Differential Equations, Order, Degree, Ordinary and Partial Differential Equation

Lecture 1 || Introduction to Partial Differential Equations||

Solving a partial differential equation using laplace transforms

First Order Partial Differential Equation

12.1: Separable Partial Differential Equations

Partial Differential Equations by Walter Strauss #shorts

Math: Partial Differential Eqn. - Ch.1: Introduction (38 of 42) The Diffusion Equation (Part 1 of 5)

Difference Between Partial and Total Derivative

Partial Differential Equations

Math: Partial Differential Eqn. - Ch.1: Introduction (32 of 42) 2nd: Using Boundary Conditions

Oxford Calculus: Separable Solutions to PDEs

Differential Equations Book for Beginners

Partial Differential Equations in Engineering Problems by Miller #shorts

Differential equations, a tourist's guide | DE1