Proof: Every Tournament has Hamiltonian Path | Graph Theory

Показать описание

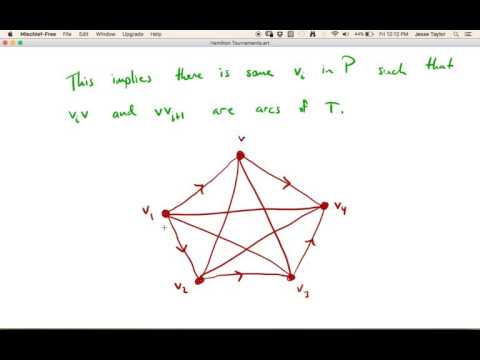
We prove that every tournament graph contains a Hamiltonian path, that is a path containing every vertex of the graph. Recall a tournament is a directed graph with exactly one arc between each pair of vertices. The proof will proceed by contradiction, and follow a similar format to other proofs we have seen related to Hamiltonian paths, Hamiltonian cycles, and Hamiltonian graphs. #GraphTheory

★DONATE★

Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!

Follow Wrath of Math on...

Рекомендации по теме

Комментарии

Support the production of this course by joining Wrath of Math as a Channel Member for exclusive and early videos, original music, and upcoming lecture notes for the graph theory series! Plus your comments will be highlighted for me so it is more likely I'll answer your questions!

WrathofMath

please keep making these videos you have no idea how much you are helping us. even the dumbest bloke like me😃 could easily understand your explanation. THANK YOU SO MUCH

mohammedseidbushira

Thank for this video, could you please explain the proof of Eulerian Trail Theorem?

mohammadrezajavadi

The fact that every tournament has a Hamiltonian Path didn't automatically seem self-evident to me. Thanks for providing a really nice explanation as always 🙏

PunmasterSTP

Why does this switch has to happen? I don't get it

dark_noone

I should say that, I‘m still a bit confused that, why the directions of (v, v_i) and (v, v_{i+1}) should be different...

carollove

Nice classes sir, it is really helpful

devupr

1:45 longest path
5:06 V, Vk; From V

ridwanulhasantanvir

Great explanation! I love Graph theory proofs. My main takeaway, however, was that for a problem I’m currently working on, I’ve been thinking about Hamiltonian cycles as opposed to paths. Turns out that last edge makes a big difference :)

colecharb

Love it! I didn't know anything about tournaments before watching your videos, so this has been a lot of fun.

mike_the_tutor

Proof: Every Tournament has Hamiltonian Path | Graph Theory

Proof: Every Tournament has Hamiltonian Path | Graph Theory

Intro to Tournament Graphs | Graph Theory

Hamiltonian Graph with examples | Hamiltonian Path & Circuit

Hamilton Tournaments - 20

Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths

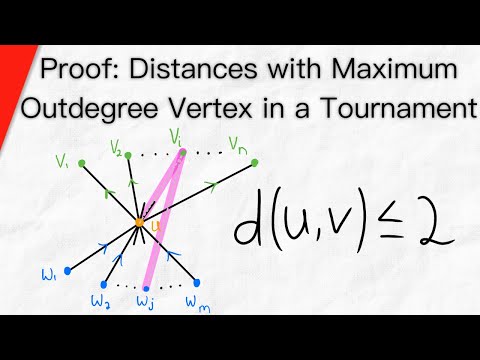
Proof for Distances from Tournament's Maximum Outdegree Vertex | Graph Theory

Hamiltonian Cycles, Graphs, and Paths | Hamilton Cycles, Graph Theory

Section 5.1 Tournaments & Directed Graphs Video Lecture

What is a Hamilton path?

Graph Theory - Tournament

Hamiltonian Graph | Hamiltonian path | Hamiltonian circuit | Graph theory

Proof: Vertices of Strong Tournament Lie on Triangles | Graph Theory

Digraphs 5 - Hamiltonian

7.2 Hamiltonian digraphs

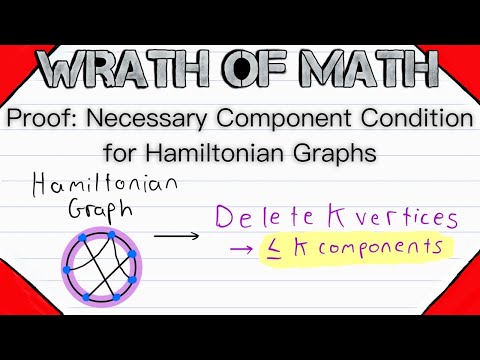
Proof: Necessary Component Condition for Hamiltonian Graphs | Graph Theory

Math 432: Graph Theory - Hamiltonian Cycles (3 of 3)

Proof: Tournament is Transitive iff it has No Cycles | Graph Theory

Discrete Mathematics #25 Graph Theory: Tournament Problem (2/2)

Discrete Math - A Sufficient Condition for Hamiltonicity

Graph Theory | Eulerian Graph & Hamiltonian Graph - Walk,Trail,Path | Discrete Mathematics by GP...

10. Hamilton Path | Hamilton Circuit | Hamilton graph Examples of Hamilton path and Hamilton circuit

Tournament graph

V6GTU1S11 Properties of hamiltonian graphs

Sperner's Lemma: A little combinatorial result