filmov
tv
The geometric view on orthogonal projections
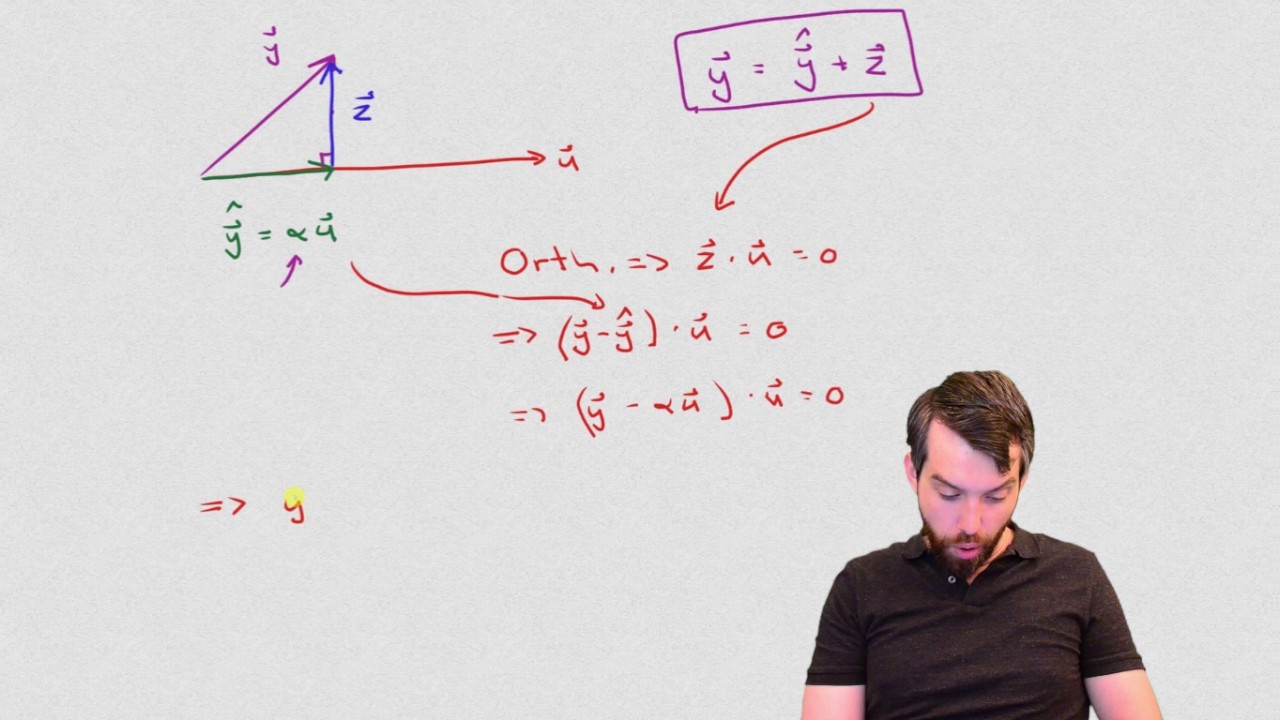
Показать описание
Learning Objectives:
1) Given a vector, compute the orthogonal projection onto another vector
This video is part of a Linear Algebra course taught by Dr. Trefor Bazett at the University of Cincinnati
1) Given a vector, compute the orthogonal projection onto another vector
This video is part of a Linear Algebra course taught by Dr. Trefor Bazett at the University of Cincinnati
The geometric view on orthogonal projections
9 Regression as an Orthogonal Projection
What is an Orthogonal Projection?
The beautiful geometric view of FOURIER SERIES // The Linear Algebra Perspective
Linear Algebra 6.2.2 Orthogonal Projections
First angles vs Third angle method | Orthographic projections animation
Orthogonal Projection - How to Present Top, Front and Side Views
Orthogonal Projection - Why 3 Views is Enough
Orthographic projection - Engineering drawing - Technical drawing
Orthogonality and Orthogonal Projection
Orthographic Projection - Engineering drawing - Technical drawing
Orthogonal Matrices - Geometric Interpretation
Beginning Orthographic Projection
Orthogonal projection
03 parallel und orthogonal
Orthogonal Projection #shorts
Orthogonal projection of a vector | Lecture 25 | Matrix Algebra for Engineers
These are orthogonal #shorts
Orthogonal Drawing 1: Introduction
CDT G10/11 - First Angle Orthographic Projection Part 1
Orthogonal projections - Platonic solids
Isometric View | How to Construct an Isometric View of an Object | Example: 4
Showing Cross Product is Orthogonal
How to find orthogonal vectors
Комментарии
 0:11:07
0:11:07
 0:08:36
0:08:36
 0:01:53
0:01:53
 0:16:03
0:16:03
 0:08:45
0:08:45
 0:06:13
0:06:13
 0:01:55
0:01:55
 0:05:23
0:05:23
 0:08:31
0:08:31
 0:27:27
0:27:27
 0:12:17
0:12:17
 0:06:03
0:06:03
 0:05:06
0:05:06
 0:00:38
0:00:38
 0:00:56
0:00:56
 0:00:59
0:00:59
 0:10:53
0:10:53
 0:00:58
0:00:58
 0:06:59
0:06:59
 0:04:08
0:04:08
 0:00:16
0:00:16
 0:09:20
0:09:20
 0:00:40
0:00:40
 0:00:21
0:00:21