filmov
tv
Change of Variables

Показать описание
The Jacobian
Change of Variables & The Jacobian | Multi-variable Integration
Change of Variables and the Jacobian
Change of variables (double integral and the Jacobian) | Lecture 31 | Vector Calculus for Engineers
Change of Variables
Change of Variables in Multiple Integrals: Double Integral Transformation with Jacobian
Integration by change of variables
Substitution rule, transformation formula, change of variables
Probability Change Of Variable: Theory (Part 1)
INTEGRAL por Cambio de Variable TRIGONOMÉTRICO | Usa el 'TRUCO' del Teorema de Pitágoras 🤫...
Example of a Change of Variables for a Double Integral: Jacobian
Definite integral by change of variable
What do you mean by variable change ?
Calculus 3 Lecture 14.8: How to Change Variables in Multiple Integrals (Using the Jacobian)
What is Jacobian? | The right way of thinking derivatives and integrals
CHANGE OF VARIABLES | DIFFERENTIAL EQUATION
Separable First Order Differential Equations - Basic Introduction
Change in Variable | Jacobian | Multiple Integration | Numerical | Maths
CHANGE OF VARIABLES IN DOUBLE INTEGRALS | CARTESIAN INTO POLAR | (x, y) INTO (u, v) | ENG. MATHS
Change of variable to solve a differential equations (KristaKingMath)
Calc 3 15.9 Notes: Change of Variables in Multiple Integrals
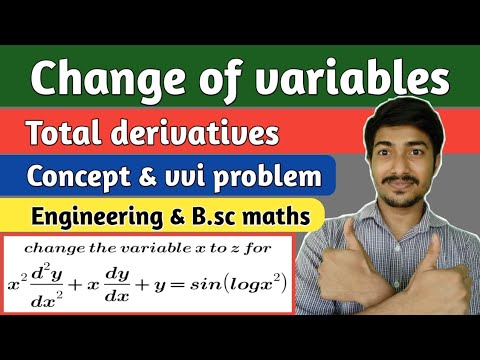
change of variables | total derivatives | engineering maths | bsc maths
Calculus 3: Change of Variables in Multiple Integrals & the Jacobian (Video #26)| Math w Profess...
How to solve PDE via change of variables
Double Integral through a Change of Coordinates (the Jacobian)
Комментарии
 0:10:07
0:10:07
 0:13:08
0:13:08
 0:10:46
0:10:46
 0:16:41
0:16:41
 0:11:40
0:11:40
 0:09:33
0:09:33
 0:12:15
0:12:15
 0:09:03
0:09:03
 0:14:54
0:14:54
 0:08:00
0:08:00
 0:10:52
0:10:52
 0:01:16
0:01:16
 1:40:05
1:40:05
 0:27:14
0:27:14
 0:07:01
0:07:01
 0:10:42
0:10:42
 0:18:53
0:18:53
 0:56:46
0:56:46
 0:05:06
0:05:06
 0:24:30
0:24:30
 0:13:31
0:13:31
 1:01:12
1:01:12
 0:09:18
0:09:18
 0:10:25
0:10:25