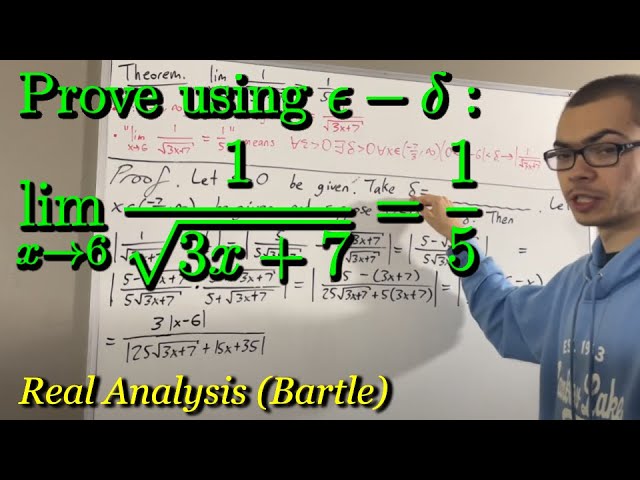
Prove the limit of 1/sqrt(3x+7) = 1/5 as x approaches 6 (using epsilon-delta) (ILIEKMATHPHYSICS)

preview_player
Показать описание
This video references the book "Introduction to Real Analysis" by Bartle and Sherbert (Fourth Edition). For more details related to the types of exercises covered in this video, see section 4.1.

Thanks and enjoy the video!

  1. ILIEKMATHPHYSICS
Рекомендации по теме
Prove the limit 0:08:34

Prove the limit of 1/sqrt(3x+7) = 1/5 as x approaches 6 (using epsilon-delta) (ILIEKMATHPHYSICS)

Proof of a 0:09:17

Proof of a Limit Value Using Epsilon and Delta

how to easily 0:08:28

how to easily write the epsilon-delta proofs for limits

Epsilon delta limit 0:09:55

Epsilon delta limit (Example 7): 1/x

This is a 0:00:48

This is a very famous limit

Epsilon delta limit 0:04:03

Epsilon delta limit (Example 6): Square Roots

Learn how to 0:01:40

Learn how to evaluate a limit at infinity

Examples on Epsilon 0:16:35

Examples on Epsilon Delta Definition of Limit (for 1 variable functions)

Calculus 1 Exam 3:10:17

Calculus 1 Exam Review Part8

When mathematicians get 0:00:37

When mathematicians get bored (ep1)

How REAL Men 0:00:35

How REAL Men Integrate Functions

How To Find 0:13:14

How To Find The Limit At Infinity

limits with epsilon-delta 0:15:21

limits with epsilon-delta definition! (linear, square root, and quadratic examples)

5 simple unsolvable 0:00:50

5 simple unsolvable equations

Do you remember 0:00:11

Do you remember the square roots of these perfect squares? 🤯 #Shorts #math #maths #mathematics

Limits of Trigonometric 0:15:23

Limits of Trigonometric Functions

Evaluating Limits at 0:04:53

Evaluating Limits at Infinity of Rational Functions with Square Roots (Example)

Proof that f(x) 0:09:56

Proof that f(x) = 1/x is Continuous on (0, infinity) using Delta-Epsilon

AP Scores are 0:00:51

AP Scores are out today

This chapter closes 0:00:16

This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation

Finding a Delta 0:13:41

Finding a Delta Given an Epsilon

Limits at Infinity 0:19:24

Limits at Infinity & Horizontal Asymptotes

Functions IIT Questions 0:00:48

Functions IIT Questions NO 11 ( X Class)

Solving Limits Mentally 0:01:00

Solving Limits Mentally #calculus #limit #infinity #rational

Комментарии
Автор

haha nice. Just getting into proofs and i love your channel

Artemis
Автор

It can be done more easily. The function f(x)=1/sqrt(3x+7) is obviously continuous in a neighbourhood of 6. But then lim_{x->6}f(x) =
1/sqrt(3(lim_{x->6})+7) = 1/sqrt(3*6+7)=1/5. The argument relies on the fact that lim_{x->y}f(x) = f(lim_{x->y}x) when f i contiuous at y.

kennethvalbjoern
Автор

How convenient that you got a |x-6|. If you had had, say |x-9| you would do |x - 6 -3| and apply triangle inequality to x - 6 and 3?

radadadadee