filmov
tv
Loop-the-loop physics problem: Forces on a vertical loop.
Показать описание
I solve the loop the loop first year undergraduate and AP physics problems
Loop-the-loop physics problem: Forces on a vertical loop.
Roller coaster loop the loop
Ch 7 - Circular Motion Loop The Loop Problem
Normal Force on a Hill, Centripetal Force, Roller Coaster Problem, Vertical Circular Motion, Physics
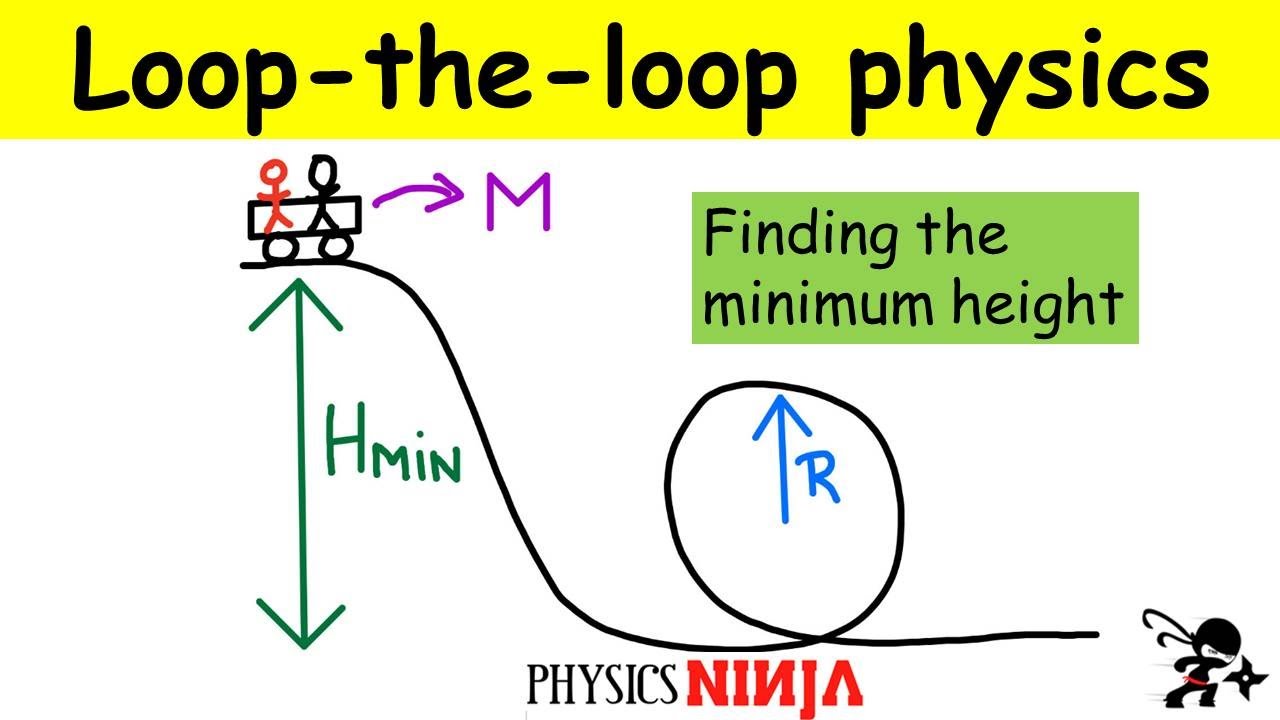
Loop-the-loop physics problem
Dynamics Demo: Loop the Loop
How to Complete Loop the Loop Physics!
Loop the Loop - Part A - Circular Motion Level 5
Physics Ka Important MCQs (chapter -4) class 12 #physicsclass12mcqs#neetpracticequestions #neet2025
Minimum Speed in Loop the Loop Problem
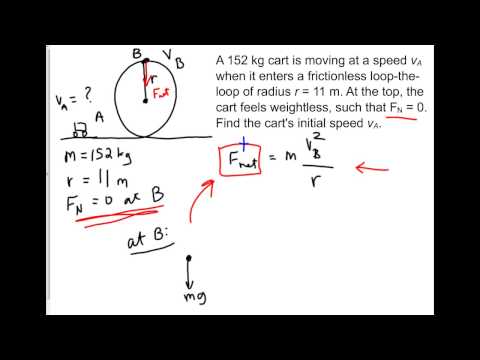
Physics problem: loop the loop. How fast must car go to complete the loop, and from what height?
Loop de loop answer part 1 | Centripetal force and gravitation | Physics | Khan Academy
Physics Tutorial: Physics of the Loop the Loop
2007 Physics Final Exam. Problem 7: Loop the Loop
7.3 Work Energy Problem Solving & Example 7.9 Loop the Loop
Classic Loop the Loop Problem from Mastering Physics
Riding a Loop - the - Loop
5 Examples of Solving Centripetal Force Problems - IB Physics
PP 6-11 Minimum Velocity Loop the Loop Problem
Chapter 5, Example #6 (Diavolo loop-the-loop)
Physics 206 - The Loop De Loop Problem
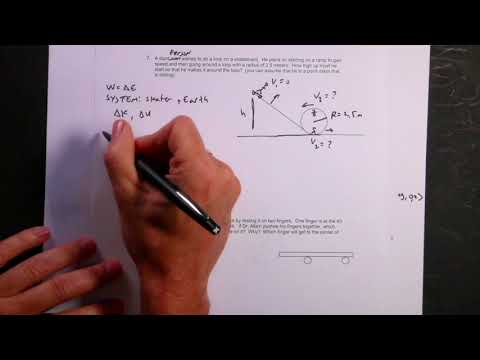
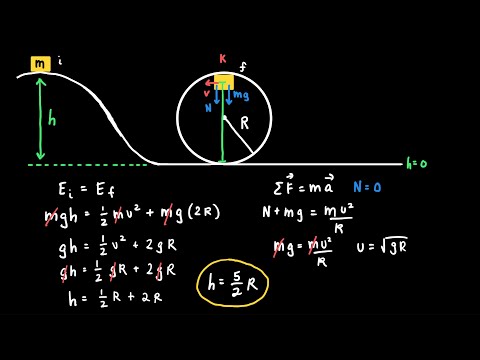
Conservation of energy, minimum height for the roller coaster loop, minimum speed for the loop.
Minimum Speed to Go Around a Loop
Why roller coaster loops aren't circular
Комментарии
 0:11:52
0:11:52
 0:11:10
0:11:10
 0:13:16
0:13:16
 0:16:00
0:16:00
 0:12:38
0:12:38
 0:02:48
0:02:48
 0:00:30
0:00:30
 0:01:05
0:01:05
 0:00:59
0:00:59
 0:02:40
0:02:40
 0:09:18
0:09:18
 0:06:05
0:06:05
 0:13:12
0:13:12
 0:07:11
0:07:11
 0:07:42
0:07:42
 0:03:47
0:03:47
 0:16:10
0:16:10
 0:21:08
0:21:08
 0:02:53
0:02:53
 0:04:47
0:04:47
 0:10:51
0:10:51
 0:04:57
0:04:57
 0:08:45
0:08:45
 0:06:27
0:06:27