filmov
tv
How to derive general relativity from Wolfram Physics with Jonathan Gorard
Показать описание
Here’s a masterclass from Jonathan Gorard.
One of the most compelling results to come out of the Wolfram Physics is Jonathan’s derivation of the Einstein equations from the hypergraph.
Whenever I hear anyone criticize the Wolfram model for bearing no relation to reality, I tell them this: Jonathan Gorard has proved that general relativity can be derived from the hypergraph.
In this excerpt from our conversation, Jonathan describes how making just three reasonable assumptions – causal invariance, asymptotic dimension preservation and weak ergodicity – allowed him to derive the vacuum Einstein equations from the Wolfram model.
In other words, the structure of space-time in the absence of matter more or less falls out of the hypergraph.
And making one further assumption – that particles can be treated as localized topological obstructions – allowed Jonathan to derive the non-vacuum Einstein equations from the Wolfram model.
In other words, the structure of space-time in the presence of matter, too, falls out of the hypergraph.
It’s difficult to overstate the importance of this result.
At the very least, we can say that the Wolfram model is consistent with general relativity.
To state it more strongly: we no longer need to take general relativity as a given; instead, we can derive it from Wolfram Physics.
—
Jonathan’s seminal paper on how to derive general relativity
Jonathan Gorard
People mentioned by Jonathan
Research mentioned by Jonathan
Concepts mentioned by Jonathan
From A Project to find the Fundamental Theory of Physics by Stephen Wolfram:
Images
—
Kootenay Village Ventures Inc.
One of the most compelling results to come out of the Wolfram Physics is Jonathan’s derivation of the Einstein equations from the hypergraph.
Whenever I hear anyone criticize the Wolfram model for bearing no relation to reality, I tell them this: Jonathan Gorard has proved that general relativity can be derived from the hypergraph.
In this excerpt from our conversation, Jonathan describes how making just three reasonable assumptions – causal invariance, asymptotic dimension preservation and weak ergodicity – allowed him to derive the vacuum Einstein equations from the Wolfram model.
In other words, the structure of space-time in the absence of matter more or less falls out of the hypergraph.
And making one further assumption – that particles can be treated as localized topological obstructions – allowed Jonathan to derive the non-vacuum Einstein equations from the Wolfram model.
In other words, the structure of space-time in the presence of matter, too, falls out of the hypergraph.
It’s difficult to overstate the importance of this result.
At the very least, we can say that the Wolfram model is consistent with general relativity.
To state it more strongly: we no longer need to take general relativity as a given; instead, we can derive it from Wolfram Physics.
—
Jonathan’s seminal paper on how to derive general relativity
Jonathan Gorard
People mentioned by Jonathan
Research mentioned by Jonathan
Concepts mentioned by Jonathan
From A Project to find the Fundamental Theory of Physics by Stephen Wolfram:
Images
—
Kootenay Village Ventures Inc.
Комментарии
 0:36:51
0:36:51
 0:06:09
0:06:09
 0:36:49
0:36:49
 0:12:55
0:12:55
 0:14:04
0:14:04
 0:07:29
0:07:29
 0:05:07
0:05:07
 0:05:28
0:05:28
 0:08:41
0:08:41
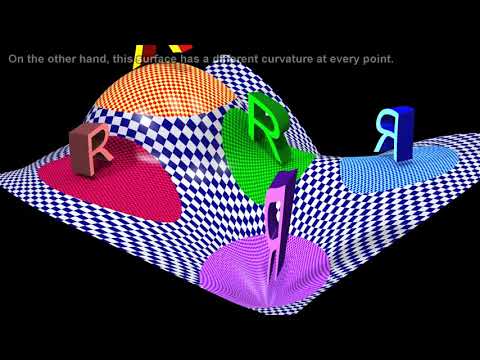 0:28:23
0:28:23
 0:02:11
0:02:11
 1:01:42
1:01:42
 0:34:41
0:34:41
 0:54:42
0:54:42
 0:36:21
0:36:21
 0:30:27
0:30:27
 0:07:27
0:07:27
 0:01:38
0:01:38
 0:11:39
0:11:39
 0:09:21
0:09:21
 0:05:38
0:05:38
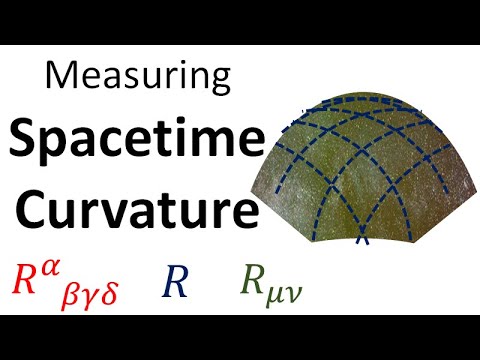 0:34:31
0:34:31
 0:47:54
0:47:54
 0:16:42
0:16:42