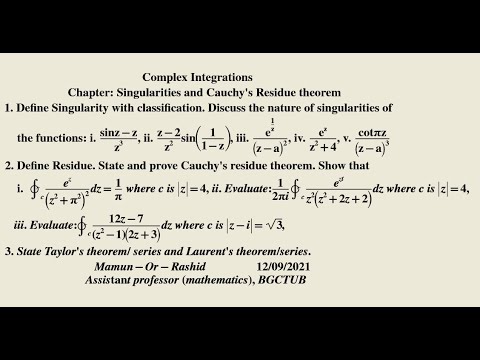
7.1 Singularities and the residue theorem

In Topic 4 we encountered branch points as an example of singularities of a function, where they fail to be analytic. Here we will investigate another type called isolated singularities of complex functions. In the region around such singularities, complex functions are described by Laurent expansions. We will see that integrals where the contour encloses an isolated singularity are determined by the coefficient associated with the first negative power in the Laurent series about that singularity: the residue. The extension to contours enclosing multiple isolated singularities yields the residue theorem for calculating complex integrals.

This topic covers: singularities; zeros; residues; the residue theorem; computing residues.

- - - - - -

This is a lecture course on complex analysis I made for an undergraduate class at the University of Bristol. I thought it'd be good to put them online so anybody can benefit from them.

If you use any of this material then please acknowledge this YouTube channel.

The course material is separated into nine topics, each comprising a handful of videos. The topics are:
1. Revision of complex numbers
2. Real analysis
3. Cauchy-Riemann equations and analyticity
4. Multivaluedness and branches
5. Cauchy's theorem and Cauchy's integral formula
6. Series expansions of complex functions
7. Singularities and the residue theorem
8. Real integrals 1
9. Real integrals 2

Here are links to material to accompany the videos: