filmov
tv
Types of Isolated Singularities - Complex Analysis By a Physicist
Показать описание
In this video we cover isolated singularities, and the three types of isolated singularities. The three kinds of isolated singularities covered are poles, essential singularities, and removeable singularities.
Understanding isolated singularities relies heavily on Laurent series.
You can refresh you memory on Laurent series here:
Music: Mirror Mind By Bobby Richards
Understanding isolated singularities relies heavily on Laurent series.
You can refresh you memory on Laurent series here:
Music: Mirror Mind By Bobby Richards
Types of Isolated Singularities - Complex Analysis By a Physicist
Complex Analysis 16 | Isolated Singularities
Types of isolated singularities (+several examples)
Lecture 2- Singularity of an Analytic function | Types of singularities | Complex Analysis
Complex analysis: Singularities
Basic Complex Analysis - Unit 3 - Lecture 5 - Singular Point
[CA/Week 2] 6. Types of singularities
The three different types of singularities
Types of Isolated Singularities
#4 types of isolated singularities|removable singularity|pole singularity|essential singularity
Basic Complex Analysis - Unit 3 - Lecture 7 - Removable Singular Point
Singularities of Analytic Functions -- Complex Analysis 20
Lec2/Singularities and Zeros/Classification of Isolated Singularities
Isolated Singularities and Residues
Basic Complex Analysis - Unit 3 - Lecture 8 - Essential Singular Point
Types of Isolated Singularity(Removable, Pole, Essential) Complex Analysis: lec-02
mod04lec35 - Isolated Singularities
Taxonomy of singularities of complex functions
Complex Analysis 28 - Types of Isolated Singularities, Cauchy Residue Theorem & Residue at infin...
Singularities and Types of Singularities | Isolated Singularities | Poles | Removable Singularities
Isolated singularities
Singularities and isolated singularities
Isolated singularities
Types of singularities || Isolated Singularity || Removable Singularity || Essential singularity
Комментарии
 0:05:25
0:05:25
 0:13:31
0:13:31
 0:18:11
0:18:11
 0:11:25
0:11:25
 0:27:37
0:27:37
 0:03:39
0:03:39
![[CA/Week 2] 6.](https://i.ytimg.com/vi/zMKqKdB7zRc/hqdefault.jpg) 0:08:04
0:08:04
 0:07:33
0:07:33
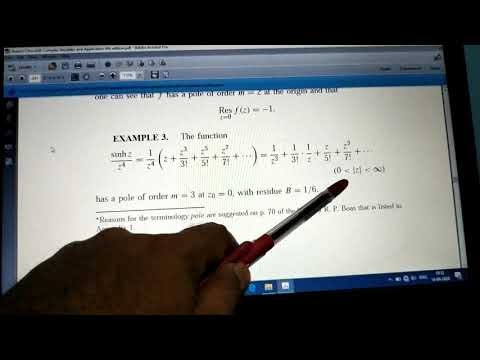 0:23:52
0:23:52
 0:27:55
0:27:55
 0:02:45
0:02:45
 0:42:07
0:42:07
 0:12:12
0:12:12
 0:18:44
0:18:44
 0:02:51
0:02:51
 0:31:41
0:31:41
 0:16:24
0:16:24
 0:17:19
0:17:19
 0:50:37
0:50:37
 0:39:31
0:39:31
 0:41:23
0:41:23
 0:06:49
0:06:49
 0:09:10
0:09:10
 0:16:30
0:16:30