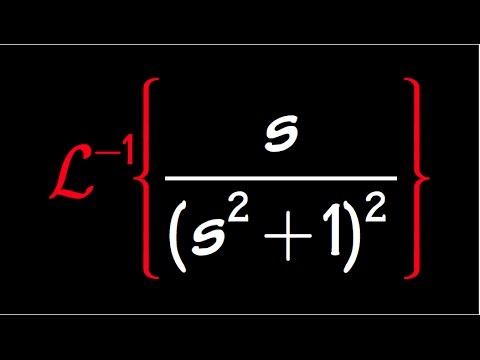
Using convolution theorem obtain the inverse Laplace transform simple example

Показать описание

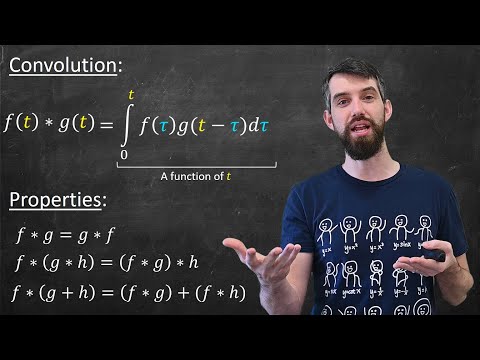
In this video explained Using convolution theorem obtain the inverse Laplace transform simple example. This is a very easy method. Solving differential equations: The convolution theorem allows us to solve differential equations in a more efficient way. By taking the Laplace transform of both sides of the differential equation we can convert it into an algebraic equation that involves the Laplace transforms of the functions involved. Then using the convolution theorem we can easily find the inverse Laplace transform of the product of the Laplace transforms which gives us the solution to the differential equation.

Another Educational Channel:-

LAPLACE TRANSFORM : 18MAT31

Fourier Transforms,Z-transform : 18MAT31 & 17MAT31

Fourier Series: 18MAT31 & 17MAT31

Calculus of Variation & Numerical Methods 18MAT31

Numerical Methods ODE's: 18MAT31 & 17MAT41

COMPLEX NUMBER: 18MATDIP31

Differential Calculus:18MATDIP31

Ordinary differential equation 18MATDIP31 & 17MATDIP31

Integral Calculus 18MATDIP31 & 17MATDIP31

Vector differentiation 18MATDIP31 & 17MATDIP31

Differential Calculus & Partial Differential 18MATDIP31 & 17MATDIP31

Joint Probability & Sampling Theory: 18MAT41 & 17MAT41

Probability Distributions: 18MAT41 & 17MAT41

Calculus of Complex Functions: 18MAT41 & 17MAT41

Curve fitting & Statistical Method 18MAT41 17MAT31

18MATDIP41 Linear Algebra

18MATDIP41 Numerical Methods

18MATDIP41 Higher order ODEs

18MATDIP41 Partial Differential Equations

Рекомендации по теме

Комментарии

Thank you sir... easily understandable 👍😊

shivaniudagatti

What is the Laplace transform of
sinht / t?

Dkhebbal

Using convolution theorem obtain the inverse Laplace transform simple example

inverse laplace of s/(s^2+1)^2, using convolution theorem

Using convolution theorem obtain the inverse Laplace transform simple example

The Convolution of Two Functions | Definition & Properties

The convolution and the laplace transform | Laplace transform | Khan Academy

Inverse Laplace Transform Using Convolution Theorem-Laplace Transform

Convolution theorem finding inverse Laplace transform example(PART-1) BY easy maths easy tricks

How to use the Convolution Theorem to Find the Laplace Transform of a Convolution EX e^(2t)*sin(t)

Inverse Laplace Transform Using Convolution Theorem-Laplace Transform

(6.3.102) Determine an Inverse Laplace Transform Using Convolution

31. Convolution Theorem | Complete Concept and Problem#1 | Inverse Laplace Transform

Using the convolution theorem to solve an initial value prob | Laplace transform | Khan Academy

❖ Convolution of Two Functions ❖

How to Find the Laplace Transform of a Definite Integral using the Convolution Theorem

Convolution Method to Find Inverse Laplace Transforms | Practice Problems

Convolution of Functions and Laplace Transforms Examples

Convolution Theorem | Problem 1 | Laplace Transform | Engineering Mathematics

Convolution Theorem #2 (V.Imp.) | Laplace Transform | Very Important Numerical Problems

Lesson 69: Using Convolution to Solve Inverse Laplace Transform (Engineering Math)

Convolution theorem finding inverse Laplace transform example(PART-3) by easy maths easy tricks

Convolution theorem finding inverse Laplace transform example(PART-2) by easy maths easy tricks

20. Convolution Theorem | Problem#2 | Inverse Laplace Transforms

Convolution theorem finding inverse Laplace transform example(PART-4) by easy maths easy tricks

Question#2 on Convolution Theorem for Finding Inverse Laplace Transform.

Inverse Laplace Transform Using Convolution Theorem in Tamil-Laplace Transform