filmov
tv
Advanced Functions 5.3 Graphing Rational Functions with Oblique Asymptotes

Показать описание
We also take a look at holes in the function.
Advanced Functions 5.3 Graphing Rational Functions
Day 5 HW #3 Graphing Rational Functions
Advanced Functions 5.3 Graphing Rational Functions with Oblique Asymptotes
Vertical and Horizontal Asymptotes Grade 12 Advanced Functions Lesson 5 3 11 7 12
MHF4U - Unit 5 - Lesson 3 - 5.3 Graphs of Rational Functions
Graphing Advanced Rational Functions With Asymptotes and Holes Using Transformations
Rational Graphs Made Easy Find the vertical and horizontal asymptote
3 5 Rational Functions Graphs Applications and Models Class
Graphing Rational Functions with Two Vertical Asymptotes
How to graph a rational function using 6 steps
5.3 Graphs of Rational Functions (Grade 12 University MHF4U)
Solving Rational Functions Grade 12 Advanced Functions Lesson 5 4 11 11 12
Advanced Functions MHF4U Lesson Chap 5.030 Graphing Rational Functions ('Linear over Linear&apo...
REVIEW for Unit 5 Test 3: Graphs of Rational Functions
Day 5 Quiz A #1 and #2 Graphing Rational Functions
Advanced Functions MHF4U Lesson Chap 5.050 Graphing curves with an oblique asymptote
Functions 2.5 Rational Functions : There's a HOLE in the graph!
The Hardest Math Test
Identifying vertical, horizontal asymptotes and holes
Math 3 - Graphing Rational Function without Hole
Advanced Functions MHF4U Lesson Chap 5.040 Graphing Rational Functions with Quadratic Denominators
Day 5 Quiz A #3 and #4 Graphing Rational Functions
Intervals of Increase and Decrease Grade 12 Advanced Functions Lesson 5 3 11 16 15
Solving Business Problems Using Rational Functions Grade 12 Advanced Functions Lesson 5 4 11 11 12
Комментарии
 0:29:00
0:29:00
 0:19:04
0:19:04
 0:26:22
0:26:22
 0:04:34
0:04:34
 0:36:46
0:36:46
 0:30:16
0:30:16
 0:10:50
0:10:50
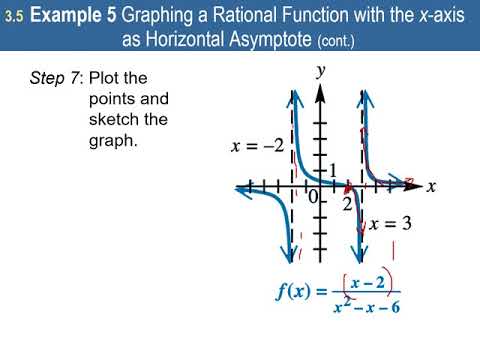 0:20:38
0:20:38
 0:14:28
0:14:28
 0:11:05
0:11:05
 0:10:40
0:10:40
 0:06:09
0:06:09
 0:30:29
0:30:29
 0:29:56
0:29:56
 0:25:20
0:25:20
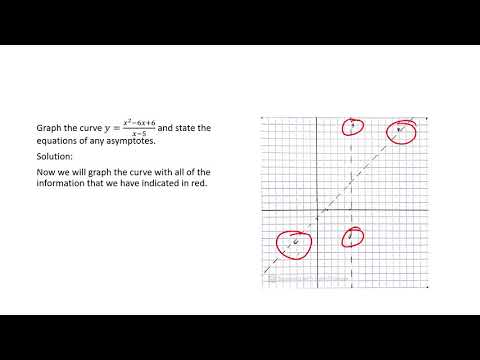 0:18:03
0:18:03
 0:10:01
0:10:01
 0:00:28
0:00:28
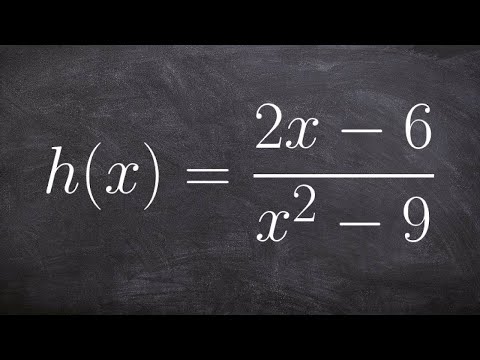 0:02:16
0:02:16
 0:06:33
0:06:33
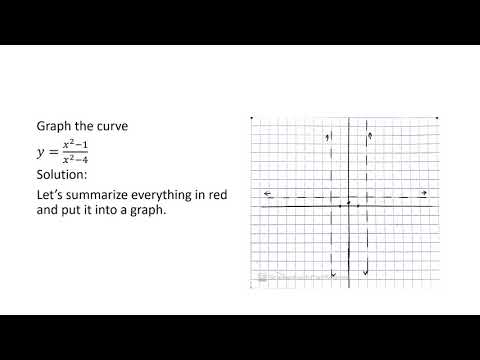 0:10:56
0:10:56
 0:20:31
0:20:31
 0:16:24
0:16:24
 0:15:15
0:15:15