filmov
tv
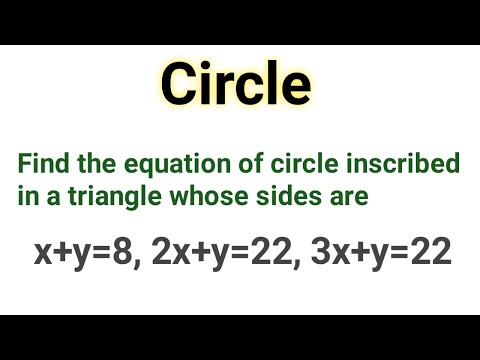
Find Equation of Circle Inscribed in a Triangle Formed by Intersection of Three Lines
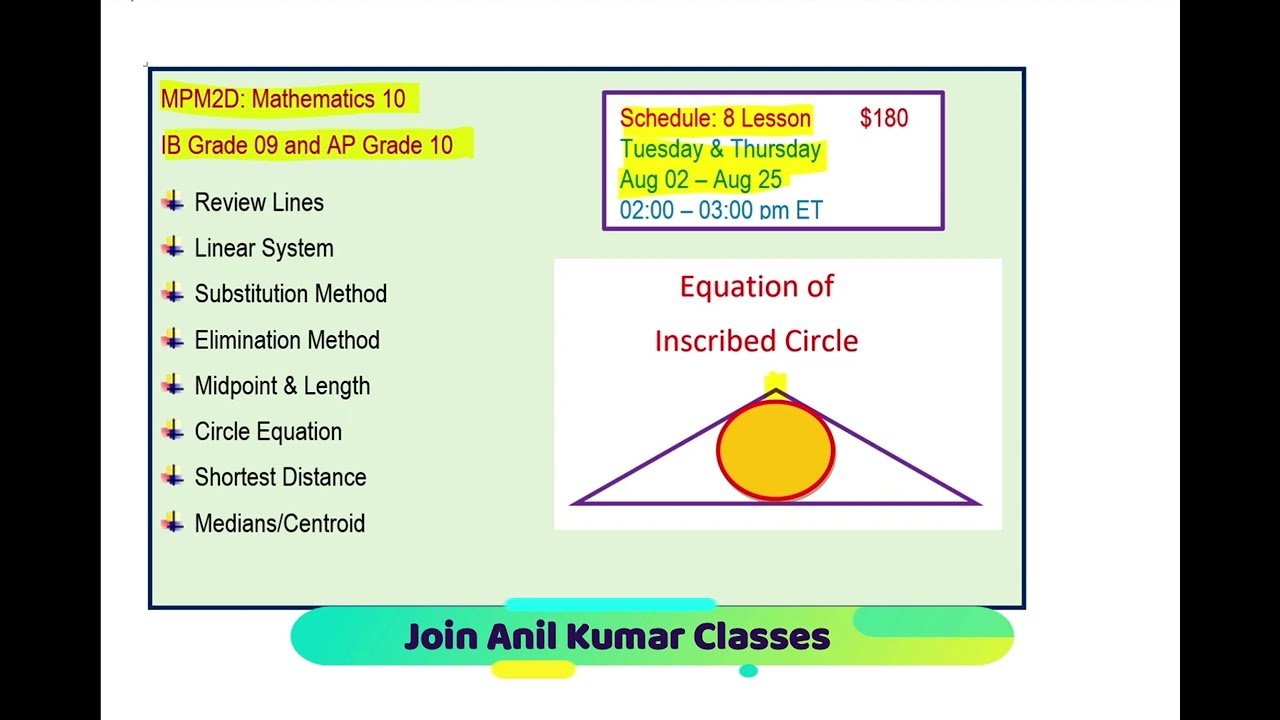
Показать описание
Circumcenter of a triangle is the point of intersection of the right bisector of the three sides of a triangle. All the three vertices are same distance away from the circumcentre and so a circle can be drawn circumscribing the triangle using this point as the centre. Orthocentre of a triangle is a point where the perpendiculars drawn from each vertex to the opposite sides intersect. For an acute angle triangle, the orthocenter is inside the triangle; for right triangle it is on the hypotenuse and for the obtuse angle triangle it lies outside the triangle. Incentre of a triangle is the point of intersection of three interior angles of a triangle. A circle inscribed in a triangle can be drawn with center at the incenter.
#mpm2d_geometry #sat_act_tutor #mpm2d_areaoftriangle #analyticgeometry #coordinategeometryclass10
#mpm2d_geometry #sat_act_tutor #mpm2d_areaoftriangle #analyticgeometry #coordinategeometryclass10
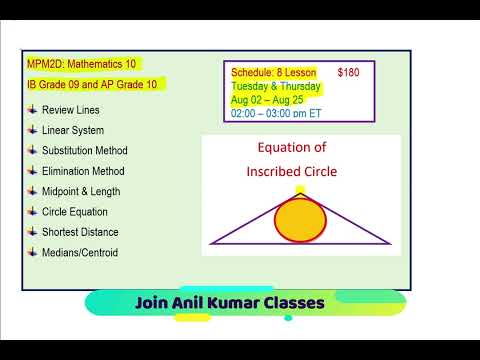 0:16:48
0:16:48
 0:04:18
0:04:18
 0:03:09
0:03:09
 0:11:23
0:11:23
 0:07:37
0:07:37
 0:10:10
0:10:10
 0:04:25
0:04:25
 0:10:04
0:10:04
 0:38:17
0:38:17
 0:04:06
0:04:06
 0:10:25
0:10:25
 0:04:31
0:04:31
 0:32:31
0:32:31
 0:05:06
0:05:06
 0:12:55
0:12:55
 0:15:31
0:15:31
 0:00:42
0:00:42
 0:05:40
0:05:40
 0:03:27
0:03:27
 0:01:01
0:01:01
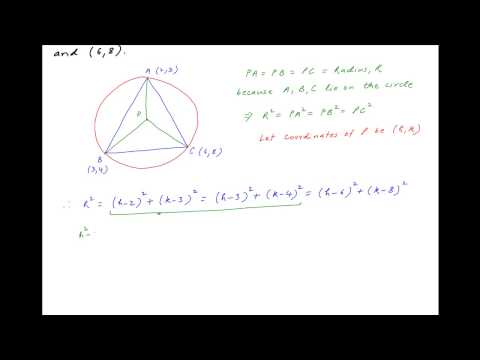 0:04:43
0:04:43
 0:00:33
0:00:33
 0:07:40
0:07:40
 0:09:35
0:09:35