Gauss~Jordan Elimination - Reduced Row-Echelon Form #1

How to Use Gauss-Jordan Elimination to Solve Systems of Linear Equations

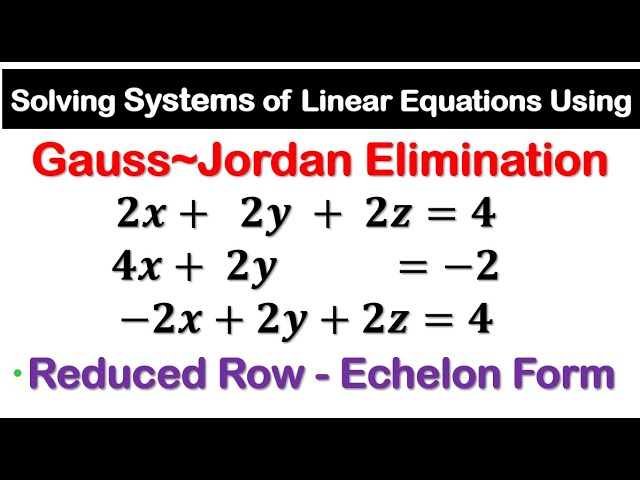
In this video, I’ll walk you through the step-by-step process of using Gauss-Jordan elimination to solve systems of linear equations. This powerful method simplifies complex systems into a form where solutions become clear and easy to interpret. Whether you're tackling two-variable equations or more advanced systems, Gauss-Jordan elimination is an essential technique in linear algebra.

📌 What You’ll Learn:
- The fundamentals of the Gauss-Jordan elimination method
- How to transform a system into its row echelon form
- How to identify and solve for variables using back substitution

🔥 Don’t forget to like, share, and subscribe for more math tutorials!

🔔Subscribe, and hit the notification bell for more math tips and tricks

Timestamps for Easy Navigation:
0:00 Introduction
0:33 Augmented Matrix
1:15 Reduced Row-Echelon form
2:20 Row Operations
18:48 Back-substitution
19:50 Final solution

You can also check this out:

~ Playlist on GPA and CGPA Calculations

~Playlist on Linear Algebra 1

~Playlist on Complex Numbers

~Playlist on BakoMaths Shorts

#MathTutorial #GaussJordanElimination #LinearEquations #Algebra #MathMadeSimple