filmov
tv
Math of Musical Scales, Part 2 of 3
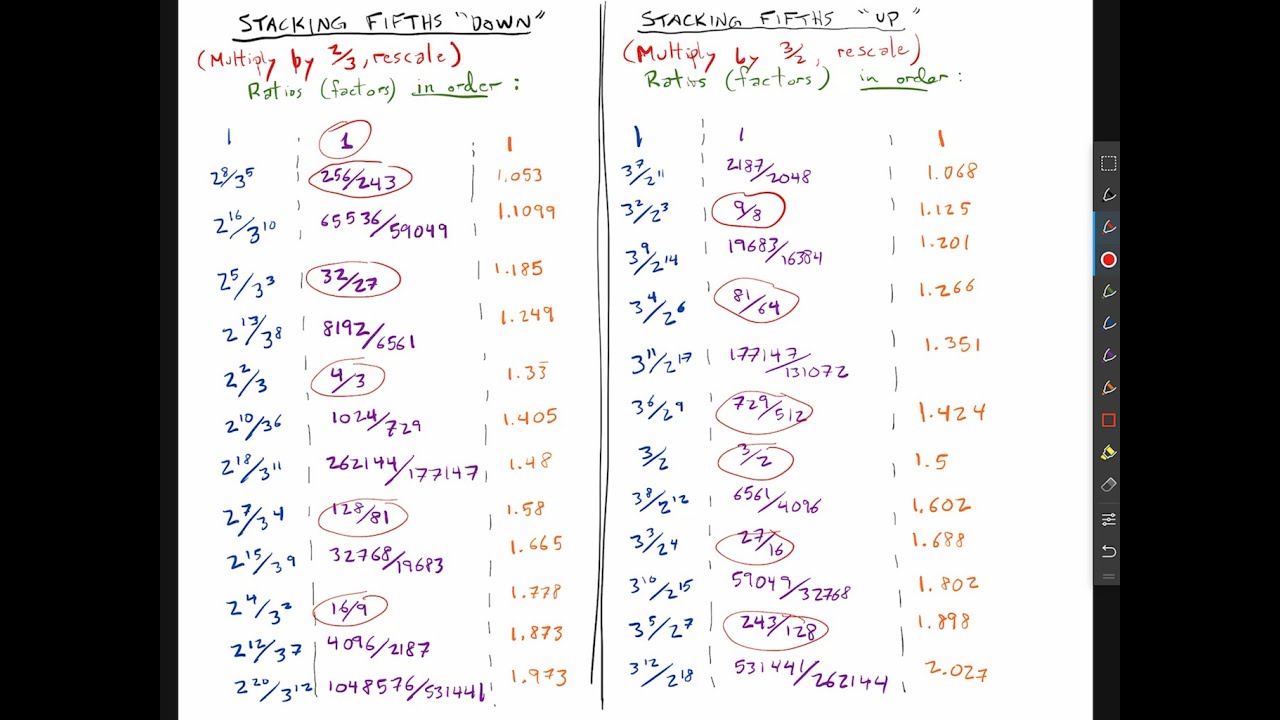
Показать описание
Math of Musical Scales, Part 1 of 3
Math of Musical Scales, Part 2 of 3
Math of Musical Scales, Part 3 of 3
The maths behind musical scales
The Math Behind Music and Sound Synthesis
How Pythagoras Broke Music (and how we kind of fixed it)
The Geometry of Music
The Simple Math of Music Theory
Encoding the Fibonacci Sequence Into Music
The mathematics of Scales and Modes | Maths and Music | N J Wildberger
MUSIC THEORY in 12 minutes for nOOBS
Music and Math, Mathematical Concepts in Music, Scales Harmony and Ratios, Math and Music
Music and Scales - Brain Waves.avi
The Mathematical Beauty of Musical Scales
Why Does Music Only Use 12 Different Notes?
Modes of Minor and other Scales | Maths and Music | N J Wildberger
Music Theory 2 Scales and Math
Modular Arithmetic and Scales
What nobody told you about Scales - Part 1/3 | [MUSIC THEORY HACK]
Whistle Math Part 1: Scales, Modes, and Keys
The Math Behind Music
Music Scales Explained in 6 Minutes
Pythagorean Scales - Maths with Music
Understanding Music scales - music theory 101
Комментарии
 0:13:52
0:13:52
 0:11:46
0:11:46
 0:12:06
0:12:06
 0:05:29
0:05:29
 0:13:26
0:13:26
 0:17:40
0:17:40
 0:02:42
0:02:42
 0:13:02
0:13:02
 0:02:40
0:02:40
 0:28:47
0:28:47
 0:11:44
0:11:44
 0:02:19
0:02:19
 0:10:21
0:10:21
 0:00:41
0:00:41
 0:17:41
0:17:41
 0:18:55
0:18:55
 0:12:18
0:12:18
 0:06:15
0:06:15
 0:03:59
0:03:59
 0:20:17
0:20:17
 0:04:01
0:04:01
 0:06:19
0:06:19
 0:01:42
0:01:42
 0:03:56
0:03:56