filmov
tv
Lagrange's Theorem and Index of Subgroups | Abstract Algebra
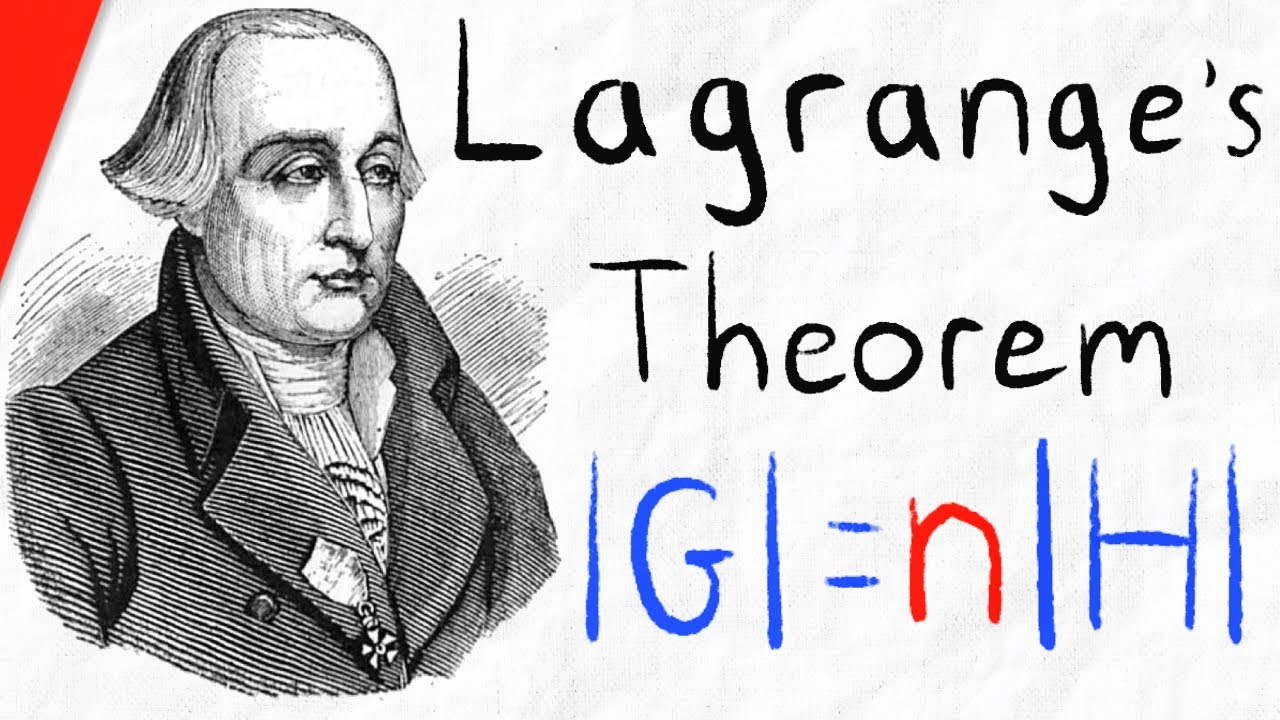
Показать описание
We introduce Lagrange's theorem, showing why it is true and follows from previously proven results about cosets. We also investigate groups of prime order, seeing how Lagrange's theorem informs us about every group of prime order - in particular it tells us that any group of prime order p is cyclic and has all of its non-identity elements as generators. Thus, there is only one group (up to isomorphism) of any given prime order. We conclude with a brief discussion of the index of a subgroup H in a group G, showing that the index of a subgroup is equal to the order of the group divided by the order of the subgroup. #abstractalgebra #grouptheory
Table of Contents
0:00 Intro
1:32 Proving Lagrange's Theorem
5:06 Examples
6:42 Groups of Prime Order are Cyclic
10:07 Index of a Subgroup
11:31 Recap
Abstract Algebra Exercises:
◉Textbooks I Like◉
★DONATE★
Thanks to Petar, dric, Rolf Waefler, Robert Rennie, Barbara Sharrock, Joshua Gray, Karl Kristiansen, Katy, Mohamad Nossier, and Shadow Master for their generous support on Patreon!
Follow Wrath of Math on...
Table of Contents
0:00 Intro
1:32 Proving Lagrange's Theorem
5:06 Examples
6:42 Groups of Prime Order are Cyclic
10:07 Index of a Subgroup
11:31 Recap
Abstract Algebra Exercises:
◉Textbooks I Like◉
★DONATE★
Thanks to Petar, dric, Rolf Waefler, Robert Rennie, Barbara Sharrock, Joshua Gray, Karl Kristiansen, Katy, Mohamad Nossier, and Shadow Master for their generous support on Patreon!
Follow Wrath of Math on...
Комментарии
 0:12:15
0:12:15
 0:09:19
0:09:19
 0:15:12
0:15:12
 0:07:22
0:07:22
 0:13:02
0:13:02
 0:06:53
0:06:53
 0:10:12
0:10:12
 0:08:29
0:08:29
 0:50:41
0:50:41
 0:06:12
0:06:12
 0:13:39
0:13:39
 0:02:52
0:02:52
 0:06:29
0:06:29
 0:13:51
0:13:51
 0:12:14
0:12:14
 0:15:26
0:15:26
 0:16:34
0:16:34
 0:02:13
0:02:13
 0:13:50
0:13:50
 0:03:03
0:03:03
 0:00:16
0:00:16
 0:00:12
0:00:12
 0:14:05
0:14:05
 0:16:04
0:16:04