filmov
tv
Calculus 1 - Derivatives and Related Rates (16 of 24) Filling a Semi-Sphereical Bowl V(H2O)=?
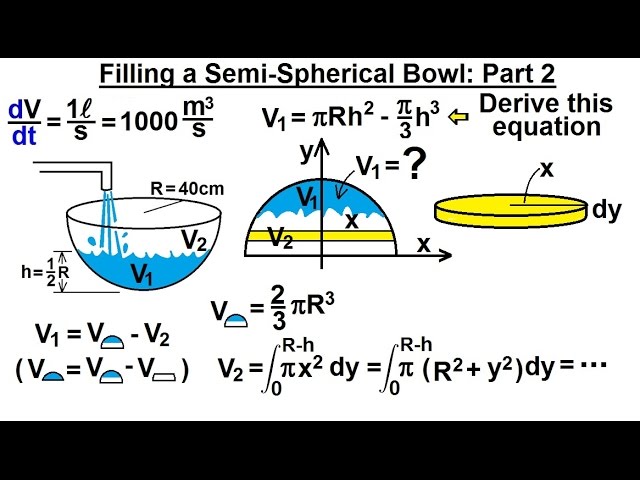
Показать описание
I this video I will derive the volume equation of the water level of a semi-spherical container.
Next video in this series can be seen at:
Calculus 1 - Derivatives
Understand Calculus in 35 Minutes
Derivative as a concept | Derivatives introduction | AP Calculus AB | Khan Academy
CALCULUS 1: DERIVATIVES
Definition of the Derivative
Derivatives for Beginners - Basic Introduction
Intro to Derivatives, Limits & Tangent Lines in Calculus | Step-by-Step
Calculus 1 Final Exam Review
Chain Rule Method of Differentiation | Derivatives #excellenceacademy #jonahemmanuel
100 calculus derivatives (ultimate derivative tutorial)
Chain Rule For Finding Derivatives
Calculus 1: Derivatives and Rates of Change (Video #7) | Math with Professor V
The essence of calculus
Review of all Derivative Rules | Calculus | jensenmath
BASIC DERIVATIVE OF ALGEBRAIC FUNCTIONS || BASIC CALCULUS
The paradox of the derivative | Chapter 2, Essence of calculus
Derivative formulas through geometry | Chapter 3, Essence of calculus
Calculus 1 - Full College Course
ALL OF Calculus 1 in a nutshell.
Calculus 1: The Derivative as a Function (Video #8) | Math with Professor V
Finding the Derivative of a Polynomial Function | Intro to Calculus #shorts #math #maths
Derivative of ln(1/x), calculus 1 tutorial
Calculus 1 Lecture 2.6: Discussion of the Chain Rule for Derivatives of Functions
Calculus 1: Limits & Derivatives (1 of 27) The Tangent Line and The Secant Line - Reviewed
Комментарии
 0:52:51
0:52:51
 0:36:22
0:36:22
 0:07:16
0:07:16
 0:20:10
0:20:10
 0:23:31
0:23:31
 0:58:04
0:58:04
 0:28:33
0:28:33
 0:55:58
0:55:58
 0:01:00
0:01:00
 6:38:13
6:38:13
 0:18:40
0:18:40
 0:33:32
0:33:32
 0:17:05
0:17:05
 0:21:26
0:21:26
 0:20:11
0:20:11
 0:16:50
0:16:50
 0:17:34
0:17:34
 11:53:48
11:53:48
 0:05:24
0:05:24
 0:27:40
0:27:40
 0:01:01
0:01:01
 0:00:52
0:00:52
 1:34:01
1:34:01
 0:02:06
0:02:06