filmov
tv
Calculus 1 Lecture 2.6: Discussion of the Chain Rule for Derivatives of Functions

Показать описание
Calculus 1 Lecture 2.6: Discussion of the Chain Rule for Derivatives of Functions
Calculus 1 Lecture 2.6: Discussion of the Chain Rule for Derivatives of Functions
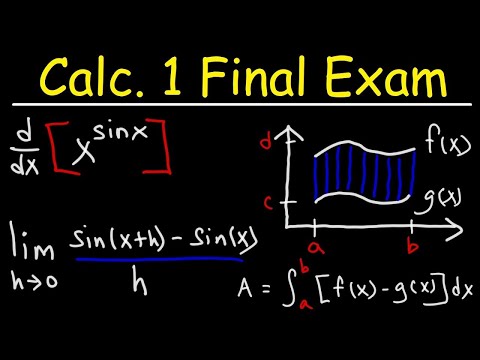
Calculus 1 Final Exam Review
Understand Calculus in 35 Minutes
How to Make it Through Calculus (Neil deGrasse Tyson)
ME564 Lecture 2: Review of calculus and first order linear ODEs
Calculus 2 Lecture 6.6: A Discussion of Hyperbolic Functions
AP Calculus AB Unit 6 Review | Riemann Sums, Integration, FTC Part I & II, U-Substitution
Calculus 1 - Derivatives
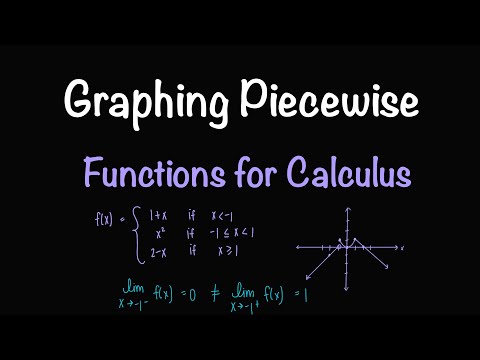
Graphing Piecewise Functions for Calculus & Finding Limits MADE EASY! | Math with Professor V
Calculus 1 and 2 Review
06 - What is a Function in Math? (Learn Function Definition, Domain & Range in Algebra)
Calculus 1 - Full College Course
A Year's Worth of Calculus in 1 Minute
ALL of calculus 3 in 8 minutes.
why calculus students struggle
The essence of calculus
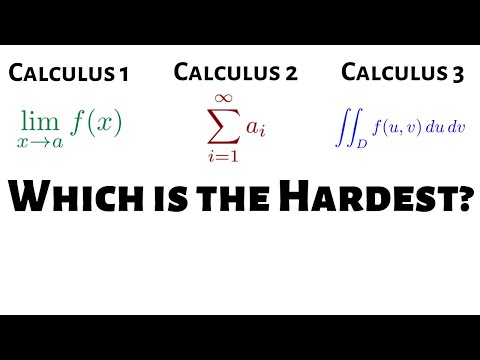
What is the Hardest Calculus Course?
when calculus students use trig identities too early
Why People FAIL Calculus (Fix These 3 Things to Pass)
Fundamental Theorem of Calculus Part 1
Calculus 1 Lecture 0.1: Lines, Angle of Inclination, and the Distance Formula
Calculus 1 - Introduction to Limits
What is a function? | Functions and their graphs | Algebra II | Khan Academy
Calculus 1 Exam 2 Review Problems and Solutions (Derivatives and Their Applications)
Комментарии
 1:34:01
1:34:01
 0:55:58
0:55:58
 0:36:22
0:36:22
 0:03:38
0:03:38
 0:48:43
0:48:43
 1:16:06
1:16:06
 0:07:43
0:07:43
 0:52:51
0:52:51
 0:34:37
0:34:37
 0:24:00
0:24:00
 0:26:18
0:26:18
 11:53:48
11:53:48
 0:01:09
0:01:09
 0:08:10
0:08:10
 0:00:09
0:00:09
 0:17:05
0:17:05
 0:01:44
0:01:44
 0:00:43
0:00:43
 0:03:15
0:03:15
 0:11:30
0:11:30
 0:48:58
0:48:58
 0:20:20
0:20:20
 0:07:57
0:07:57
 1:09:27
1:09:27