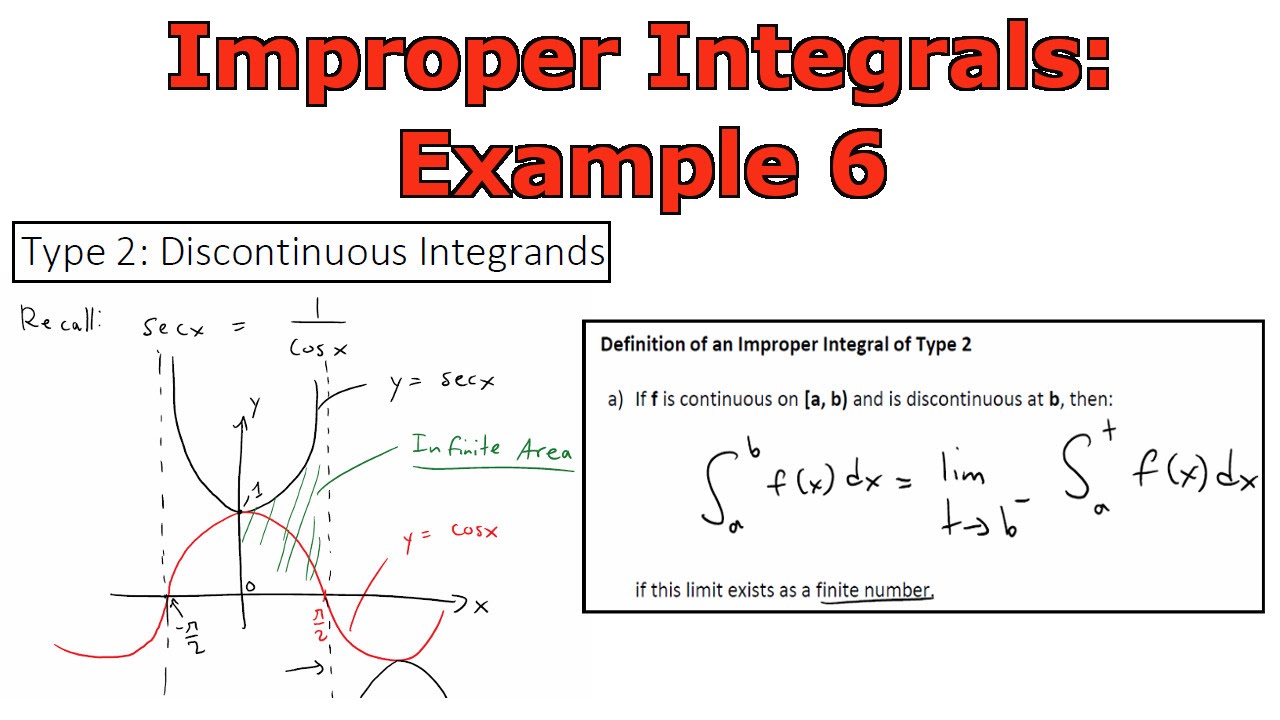
Improper Integrals: Example 6: sec(x)

In this video I go over another example on improper integrals and this time solve the integral of the function sec(x) from x = 0 to x = π/2. This function has a vertical asymptote at x = π/2 because sec(x) approaches infinite as x approaches π/2, thus making it a Type 2 improper integral. Solving this by first writing it as a limit of a definite integral yields a divergent integral because the limit approaches infinite. In this particular integral, the divergent limit represents an infinite area under the curve.

Related Videos:

------------------------------------------------------

[mes]

I don't always integrate trig functions but when I do I usually deal with divergent improper integrals ;)