filmov
tv
Haar measure 07 Existence Lemma 2 part 2 a lemma on uniform continuity

Показать описание
In mathematics, there are a couple of theorems whose statements we are super-familiar with, but whose (usually long) proofs we wish we never have to read in details. The theorem on the existence and uniqueness of Haar measures on locally compact groups was one of such on my list. Recently however, I found the beauty in its proof: the art of creating an integration theory out of very limited information: locally compact group. In this proof, you will be able to see an ingenious use of Tychonoff's theorem, a canonical application of Riesz Representation theorem, a magical manipulation of Fubini-Tonelli theorem, among many other tricks... I couldn't wait to figure out all details and now, present it to you! In this video series, we will start with Haar's original idea of set-theoretical proof (1933) and then move on to Weil's functional proof (1940). I will try to provide motivations as well as adequate details.
Haar measure 07 Existence Lemma 2 part 2 a lemma on uniform continuity
Haar measure 06 Existence Lemma 2 part 1 approximates are approximately additive
Haar measure 08 Existence Lemma 2 part 3 finishing the proof and a corrollary
Haar measure 04 Existence Lemma 1 properties of the generalized Haar covering number
Haar measure 7 - right invariance and modular function
Haar measure 02 Existence Haar's original idea
Haar measure 6 - proof of left invariance
Haar measure 05 Existence Weil's idea of generalizing Haar's approximates
Haar measure 11 Existence The candidate extends to a left invariant p l f part 1
Haar measure 09 Existence Tychonoff's theorem and the candidate for positive linear functional
Haar measure 01 introduction
Haar measure 03 Existence Weil's generalization of Haar covering number
Haar measure 10 Existence The approximation to the candidate
Haar measure 12 Existence The candidate extends to a left invariant p l f part 2
Lecture 26: Haar measures
Haar measure - Lecture 2
Haar measure 16 Uniqueness The general case
Lecture 25, Haar measures, a teaser.
Haar measure 13 Existence Application of Riesz Representation theorem The left invariance
Anti-Concentration for the Unitary Haar Measure and Applications to Random Quantum Circu
Properties of Radon measures and Lusin's theorem on LCH spaces
The lemma of Jordan
Learning Beyond Stabilizer States
Zoom Lecture 4: Foundations of Quantitative Risk Measurement
Комментарии
 0:24:23
0:24:23
 0:12:49
0:12:49
 0:29:12
0:29:12
 0:14:17
0:14:17
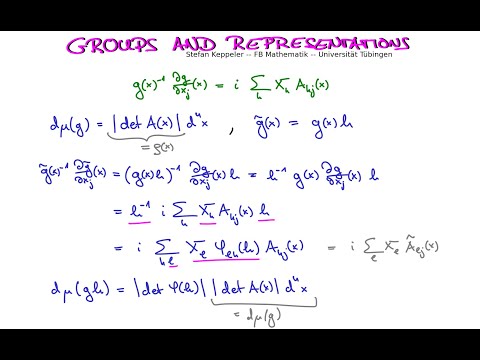 0:05:56
0:05:56
 0:08:29
0:08:29
 0:05:21
0:05:21
 0:06:16
0:06:16
 0:21:16
0:21:16
 0:21:47
0:21:47
 0:08:27
0:08:27
 0:26:24
0:26:24
 0:12:23
0:12:23
 0:11:21
0:11:21
 1:08:00
1:08:00
 0:21:41
0:21:41
 0:26:00
0:26:00
 0:52:36
0:52:36
 0:19:33
0:19:33
 0:43:13
0:43:13
 0:17:51
0:17:51
 0:09:32
0:09:32
 1:03:45
1:03:45
 1:46:28
1:46:28