filmov
tv
Haar measure 12 Existence The candidate extends to a left invariant p l f part 2

Показать описание
In mathematics, there are a couple of theorems whose statements we are super-familiar with, but whose (usually long) proofs we wish we never have to read in details. The theorem on the existence and uniqueness of Haar measures on locally compact groups was one of such on my list. Recently however, I found the beauty in its proof: the art of creating an integration theory out of very limited information: locally compact group. In this proof, you will be able to see an ingenious use of Tychonoff's theorem, a canonical application of Riesz Representation theorem, a magical manipulation of Fubini-Tonelli theorem, among many other tricks... I couldn't wait to figure out all details and now, present it to you! In this video series, we will start with Haar's original idea of set-theoretical proof (1933) and then move on to Weil's functional proof (1940). I will try to provide motivations as well as adequate details.
 0:11:21
0:11:21
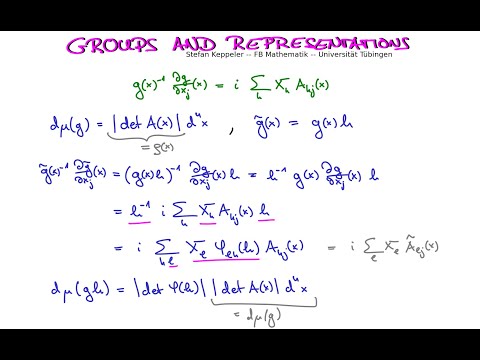 0:05:56
0:05:56
 0:12:23
0:12:23
 0:19:33
0:19:33
 0:59:48
0:59:48
 0:29:12
0:29:12
 0:12:49
0:12:49
 0:57:27
0:57:27
 0:21:41
0:21:41
 0:12:15
0:12:15
 0:52:36
0:52:36
 0:26:00
0:26:00
 0:43:13
0:43:13
 0:10:38
0:10:38
 0:00:31
0:00:31
 0:00:13
0:00:13
 0:47:00
0:47:00
 0:00:42
0:00:42
 1:01:12
1:01:12
 1:22:20
1:22:20
 0:46:15
0:46:15
 0:09:20
0:09:20
 0:12:29
0:12:29
 0:15:52
0:15:52