filmov
tv
Linear Relations and Applications Test MPM1D IB Grade 9
Показать описание
#linearequations_wordproblems #linearequations_applications #globalmathinstitute #anilkumar
Q1. True or False
a. A line with undefined slope is horizontal.
b. A line with negative slope always tilts up to the left.
c. The y-intercept of a line is the point with x-coordinate equal to zero.
d. For the line, the run is considered the horizontal change.
e. The graph of x = 4 is a line parallel to y – axis.
f. The point (-3, 5) is located below the x – axis.
g. A line with a slope of 2 is perpendicular to a line with slope ½.
h. The line 3x + y = 6 has the slope of -3.
i. The point (-1, -3) lies on the line y = 2x + 5.
j. The line y = 4 has the slope equal to zero.
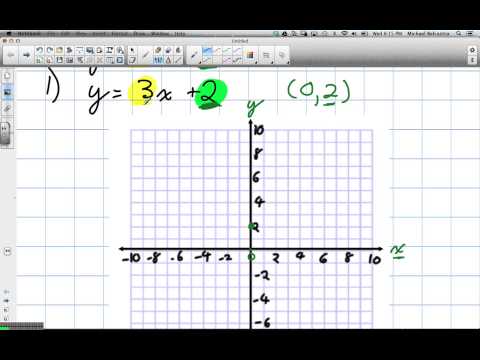
Q2. Determine equation of each line shown in the graph and find the point of intersection.
Q3. Find equation of line with x-intercept of 4 and y-intercept of -3.
Q4. Determine the equation of a line passing through the points A(2, 5) and B(4, 11).
Q5. Find the intercepts of the line 5x – 3y + 15 = 0.
Q6. The equation of the line is 2x – 5y = k. Find k if line passes through the point P(-7, -2).
Q7. Find equation of the line which passes through (-3, 6) and is parallel to 3x – 4y = 12.
Q8. Use intercepts method to sketch the line 27x – 36y = 108.
Applications
Q1. The annual cost of operating a motorcycle varies partially as the distance driven. The cost is $610 for 2000 km, and $850 for 5000 km.
a. Determine the relation that represents this relation.
b. Find the cost of driving 9000 km.
c. If the annual cost is $550, how many km can be driven.
Q2. A health club charges its members a fixed annual fee, plus a fee each time they visit the club. In the same year, Richard visited the club 50 times and paid total of $450, Nigel visited the club 120 times and paid a total of $800. What are the fixed fee and fee per visit?
Q3. A school team travels from Malton to Ottawa, a distance of approximately 520 km. The first part of the trip is completed by car, which averaged 80 km/h. The last part of the trip is by train which travels 120 km/h. If entire trip takes 5 hours, determine the distance the school team spent travelling by train. #GCSE #SAT #EQAO #IBSLmath
Q1. True or False
a. A line with undefined slope is horizontal.
b. A line with negative slope always tilts up to the left.
c. The y-intercept of a line is the point with x-coordinate equal to zero.
d. For the line, the run is considered the horizontal change.
e. The graph of x = 4 is a line parallel to y – axis.
f. The point (-3, 5) is located below the x – axis.
g. A line with a slope of 2 is perpendicular to a line with slope ½.
h. The line 3x + y = 6 has the slope of -3.
i. The point (-1, -3) lies on the line y = 2x + 5.
j. The line y = 4 has the slope equal to zero.
Q2. Determine equation of each line shown in the graph and find the point of intersection.
Q3. Find equation of line with x-intercept of 4 and y-intercept of -3.
Q4. Determine the equation of a line passing through the points A(2, 5) and B(4, 11).
Q5. Find the intercepts of the line 5x – 3y + 15 = 0.
Q6. The equation of the line is 2x – 5y = k. Find k if line passes through the point P(-7, -2).
Q7. Find equation of the line which passes through (-3, 6) and is parallel to 3x – 4y = 12.
Q8. Use intercepts method to sketch the line 27x – 36y = 108.
Applications
Q1. The annual cost of operating a motorcycle varies partially as the distance driven. The cost is $610 for 2000 km, and $850 for 5000 km.
a. Determine the relation that represents this relation.
b. Find the cost of driving 9000 km.
c. If the annual cost is $550, how many km can be driven.
Q2. A health club charges its members a fixed annual fee, plus a fee each time they visit the club. In the same year, Richard visited the club 50 times and paid total of $450, Nigel visited the club 120 times and paid a total of $800. What are the fixed fee and fee per visit?
Q3. A school team travels from Malton to Ottawa, a distance of approximately 520 km. The first part of the trip is completed by car, which averaged 80 km/h. The last part of the trip is by train which travels 120 km/h. If entire trip takes 5 hours, determine the distance the school team spent travelling by train. #GCSE #SAT #EQAO #IBSLmath
0:36:28
Linear Relations and Applications Test MPM1D IB Grade 9
0:32:05
Linear Equations - Algebra
0:45:44
Linear Relations Complete Solved Test Paper y = mx + b MPM1D Grade 9 Math
0:21:22
Review Lines and Linear Relations with Application Important for EQAO TEST Preparation
0:39:43
Linear Relations Review Test MPM1D
0:08:56
How to Graph Linear Relations Grade 9 Academic
0:01:36
Linear Relationship Between Variables : Algebra
0:15:01
Linear Functions
1:01:01
MACHINE LEARNING SPECIALTY (MLS-C01) Exam Practice Questions - ANALYSIS P1
0:01:57
How to Distinguish Between Linear & Nonlinear : Math Teacher Tips
0:13:10
Graphs of linear equations | Linear equations and functions | 8th grade | Khan Academy
0:11:20
Linear Equation | Solving Linear Equations
0:16:28
Linear Relations First Difference Find Unknown Values from Table Write Equation of Line and Points
0:06:05
Depleting Bank Account Linear Relation Test Linear Application
0:04:34
How do we solve a system of linear equations using any method
0:34:20
Full Linear Relations Practice Test | y = mx+b | Rule of Four #howto #crashcourse #amazing #math #IQ
0:02:34
Linear Regression in 2 minutes
0:01:54
How to Solve Linear Equations With Variables on Both Sides : Linear Algebra Education
0:05:15
Solving Systems of Equations By Graphing
0:15:18
📐 Master SAT Math: Ace Linear Equation Questions Every Time! 🎯
0:06:24
How To Solve Linear Inequalities, Basic Introduction, Algebra
0:09:26
Solving Linear Equations in One Variable - Grade 7 Math @MathTeacherGon
0:09:43
Learn Functions – Understand In 7 Minutes
0:05:57
Drawing Linear Graphs
Комментарии