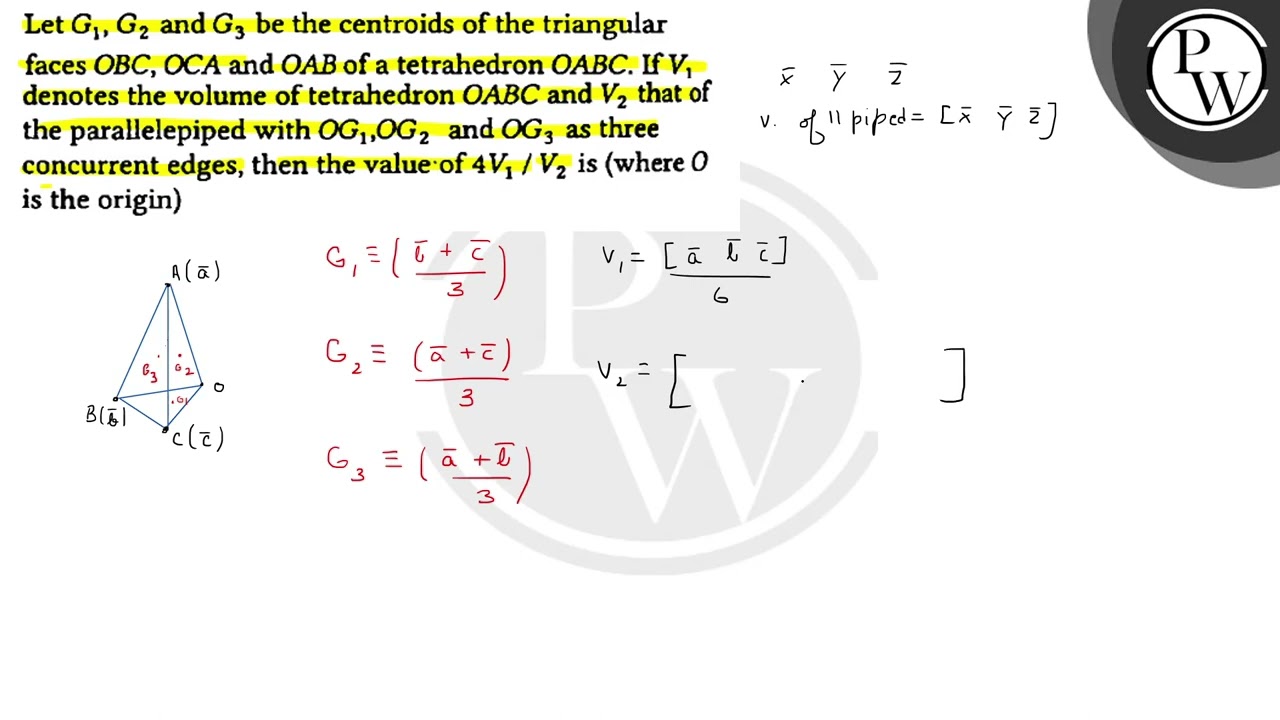
Let \( G_{1}, G_{2} \) and \( G_{3} \) be the centroids of the tria...

Let \( G_{1}, G_{2} \) and \( G_{3} \) be the centroids of the triangular faces \( O B C, O C A \) and \( O A B \) of a tetrahedron \( O A B C \). If \( V_{1} \) denotes the volume of tetrahedron \( O A B C \) and \( V_{2} \) that of the parallelepiped with \( O G_{1}, O G_{2} \) and \( O G_{3} \) as three concurrent edges, then the value of \( 4 V_{1} / V_{2} \) is (where 0 is the origin)