Let `G_(1), G(2) and G_(3)` be the centroid of the triangular faces OBC, OCA and OAB

preview_player
Показать описание
Let `G_(1), G(2) and G_(3)` be the centroid of the triangular faces OBC, OCA and OAB of a tetrahedron OABC. If `V_(1)` denotes the volume of tetrahedron OABC and `V_(2)` that of the parallelepiped with `OG_(1), OG_(2) and OG_(3)` as three concurrent edges, then the value of `(4V_(1))/(V_2)` is (where O is the origin
  1. Doubtnut
Рекомендации по теме
Let A1 and 0:12:41

Let A1 and A2 be two arithmetic means and G1, G2 and G3 be three geometric means of two distinct

Let g:R to 0:08:39

Let g:R to R be a non constant twice differentiable function such that g'(1/2)=g'(3/2).If ...

Let g(x)=x^2+bx+c. If 0:01:48

Let g(x)=x^2+bx+c. If g(2)=0 and g(-3)=0, find b+c

Find the derivative 0:01:23

Find the derivative of inverse of a function given values

Let `g(x)` be 0:02:00

Let `g(x)` be a function satisfying `g(0) = 2, g(1) = 3, g(x + 2) = 2g(x)-g(x + 1)`, then fi...

Learn how to 0:02:15

Learn how to evaluate for a function

Let g x 0:00:31

Let g x x f t dt where f is the function whose graph is shown a Evaluate g 0 g 1 g 2 g 3 and g 6 ...

Find inside function 0:02:03

Find inside function from the given Composite Function

If the mappings 0:03:09

If the mappings f and g are given by: f={(1,2),(3,5),(4,1)} and g={(2,3),(5,1),(1,3)},rite fog.

f(x)=3x-1; g(x)=x^2-x; Find 0:01:19

f(x)=3x-1; g(x)=x^2-x; Find (f/g)(x)

Let g : 0:03:47

Let g : R → R be a non constant twice differentiable such that g' (1/2)=g'(3/2). If a real...

JEE main 2016:Let 0:09:42

JEE main 2016:Let 𝑓:𝑅→𝑅,𝑔:𝑅→𝑅 and ℎ:𝑅→𝑅 be differentiable functions such that 𝑓(𝑥)=𝑥^3+3𝑥+2,𝑔(𝑓(𝑥))=...

Solving the Composition 0:03:11

Solving the Composition of Two Functions

If m is 0:05:43

If m is the AM of two distinct real numbers l and n(l, n1) and G_1, G_2 and G_3 are three geometr...

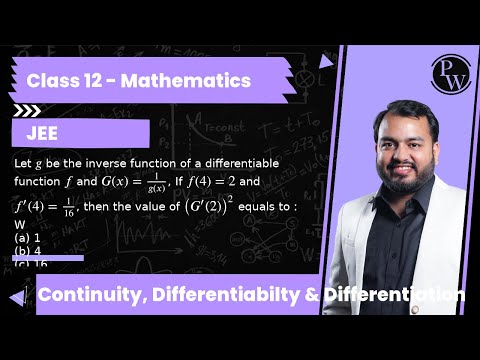Let `g` be 0:02:28

Let `g` be the inverse function of `f and f'(x)=(x^(10))/(1+x^(2)).` If `g(2)=a` then `

How to Evaluate 0:06:52

How to Evaluate Functions? Evaluating Function - General Mathematics

Operation on Functions 0:08:06

Operation on Functions f(x) = 2x + 1 g(x) = x² - 4 (General Mathematics)

Composition of Functions 0:05:58

Composition of Functions - Grade 11 - General Mathematics

Let `A ={1,2,3,4}. 0:03:37

Let `A ={1,2,3,4}. ` Let ` f : A to A ' and ' g : A to A` defined by `f : ={(1,4),...

Prove that f(x^(-1)) 0:04:09

Prove that f(x^(-1)) = f(x)^(-1) for a Group Homomorphism

for any graph 0:14:20

for any graph G, the chromatic no. is less than or equal to the max. deg. of G + 1

What does the 0:14:42

What does the Muon g-2 experiment tell us?

Let g be 0:03:56

Let g be the inverse function of a differentiable function f and G(x)=1/g(x), If f(4)=2 and f^'...

How Much G-Force 0:00:43

How Much G-Force Can a Person Withstand?