filmov
tv
Definition of the Kernel of a Group Homomorphism and Sample Proof
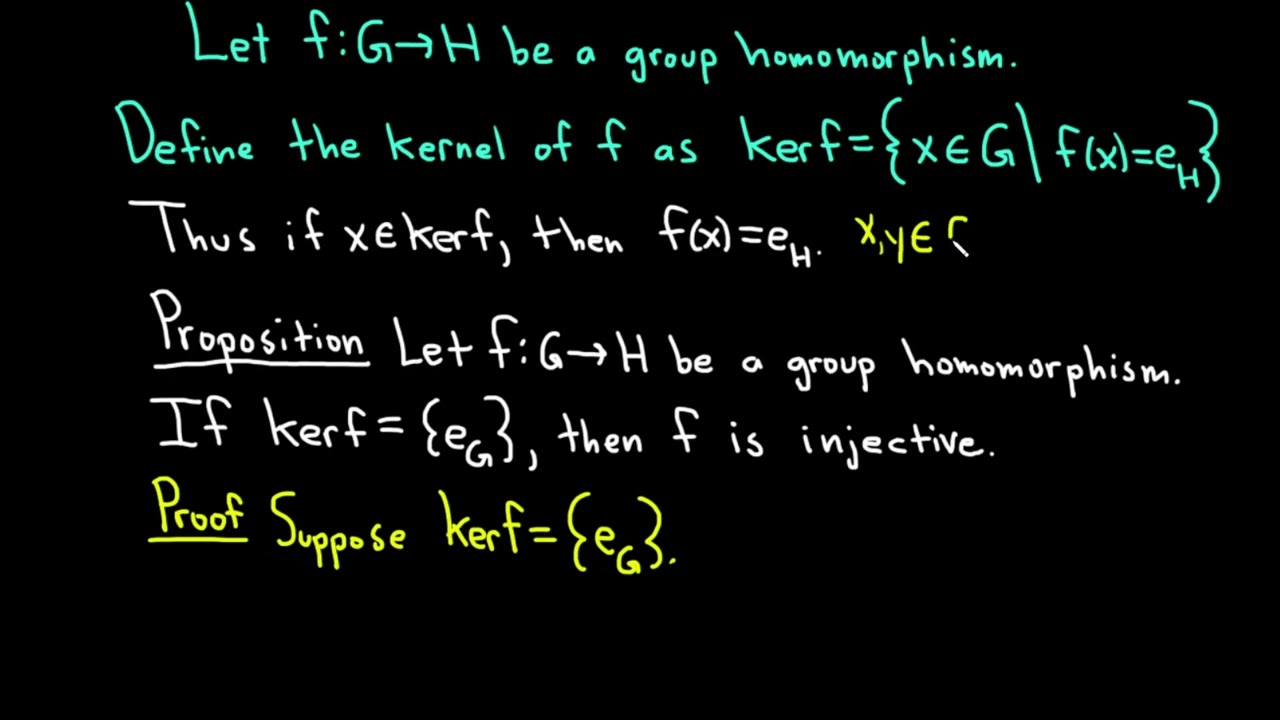
Показать описание
Let f be a group homomorphism from G into H. We define the kernel of f as kerf = {x in G | f(x) = e_H} where e_H is the identity in H. Thus is x is in kerf then we have f(x) = e_H. We prove that if the kernel of f contains only the identity element of G, then f must be an injective function. This is a simple but fun proof. I hope this helps someone learning abstract algebra!
(these are my affiliate links)
***********Math, Physics, and Computer Science Books***********
These are my affiliate links. As an Amazon Associate I earn from qualifying purchases.
If you enjoyed this video please consider liking, sharing, and subscribing.
There are several ways that you can help support my channel:)
************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course
Advanced Calculus Course
Calculus 1 Course
Calculus 2 Course
Calculus 3 Course
Calculus 1 Lectures with Assignments and a Final Exam
Calculus Integration Insanity
Differential Equations Course
Differential Equations Lectures Course (Includes Assignments + Final Exam)
College Algebra Course
How to Write Proofs with Sets Course
How to Write Proofs with Functions Course
Trigonometry 1 Course
Trigonometry 2 Course
Statistics with StatCrunch Course
Math Graduate Programs, Applying, Advice, Motivation
Daily Devotionals for Motivation with The Math Sorcerer
Thank you:)
(these are my affiliate links)
***********Math, Physics, and Computer Science Books***********
These are my affiliate links. As an Amazon Associate I earn from qualifying purchases.
If you enjoyed this video please consider liking, sharing, and subscribing.
There are several ways that you can help support my channel:)
************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course
Advanced Calculus Course
Calculus 1 Course
Calculus 2 Course
Calculus 3 Course
Calculus 1 Lectures with Assignments and a Final Exam
Calculus Integration Insanity
Differential Equations Course
Differential Equations Lectures Course (Includes Assignments + Final Exam)
College Algebra Course
How to Write Proofs with Sets Course
How to Write Proofs with Functions Course
Trigonometry 1 Course
Trigonometry 2 Course
Statistics with StatCrunch Course
Math Graduate Programs, Applying, Advice, Motivation
Daily Devotionals for Motivation with The Math Sorcerer
Thank you:)
Комментарии
 0:04:53
0:04:53
 0:05:38
0:05:38
 0:01:01
0:01:01
 0:05:52
0:05:52
 0:09:00
0:09:00
 0:00:36
0:00:36
 0:05:35
0:05:35
 0:00:46
0:00:46
 2:12:14
2:12:14
 0:00:16
0:00:16
 0:00:33
0:00:33
 0:02:21
0:02:21
 0:01:24
0:01:24
 0:00:37
0:00:37
 0:03:07
0:03:07
 0:06:48
0:06:48
 0:07:58
0:07:58
 0:00:30
0:00:30
 0:07:19
0:07:19
 0:05:47
0:05:47
 0:12:04
0:12:04
 0:01:00
0:01:00
 0:10:01
0:10:01
 0:03:54
0:03:54