filmov
tv
Quotient Rule Proof
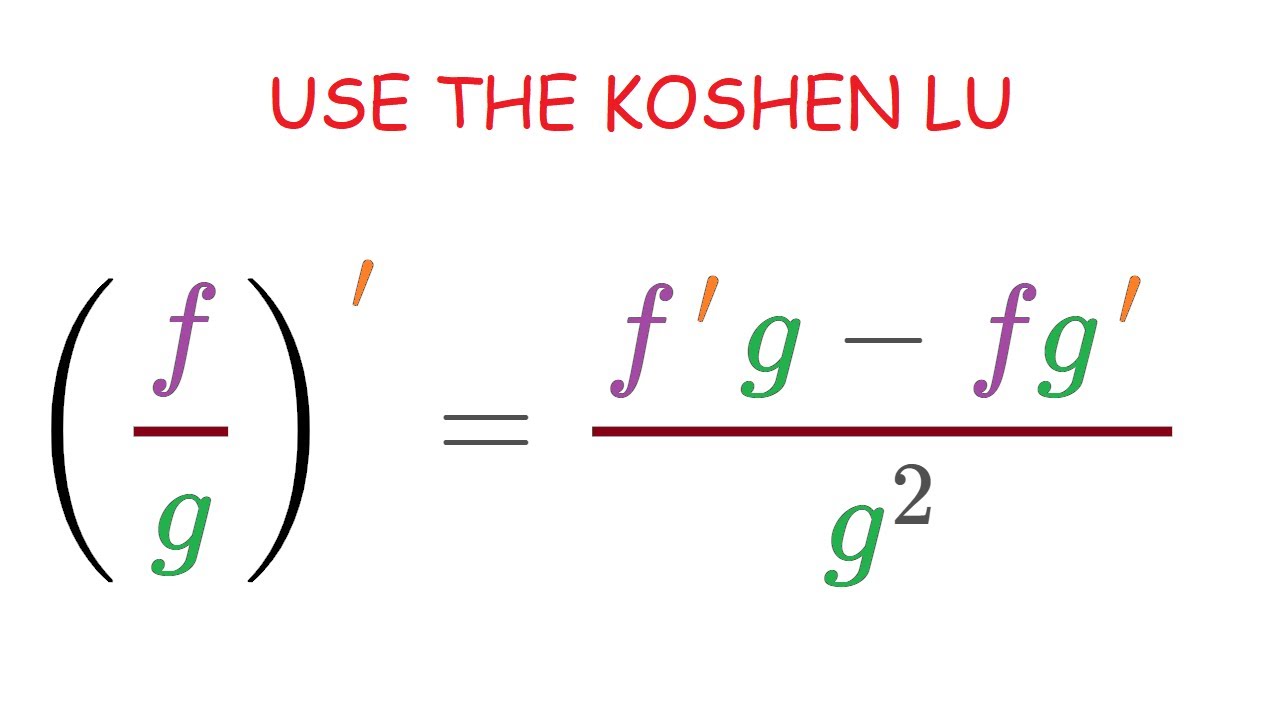
Показать описание
Proof of the quotient rule from calculus, using the definition of the derivative. This is a must-see for calculus lovers, enjoy!
Quotient Rule Proof
Proof of The Quotient Rule
Quotient Rule Proof
Quotient rule from product & chain rules | Derivative rules | AP Calculus AB | Khan Academy
Quotient Rule Proof: How to Prove The Quotient Rule from the Product Rule and Chain Rule
COOL Quotient Rule Proof
Proof of Quotient Rule
How to derive the QUOTIENT RULE from scratch
PROOF of Quotient Rule of Differentiation
a unifying product quotient rule proof
The Quotient Rule - Intuitive Proof
quotient rule proof 1st principles 20016
Proving the Quotient Rule of Differentiation #shorts
How do we prove the quotient rule? - Week 5 - Lecture 11 - Mooculus
Deriving the Quotient Rule from first principles
Proof of the Quotient Rule
Quotient Rule
Quotient Rule For Derivatives
Quotient Rule for Derivatives - Proof (Using the Product and Reciprocal Rule)
Calculus 3.07i - The Quotient Rule
Proof of Quotient Rule in Differentiation
Quotient Rule Proof - Understanding the Derivative Formula
Quotient rule proof using the chain and product rules - Calculus
Quotient rule | Derivative rules | AP Calculus AB | Khan Academy
Комментарии
 0:05:05
0:05:05
 0:06:53
0:06:53
 0:07:36
0:07:36
 0:05:15
0:05:15
 0:04:44
0:04:44
 0:04:53
0:04:53
 0:07:46
0:07:46
 0:05:33
0:05:33
 0:06:16
0:06:16
 0:03:22
0:03:22
 0:06:11
0:06:11
 0:09:26
0:09:26
 0:00:55
0:00:55
 0:05:02
0:05:02
 0:09:38
0:09:38
 0:04:34
0:04:34
 0:08:13
0:08:13
 0:11:55
0:11:55
 0:03:08
0:03:08
 0:10:59
0:10:59
 0:06:24
0:06:24
 0:13:06
0:13:06
 0:08:06
0:08:06
 0:03:52
0:03:52