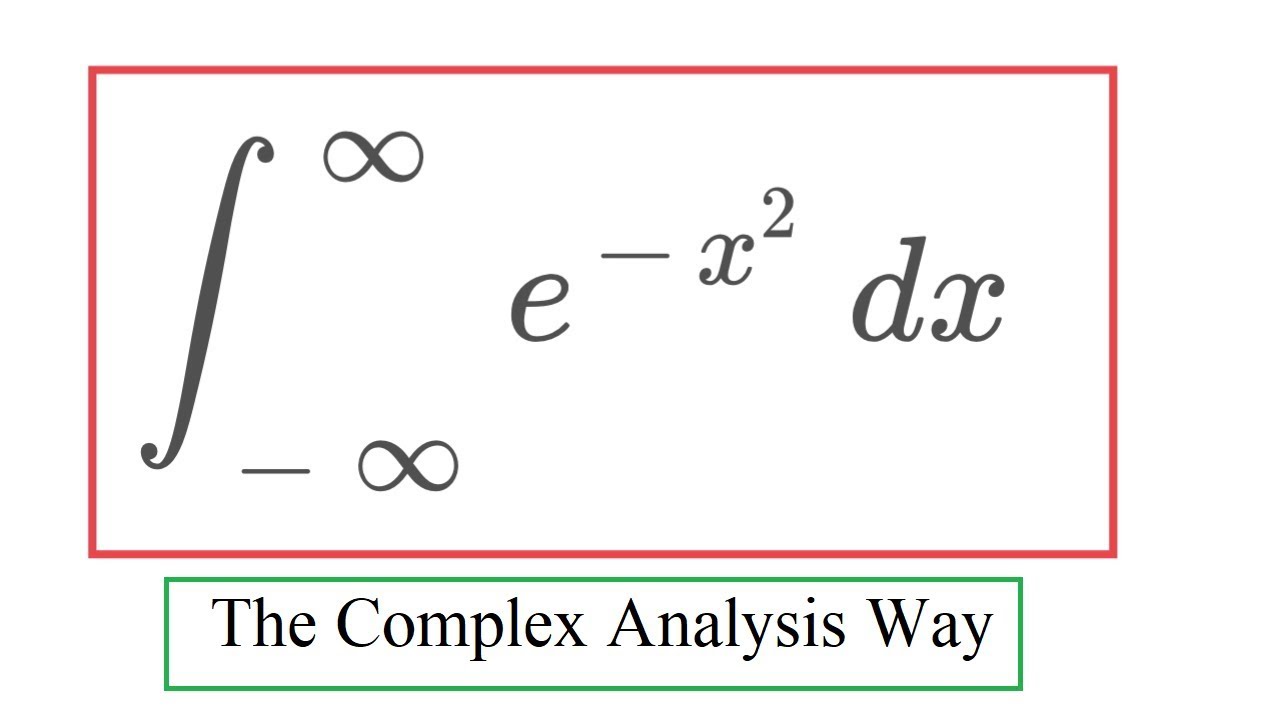
Gaussian Integral 11 Complex Analysis

Welcome to the awesome 12-part series on the Gaussian integral. In this series of videos, I calculate the Gaussian integral in 12 different ways. Which method is the best? Watch and find out!

In this video, I use complex analysis to calculate the Gaussian integral. More precisely, I integrate the function e^(-z^2/2)/(1 + e^(-tau z)) over a thin rectangle (tau is a fixed constant) using residues. At the end of the video, I show you how I found the function f and the constant tau. This is a must-see for residue-lovers, enjoy!

[ozzyfromspace]

This was informative to me for the following reason: it demonstrated that we can create designer functions that do what we want on a given contour. That's what I got from the last 10 minutes or so. Also, the gaussian function is analytic, so it's hard to evaluate because it's path integrals are usually closely related to zero. This, the designer element of complex analysis here is to construct functions with the right poles so that you can generate residues and solve your functions. I kinda glossed over the solution process, but seeing you design f(z) at the end was the real highlight. Thanks, Dr. Peyam!

[frozenmoon]

Complex analysis finally came! Great job, Dr. Peyam!

[slavinojunepri]

Designing the analytic function to finally arrive at the standard gaussian integral is my biggest takeaway from this video. Imposing the integral of such function on the lateral sides of the rectangle simplifies the functional design, making it look even more artistique. Thank you Dr. Peyam!

[emanuelmartinez]

It's amazing how complex analysis is connected to almost the whole math!...BTW great video Dr....I'm a really fan of this videos :)

[alejandrojimenez]

One of the most fun problems I have done thus far in math, I remember bashing my head against a wall (figuratively) trying to figure the f(z) to start the problem, but once I did I knew it was possible and was able to finish it up lol

[tomokinagatsuka]

It’s finally over.
I had quite a time!
What will u do next?

[sofianeafra]

How to calcul the integral from 0 to 1 of ln(x) ? Please 🙏

[tomatrix]

At 16:00 why did you sub R-t as simply t and not U, or is there some obvious reason I am missing (as a newcomer to this topic)
Edit: you explained it was change of variable after, all understood!

[LouisEmery]

7:00 Yeah, there are 31 minutes left to the video for the 4 line integrals.

[mohdmohsin]

How can we draw rectangular using function reply plz....

[lebecccomputer]

You’ve gotta use complex analysis to integrate x^-x from 0 to 1!!!

[Rundas]

Absolute monstrosity of an integral.
I'm still wondering what could be able to top this.
Maybe the fundamental theorem of engineering?