THE GAUSSIAN INTEGRAL

➡️ The Gaussian integral plays a central role in mathematics and statistics, especially in relation to the normal distribution, which is represented by the Gaussian bell curve. This curve, which takes its name from its characteristic shape, describes the probability distribution of many natural phenomena and is of crucial importance for probability theory and statistics.

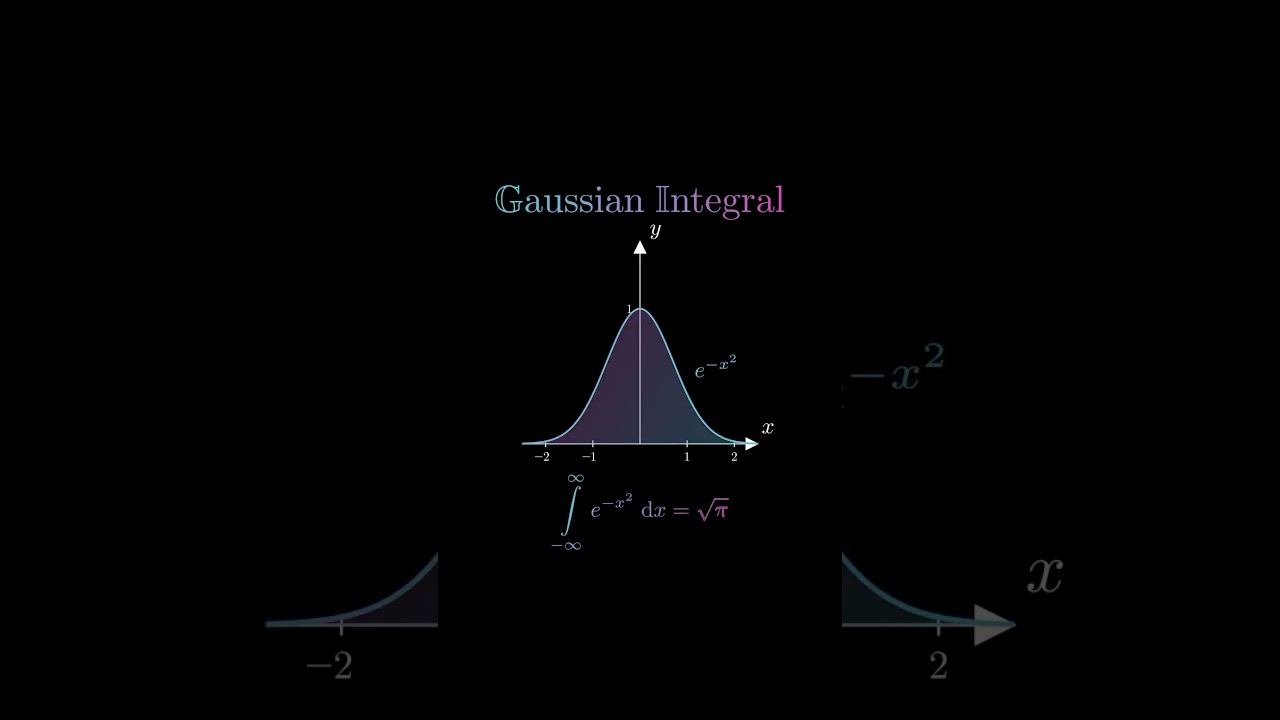
The Gaussian bell curve has a symmetrical shape and is described by an exponential function that decreases from a maximum in the center. The area under this curve represents the totality of all probabilities, which always results in one when integrated over the entire domain of definition.

A remarkable and beautiful result of integrating the Gaussian bell curve over the entire real axis is that the integral yields the value root pi. This fact is not only mathematically elegant, but also surprising, as it establishes a direct connection between the normal distribution and the number pi. The calculation of this integral requires sophisticated techniques of analysis and illustrates the deep connections between different mathematical areas.

The result shows how certain geometric and analytical concepts can work together to lead to simple but profound mathematical truths. The realization that the integral of the Gaussian bell curve is root pi underscores the beauty of mathematics and its ability to provide insight into complex phenomena through elegant solutions. 🧮🔥
•
Follow @mathimatical idea for more🤝🏼🔥
•
•
•
•
•
——————————————————————————
#math #manim #python #mathematics #physics