filmov
tv
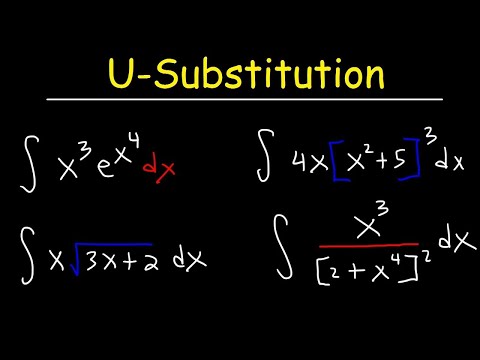
U substitution with a radical

Показать описание
👉 Learn how to evaluate the integral of a function. The integral, also called antiderivative, of a function, is the reverse process of differentiation. Integral of a function can be evaluated as an indefinite integral or as a definite integral. A definite integral is an integral in which the upper and the lower limits/boundaries are known, otherwise the integral is indefinite.
There are various formulas, depending on the function, and methods used in evaluating the integral of a function. Some of the methods includes: by direct integration, by substitution, by integration by parts, etc
Organized Videos:
✅The Integral
✅Riemann Sum Approximation
✅Evaluate Integrals
✅Find the Particular Solution
✅Find The Integral of The Expression
✅Evaluate Using The Second Fundamental Theorem of Calculus
✅Trapezoid Area Approximation
✅Integration | Learn About
✅Separated Integrals Integration
✅Find The Average Value of a Function
✅Find the Antiderivative of a Function
Connect with me:
#integration #brianmclogan
There are various formulas, depending on the function, and methods used in evaluating the integral of a function. Some of the methods includes: by direct integration, by substitution, by integration by parts, etc
Organized Videos:
✅The Integral
✅Riemann Sum Approximation
✅Evaluate Integrals
✅Find the Particular Solution
✅Find The Integral of The Expression
✅Evaluate Using The Second Fundamental Theorem of Calculus
✅Trapezoid Area Approximation
✅Integration | Learn About
✅Separated Integrals Integration
✅Find The Average Value of a Function
✅Find the Antiderivative of a Function
Connect with me:
#integration #brianmclogan
U substitution with a radical
How To Integrate Using U-Substitution
Determine Indefinite Integrals Using U-substitution: Radicals
How to apply u substitution to radicals
A Nice Integral With Radicals | U - substitution @higher_mathematics
Integral using u substitution with a radical expression
U Substitution with a definite integral of a square root
Calculus: Integration Using U-Substitution With Radical
【Multi Sub】🫨Married to a heir? Her fate turns into a shocking reversal when the ‘dead man’ awakens!👀...
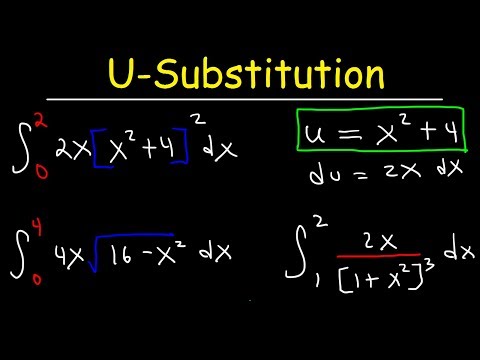
U-substitution With Definite Integrals
Evaluate the integral with the square root using u substitution
U-substitution integration with a square root
Evaluate a Definite Integral Using U-Substitution: Square Root
How to integrate when there is a radical in the denominator
Calculus - Indefinite Integrals with Radical U Substitution Guided Practice
U-Substitution 5-sample video
Integration Using The Substitution Rule
Antiderivative of function with radical using u substitution 230FRE6
How to Integrate Using U-Substitution (NancyPi)
u substitution method evaluate definite integral involving a square root (sqrt) calculus
Integrate Radical Roots Mixed Function by Substitution
YOU DECIDE: u-substitution or foil method?? #calculus #calculushelp #mathematics #integrals
U substitution integrating variable * Radical
u-Substitution: 1st Degree Rational Radical Polynomial Function Example
Комментарии
 0:06:34
0:06:34
 0:21:35
0:21:35
 0:06:22
0:06:22
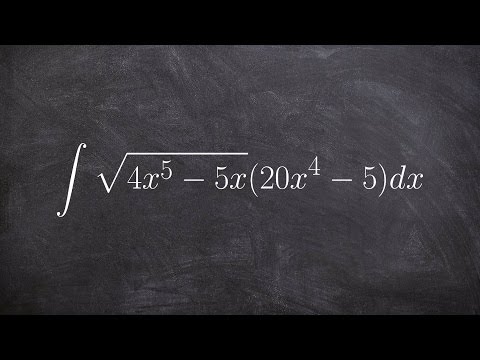 0:03:50
0:03:50
 0:05:34
0:05:34
 0:01:08
0:01:08
 0:11:33
0:11:33
 0:10:52
0:10:52
 1:52:37
1:52:37
 0:11:03
0:11:03
 0:03:25
0:03:25
 0:03:38
0:03:38
 0:05:27
0:05:27
 0:02:45
0:02:45
 0:04:38
0:04:38
 0:23:17
0:23:17
 0:10:40
0:10:40
 0:01:50
0:01:50
 0:25:48
0:25:48
 0:04:23
0:04:23
 0:06:19
0:06:19
 0:00:35
0:00:35
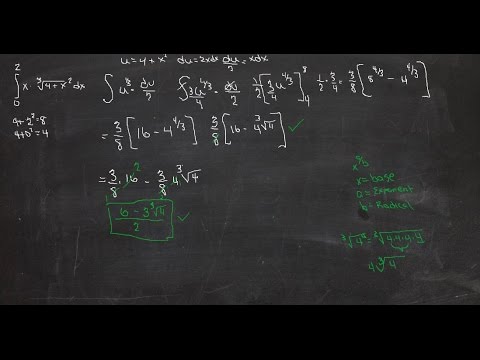 0:11:12
0:11:12
 0:08:55
0:08:55