Proving a Sequence Converges Advanced Calculus Example

preview_player
Показать описание
Proving a Sequence Converges Advanced Calculus Example.
  1. The Math Sorcerer
  2. Math
  3. advanced calculus
  4. Calculus (Field Of Study)
  5. Mathematics (Field Of Study)
  6. Sequence
Рекомендации по теме
Proving a Sequence 0:08:53

Proving a Sequence Converges Advanced Calculus Example

Proving a Sequence 0:07:57

Proving a Sequence Converges with the Formal Definition Advanced Calculus

Proving a sequence 0:05:30

Proving a sequence converges using the formal definition | Series | AP Calculus BC | Khan Academy

Proving a Sequence 0:05:42

Proving a Sequence Converges Advanced Calculus Example with {(1/n^4)}

Sequences: Proving Convergence 0:11:46

Sequences: Proving Convergence and Divergence

Proof: Sequence (3n+1)/(n+2) 0:06:53

Proof: Sequence (3n+1)/(n+2) Converges to 3 | Real Analysis

24 how to 0:12:46

24 how to prove a sequence converges

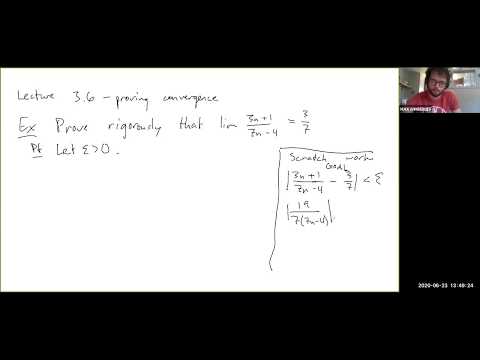
Lecture 3.6 - 0:14:34

Lecture 3.6 - Proving convergence

Proving Sequence Convergence 0:06:34

Proving Sequence Convergence

Proof: Convergent Sequence 0:05:47

Proof: Convergent Sequence is Bounded | Real Analysis

Proof: The Limit 0:13:22

Proof: The Limit of a Sequence is Unique | Real Analysis

Limit of a 0:09:52

Limit of a Sequence by Epsilon definition (Hard example)

Calculus 2 Lecture 2:27:29

Calculus 2 Lecture 9.1: Convergence and Divergence of Sequences

How to Prove 0:06:33

How to Prove a Multidimensional Sequence does Not Converge

Proof: Constant Sequence 0:06:38

Proof: Constant Sequence Converges to its Constant Value | Real Analysis

Definition of the 0:13:59

Definition of the Limit of a Sequence | Real Analysis

Convergence and Divergence 0:16:18

Convergence and Divergence - Introduction to Series

Constant sequences converge 0:06:07

Constant sequences converge

Proof that a 0:03:32

Proof that a Sequence Converges to 0 if the Absolute Value Sequence Converges to 0

How to Prove 0:03:58

How to Prove a Sequence Converges Example with 1/sqrt(n)

Using the Monotone 0:04:54

Using the Monotone Convergence Theorem to Prove a Recursive Sequence Converges: Example 1

Proof: Convergent Sequences 0:05:45

Proof: Convergent Sequences are Cauchy | Real Analysis

Proof: Limit Law 0:05:21

Proof: Limit Law for Constant Times a Convergent Sequence | Real Analysis

Convergence and Divergence: 0:09:40

Convergence and Divergence: The Return of Sequences and Series

Комментарии
Автор

I'm not sure how the last inequality follows at 2:07. What if n = 1? Then 4/sqrt(n) + 2/n = 4/sqrt(n) + 2/sqrt(n) instead of being less than.

crm
Автор

Does capital 'N' have to be a positive integer? I'm using Analysis: with introduction to proof by Lay, and he writes that a sequence converges provided that for each epsilon > 0, there exists a real number 'N' such that for any natural number 'n', n > N implies that | s_n - s | < epsilon.

mmeixner
Автор

at 2:00 why don't you square root the top number as well? why only the n?

roshnipatel
Автор

At 6:17, shouldn't it be only 'less than' instead of 'less than equal to' ?

harleendiaries
Автор

What are the n's for and where do they relate and why do they matter???

kagayakuangel
Автор

idk why we had to add 1 tho. otherwise great explanation

sundaymanali
Автор

I don't see how your +1 thing is necessary... n needs to be greater than (6/eps)^2. As these are sequences were are dealing with n needs to be greater than the next integer greater or equal to (6/eps)^2. That integer is ceil((6/eps)^2). I don't see where we need the +1 in that.

ElizaberthUndEugen