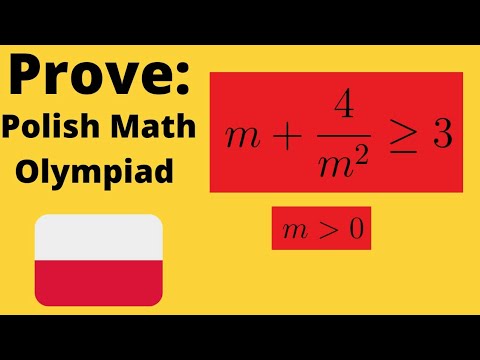
An Olympiad Problem from Poland 🇵🇱

🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡

When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
You can find ARML books and many others here. CHECK IT OUT!!! ❤️ ❤️ ❤️
❤️ f(x + y) - f(x - y) = f(x)f(y) Poland MO

If you need to post a picture of your solution or idea:
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries

#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva

PLAYLISTS 🎵 :

[krishnanadityan]

After all the arguments, we prove that f(x)=0

[sngmn]

Replace y with -y and with given eqn we will get a system:
{ f(x+y) - f(x-y) = f(x)f(y)
{ f(x-y) - f(x+y) = f(x)f(-y)
Sum these eqns:
0 = f(x)(f(y) + f(-y))
Suppose f(x) = 0 then its done.
Suppose f(y) + f(-y) = 0 then f(y) - odd function, stop what? But we already prove that f(x) is even(at 4:11) ))) So f(x) and even and odd function. What function it can be? Right, f(x) = 0.

[ChristopherEvenstar]

I was able to follow the steps. It went great! I love watching the functional equation videos. I feel like my brain gets more flexible. Thanks!

[grchauvet]

I hoped there was a non-trivial solution for some other field - e.g. one of characteristic 2, but the proof is even quicker: For any x, we have 0 = f(0)-f(0) = f(x+x)-f(x-x) = f(x)^2, and hence f(x) = 0.

[cicik]

- substitute 0, 0 : f(0) - f(0) = f²(0) => f(0) = 0
- substitute 0, n:f(n) -f(-n) = f(0)f(n) =0 so f(n) = -f(n), so f is symmetrical

- substitute x = a+b, y = a-b:
f(a) * f(b) = f(2x) - f(2y) = f(b+a)(b-a) (because f is symmetrical) = f(2y) - f(2x) so
2f(2x) = 2f(2y) or f is constant, but we figured out above that f = 0
- answer: f(anything) = 0

[majora]

I finished up from step 4 in a slightly different way.

• -f(2x) = f(x) * f(-x)
• -f(2x) = f(-x) * f(-x) from step 2
• -f(2x) = [f(-x)]^2
• -f(2x) = f(-2x) from step 3


Previously we deduced that f(x) is even, and we just now deduced f(x) is odd too. The only function that's both even and odd at the same time is f(x) = 0.

[Cow.cool.]

i found that f(x) was zero but kept trying because i thought there was another solution. Its like a math rickroll

[Sparkles.]

Could you please say, how one would know that the answer satisfies the condition...Thank you😊

[davidsousaRJ]

If we substitute y = 0 and keep x we get f(x) = 0 as a solution since the beginning. But then we have to keep trying other substitutions until we verify no other solutions are possible.

[orchestra]

Beautiful👍 I did it in a bit different way though. I figured out that f(0)=0, but missed out on the even/odd function argument
However, I noticed that if we put y=∆x and ∆x->0, then in the limit the LHS gives 2∆x*f'(x) and the RHS gives f(x)*f'(0)*∆x, because This all of course rests on the assumption that this limit exists, and f is differentiable at x.
Then, we have 2 f'(x) = f(x)*f'(0). The solution of this ODE is f(x) = f(0)*exp(f'(0)*x/2). But because f(0)=0, then f(x) = 0 for all x.

[paulortega]

So close. If it had only been f(x+y) + f(x-y) = f(x)*f(y).

[kengo]

f(0)=0
(f(x+h)-f(x-h))/(2h) = f(x)/2 * f(h)/h
h -> 0
f’(x) = f(x)/2 * (f(h) - 0)/(h - 0) = f(x)/2 * (f(0 + h) - f(0))/(h - 0) = f(x)/2 * f’(0)
But when x = 0
f’(0) = f(0)/2 * f’(0) = 0 because f(0) = 0
f’(x) = 0
==> f(x) = 0

[anotherelvis]

f(x)*f(0)=f(x+0)-f(x-0)=0 => f(0)=0
f(0+y)-f(0-y)=f(y)*f(0)=0 => f(y)=f(-y)
f(x+x)-f(x-x)=f(x)*f(x) => f(2x)+0=f(x)^2
f(x-x)-f(x-(-x))=f(x)*f(-x) => -f(2x)-0=f(x)*(-f(x))= f(x)^2
So
f(2x)=f(x)^2-f(2x) => f(2x)=0

[tontonbeber]

f(x+y)-f(x-y) = f(x)f(y)
(a) y=0 => f(x)-f(x) = 0 = f(x)f(0)
=> f(x)=0 or f(y)=0 the second in inside the first, so f(0)=0
(b) x=0 => f(y)-f(-y) = f(0)f(y) = 0 => f(y)=f(-y)
(c) y=x => f(2x)-f(0) = f(2x)=f(x)²
(d) y=-x => f(0)-f(2x) = -f(2x) = f(x)f(-x) = (b) f(x)²
(c, d) => f(2x)=-f(2x) => f(2x)=0 and so f(x)=0 forall x

[leonidfedyakov]

This video should be shortened to 2 minutes. Too many words.

[andypandy]

What a useless function that is always zero.. :D

[alextang]

f(x+y)-f(x-y)=f(x)*f(y)
Put x=x, y=0
f(x)-f(x)=f(x)*f(0)
f(0)*f(x)=0
Therefore f(0)=0 or f(x)=0
In short f(x)=0 answer. 😋😋😋😋😋😋

[DutchMathematician]

The way I solved it.
In such questions it is often beneficial to insert x=0, y=0 (or both), as well as x=y and/or x=-y.

I proceeded as follows.
Fill in x=0 and y=0. This gives:
f(0)-f(0)=0=f(0)*f(0)
Hence f(0)=0.
Substituting x=y, we get (for arbitrary x):
f(2*x)-f(0)=f(x)*f(x)
or:
f(2*x)=f(x)*f(x)
This means that f(2*x) is the product of two identical real numbers, hence f(2*x) is non-negative. Since x was arbitrary, we can conclude that f(2*x) is non-negative for all x and hence the same holds for f(x).
Now substitute y=-x in the general equation. We get:
f(0)-f(2*x)=f(x)*f(-x)
or (since f(0)=0):
-f(2*x)=f(x)*f(-x)
Since we've concluded that f(x)≥0 for every x, this only can mean that f(x)=0 for every x.

[dominiquelarchey-wendling]

f(x+y)-f(x-y) = f(x)f(y)

x, y := 0 gives f(0)-f(0) = f(0)f(0), hence f(0)² = 0. Thus f(0) = 0
x := 0 gives f(y)-f(-y) = f(0)f(y) = 0. Hence f(y) = f(-y) for any y.

y := -y gives f(x-y)-f(x+y) = f(x)f(-y) = f(x)f(y) for any x, y

Thus we have for any x, y we have

f(x+y)-f(x-y) = f(x)f(y)
f(x-y)-f(x+y) = f(x)f(y)

adding both gives

0 = 2f(x)f(y) hence f(x)f(y) = 0 for any x, y

Hence with y := x, we get f(x)² = 0, thus f(x) = 0 for any x