filmov
tv
Algebraic Extensions: The Minimal Polynomial (Algebra 3: Lecture 9 Video 2)

Показать описание
Lecture 9: We started this lecture by discussing how a homomorphism between fields gives a homomorphism between polynomial rings. We saw that when the homomorphism was an isomorphism, we got an isomorphism between quotient fields. Applying a theorem from the previous lecture then gave an isomorphism between the two fields F(alpha) and F'(beta) satisfying certain properties. We then started our discussion of Algebraic Extensions. We defined what it means for an element to be algebraic over F and what it means for an extension to be algebraic. We proved that if alpha is algebraic over F then there exists a unique monic polynomial of minimal degree in F[x] having alpha as a root. This is the minimal polynomial for alpha over F. We saw that the degree of F(alpha)/F is equal to the degree of this minimal polynomial. We gave some examples of minimal polynomials. We then used these results to prove that finite extensions are algebraic.
 0:02:05
0:02:05
 0:17:35
0:17:35
 0:12:49
0:12:49
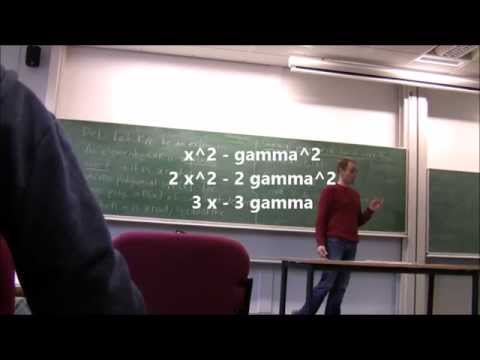 0:32:07
0:32:07
 0:08:02
0:08:02
 0:30:16
0:30:16
 0:07:43
0:07:43
 0:42:22
0:42:22
 0:05:55
0:05:55
 0:17:47
0:17:47
 0:13:49
0:13:49
 0:04:08
0:04:08
 0:05:47
0:05:47
 0:30:11
0:30:11
 0:17:16
0:17:16
 0:13:24
0:13:24
 0:52:04
0:52:04
 0:14:52
0:14:52
 0:13:37
0:13:37
 0:12:50
0:12:50
 0:09:24
0:09:24
 0:00:55
0:00:55
 0:12:00
0:12:00
 0:12:15
0:12:15