filmov
tv
A Random Number Paradox (Part 1)

Показать описание
In this short, I talk about an interesting number paradox about random numbers that I've heard before. Stay tuned next week for part 2, which will make things even more confusing!
Patreon Supporters:
Christoph Kovacs
David Johnston
Jason Killian
p11
Richard Penner
Rosario
trb
Patreon Supporters:
Christoph Kovacs
David Johnston
Jason Killian
p11
Richard Penner
Rosario
trb
A Random Number Paradox (Part 1)
A Random Number Paradox (Part 2)
The Random Number Paradox
Cover's Paradox is very cool
Simulation in R (for the birth month paradox)
The two envelopes paradox (part 2)
Bertrand's Paradox (with 3blue1brown) - Numberphile
Paradox of the drunken man
Every Weird Math Paradox - Part 2
Randomized algorithms lecture #2 - birthday paradox, random shuffle, hashing
How An Infinite Hotel Ran Out Of Room
Why “probability of 0” does not mean “impossible” | Probabilities of probabilities, part 2
More on Bertrand's Paradox (with 3blue1brown) - Numberphile
The Birthday Paradox : Probability and Statistics
A Number Theory Paradox?
Random Numbers (the next bit) - Numberphile
The Birthday Paradox: A Code in Three Acts
The Banach–Tarski Paradox
The most unexpected answer to a counting puzzle
The Hidden Paradox: Why Random Text Outperforms Novel Information
The 'Let's Make a Deal' Paradox Part 1
A 300 year old probability paradox | St. Petersburg Paradox
The Birthday Paradox
Every Math Paradox Explained - FULL Video
Комментарии
 0:00:30
0:00:30
 0:00:48
0:00:48
 0:01:34
0:01:34
 0:02:11
0:02:11
 0:03:39
0:03:39
 0:01:00
0:01:00
 0:10:43
0:10:43
 0:01:00
0:01:00
 0:14:06
0:14:06
 0:21:02
0:21:02
 0:06:07
0:06:07
 0:10:01
0:10:01
 0:23:38
0:23:38
 0:03:05
0:03:05
 0:10:52
0:10:52
 0:09:23
0:09:23
 0:13:02
0:13:02
 0:24:14
0:24:14
 0:05:13
0:05:13
 0:05:37
0:05:37
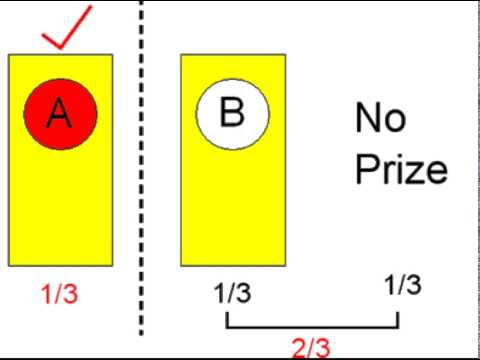 0:01:13
0:01:13
 0:11:58
0:11:58
 0:08:03
0:08:03
 0:33:01
0:33:01