filmov
tv
Limit of sin(x^2 + y^2)/(x^2 + y^2) using Polar Coordinates and L'Hopital's Rule
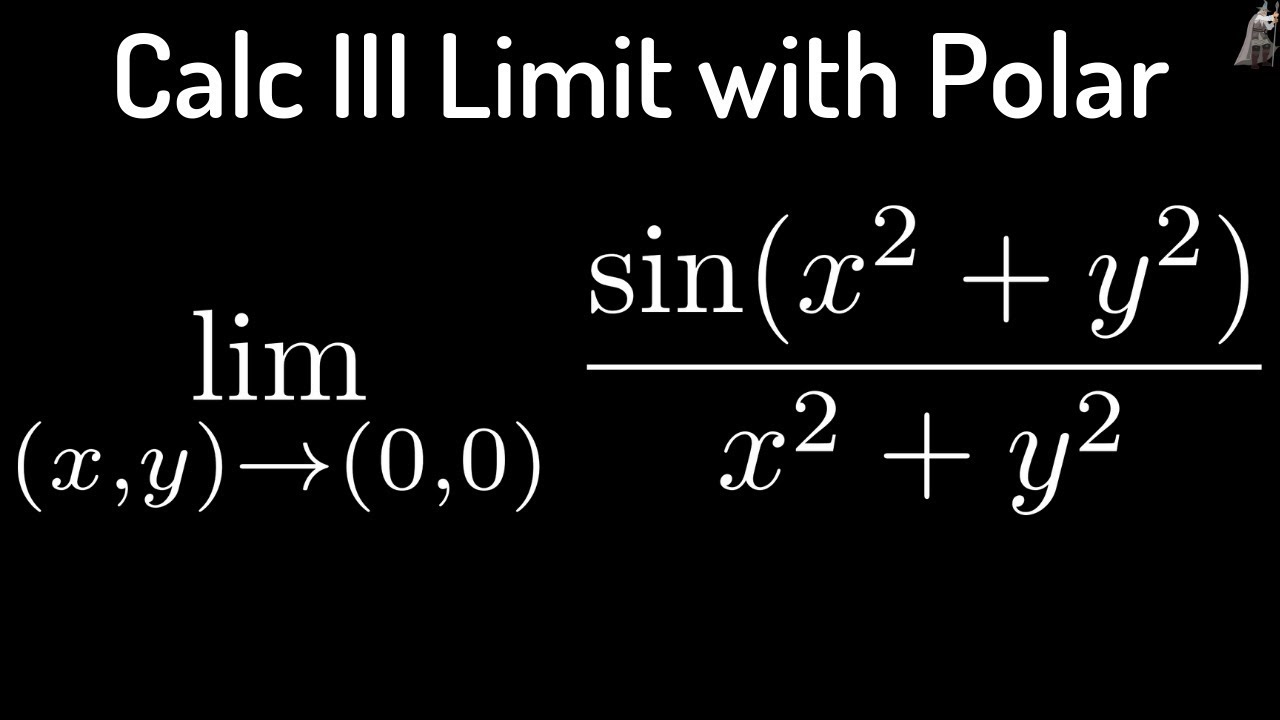
Показать описание
Limit of sin(x^2 + y^2)/(x^2 + y^2) using Polar Coordinates and L'Hopital's Rule
Limit of sin(x^2 + y^2)/(x^2 + y^2) using Polar Coordinates and L'Hopital's Rule
lim (x,y) approaches (0,0) of sin(x^2+y^2)/(x^2+y^2)
Multivariable Calculus Limit of x^2y/(x^2 + y^2) using Polar Coordinates
the value of `lim_(x- gty) (sin^2x-sin^2y)/(x^2-y^2)` equals
Multivariable Calculus Limit of y^2/(x^2 + y^2) as (x,y) approaches (0,0)
#Trigonometry all formulas
Limits of multivariable functions
Continuity and Differentiability 12 | Part 1 | One Shot in English | JEE Main & Advanced
Limit Trigonometric Function (1 - cos2x)/x^2 Half angle Formula application
How REAL Men Integrate Functions
Area bounded by: y=x^2+2, y=sin(x), x=-1, x=2 | Area under the curve | Application of Integration...
Find derivative implicitly to find equation tangent line at (0, 1/2) x^2 + y^2 = (2x^2 + 2y^2 -x)^2
When mathematicians get bored (ep1)
🟡05 - Limit and Continuity of Functions of Two Variables
sin(x^2 * y^2) = x find dy/dx using implicit differentiation
lim (x,y) approaches (0,0) of e^y sinx/x
Find derivative implicitly with respect to x for y sin (x^2) = x sin (y^2)
Limit x^2(3 + sin(x))/(x + sin(x))^2 as x approaches zero Calculus 1 Limits with sinx/x
IIT Bombay CSE 😍 #shorts #iit #iitbombay
5 simple unsolvable equations
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
when calculus students use trig identities too early
f(x,y)= (x2-y2) /(x2+y2) Show that the repeated limit exist and are unequal but the double limit .
lim [sin(x)(1-cosx))/x^2, trig limit example(watch to end to see all work in one place)
Комментарии
 0:01:54
0:01:54
 0:01:05
0:01:05
 0:03:53
0:03:53
 0:01:49
0:01:49
 0:03:06
0:03:06
 0:00:16
0:00:16
 0:11:35
0:11:35
 2:55:04
2:55:04
 0:08:13
0:08:13
 0:00:35
0:00:35
 0:11:52
0:11:52
 0:04:19
0:04:19
 0:00:37
0:00:37
 0:26:31
0:26:31
 0:02:15
0:02:15
 0:00:37
0:00:37
 0:05:45
0:05:45
 0:03:26
0:03:26
 0:00:11
0:00:11
 0:00:50
0:00:50
 0:00:15
0:00:15
 0:00:43
0:00:43
 0:08:39
0:08:39
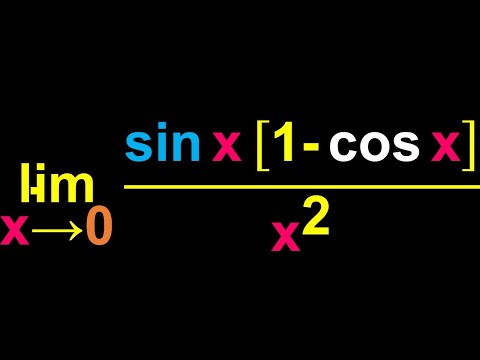 0:01:01
0:01:01