Path Connectedness

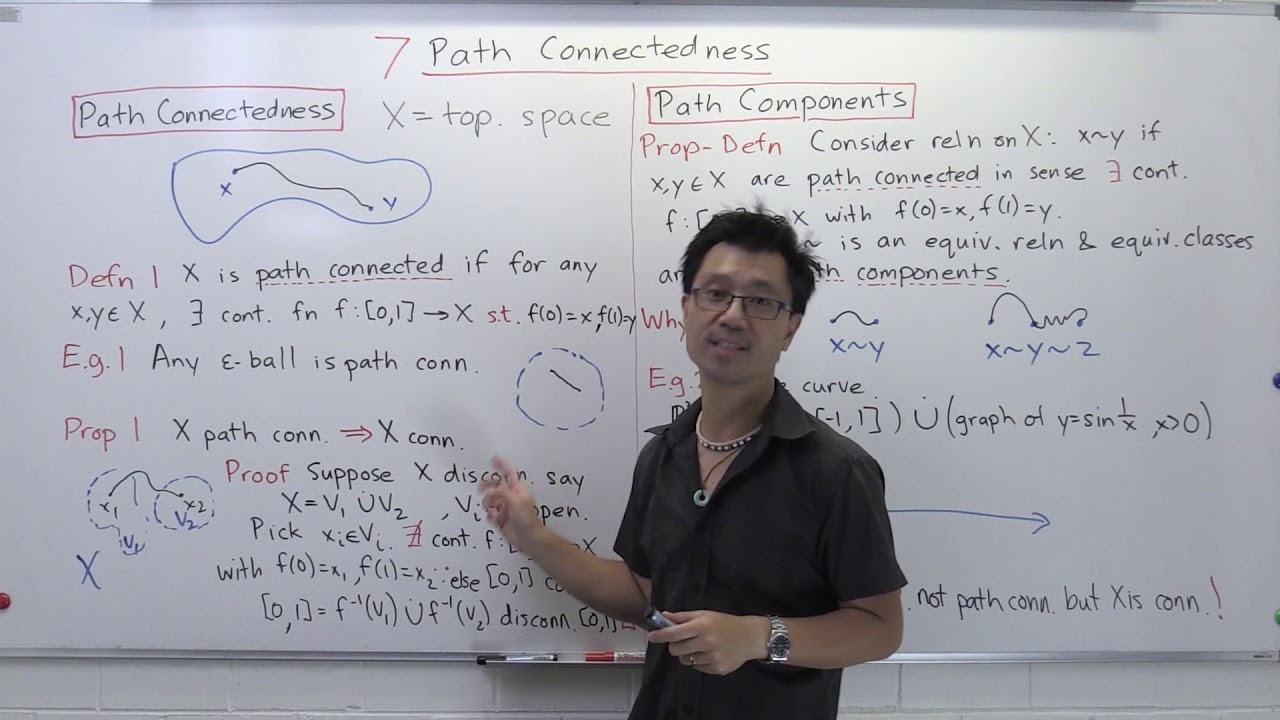
There is another natural way to define the notion of connectivity for topological spaces. It is called path connectedness to distinguish it from the notion introduced in the previous video. We examine this notion in this video. We show in particular how path connectedness implies connectedness but the converse does not hold. We look also at the notion of path components which gives a precise mathematical definition to the geometrically intuitive idea of breaking up spaces into connected pieces. We finally look at the local version of path connectedness and how local path connectedness and connectivity ensure path connectivity.

[filippogovi]

Hello,

When you prove that the path from x to z is transitive, can you really speak of a composition? I mean, as defined f:[0, 1]->X will map either to x or y, what you would have to do is show that either [0, 1] is homeomorphic to the set connecting f(0)=x to f(1)=y, or you could define a piecewise function g:[0, 2]-->y such that g(x)={(f(x), if 0\leq x < 1) and (f'(x-1), if 1 \leq x < 2), where f:[0, 1] --> X such taht f'(0)=x and f'(1)=y. Then since [0, 2] is an interval of R, we can very easily prove it is connected. Am I mistaken?

I apologise if this is completely wrong.

Cheers!

[drewduncan]

"The proof that the Topologist's Sine Curve is not path connected is left as an exercise to the viewer."

[laxmividyaclasses]

I am from India. Your explanation is very easy to understand. Thanks for this video.