Session 1: What do you mean Parametrization of a Surface and Grid curves? Examples using GeoGebra

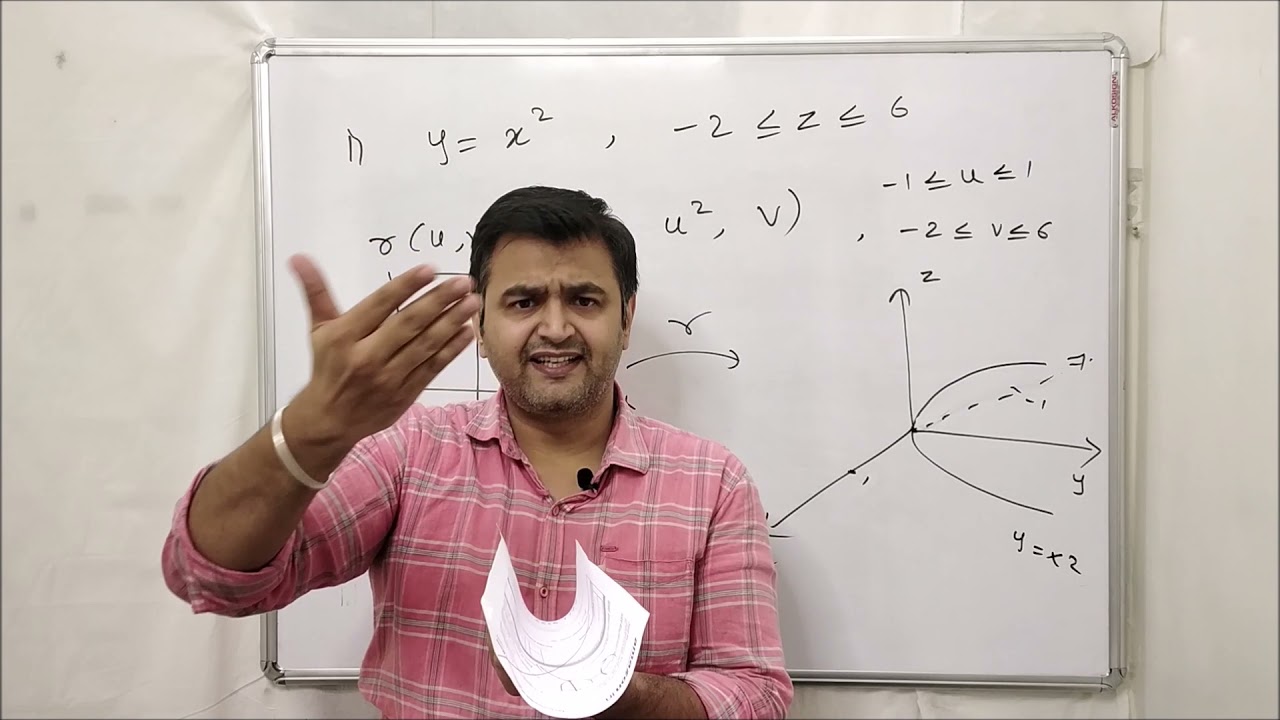
In this video, we will see what is the definition of parameterization of surfaces. Parametrization plays an important role in determining the dimension of a surface, in solving surface integral problems.

We will see plenty of examples on how to parameterize a surface. We will also see the notion of grid curves and we will also see that how GeoGebra will help us to plot the Surface and also how it help us to correct our attempt.

[charusheeladeshpande]

Two important points are missing in the whole presentation:
1. u =constant and v=constant give you two different ways of generating(looking at) the surface.
For instance you can think of cone being made up of circles of increasing radii put one on top of the other or you can think of cone as being made up of lines passing through the origin and moving along a circle.
In short a surface is made up of curves and especially u=const and v= const are called the parameter curves of the surface.
2. Surface is two dimensional object and hence will require exactly 2 parameters to describe it completely. Just as curve is one dimensional and requires only one parameter and exactly the same way if you have a solid which is 3 dimensional you will need 3 parameters !
3. Parametrization is very useful in the sense that you could look at the object in any dimensional space and parameter will remain the same. The function r that you are talking of is actually the position vector of any point on the surface. Keeping that in mind helps too.

[Amantheparadise]

Best on YouTube for higher mathematics

[rudrakshadixit]

is this a paraboloid or a parabolic cylinder?

[nihar]

Thanks sir, we all were waiting for this series

[sagarmali]

One thing is worth mentioning here, the point when you relate orthogonal trajectory, line and circle, is mind blowing !

2 ) Ans : r(u, v) = (u, v, 2u+3v-6) where u : 0 to 3, v : 0 to 4

[ashutoshmoharir]

1. u fixed: circles, v fixed: semi-circles.
2. r(u, v)=(u, v, 2u+3v-6), u:[0, 3], v:[0, 4].
3. u fixed: hyperbolas, v fixed: parabolas.
4. u fixed: ellipses, v fixed: parabolas.
5. u fixed: helixes, v fixed: lines. ???