filmov
tv
Calculus 3: Triple Integrals (8 of 25) Volume of a Rotated Curve: Method 1: Cylindrical
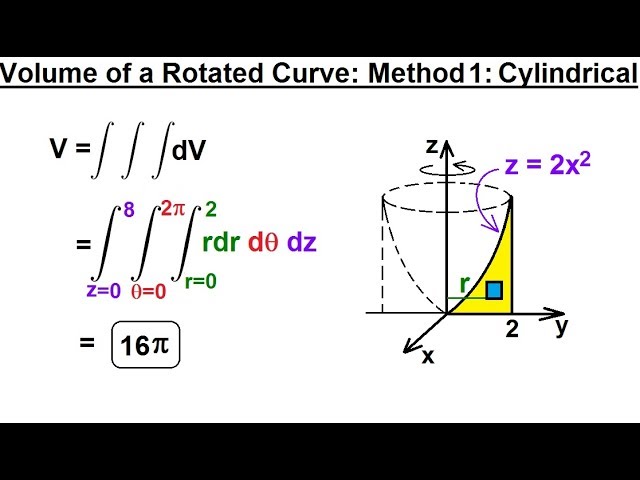
Показать описание
In this video I will find volume of a rotated curve z=2x^2 using triple integrals in the cylindrical coordinate system.
Next video in this series can be seen at:
Calculus 3: Triple Integrals (8 of 25) Volume of a Rotated Curve: Method 1: Cylindrical
Triple Integrals - Calculus 3
Calculus 3: Ch 13.1 Triple Integrals (8 of TBD) Rectangular Coordinates: A Simple Example
Calculus 3 Lecture 14.6: How to Solve TRIPLE INTEGRALS (Along with Center of Mass and Volume)
ALL of calculus 3 in 8 minutes.
Calculus 3 Lecture 14.7: TRIPLE Integrals Over Regions with CYLINDRICAL or SPHERICAL Coord.
8 Triple Integrals over More General Regions
Double and Triple Integrals
Double And Triple Integrals |01| BSc 1st Semester Maths 2nd Book Integral Calculus Unit 2 Chapter 6
Triple Integrals in Cartesian Coordinates | Volume between Surfaces
Calc 3 15.8 Notes: Triple Integrals in Spherical Coordinates
Calculus 3: Triple Integrals (1 of 25) What is a Triple Integral?
Calculus - How to find the bounds of a triple integral
Triple Integrals Practice Problems
Multivariable Calculus | Triple integral with spherical coordinates: Example.
15.6: Triple Integrals
Triple Integrals Part 2: Volume of a Tetrahedron computed 2 ways
Calculus III: Triple Integrals and Applications
Triple Integrals in Cylindrical Coordinates
What is Double integral? Triple integrals? Line & Surface integral? Volume integral? #SoME2
15.8.4: Setting Up an Integral That Gives the Volume Inside a Sphere and Below a Half-Cone
14.06.06 Finding Limits for Triple Integrals
Calculus 3: Ch 13.1 Triple Integrals (12 of TBD) Voume of a Paraboloid (Open to z-Axis)
Calculus 3: Triple Integrals in Spherical Coordinates (Video #25) | Math with Professor V
Комментарии
 0:05:14
0:05:14
 0:10:06
0:10:06
 0:06:08
0:06:08
 3:33:32
3:33:32
 0:08:10
0:08:10
 3:20:10
3:20:10
 0:06:48
0:06:48
 0:15:29
0:15:29
 0:56:30
0:56:30
 0:07:13
0:07:13
 0:23:49
0:23:49
 0:09:14
0:09:14
 0:04:56
0:04:56
 0:20:34
0:20:34
 0:14:54
0:14:54
 0:40:27
0:40:27
 0:11:44
0:11:44
 1:34:24
1:34:24
 0:20:46
0:20:46
 0:05:59
0:05:59
 0:07:51
0:07:51
 0:10:40
0:10:40
 0:08:46
0:08:46
 0:52:57
0:52:57