The Phistomefel Ring Theorem: The Beauty Hidden Within Sudokus

Hi,

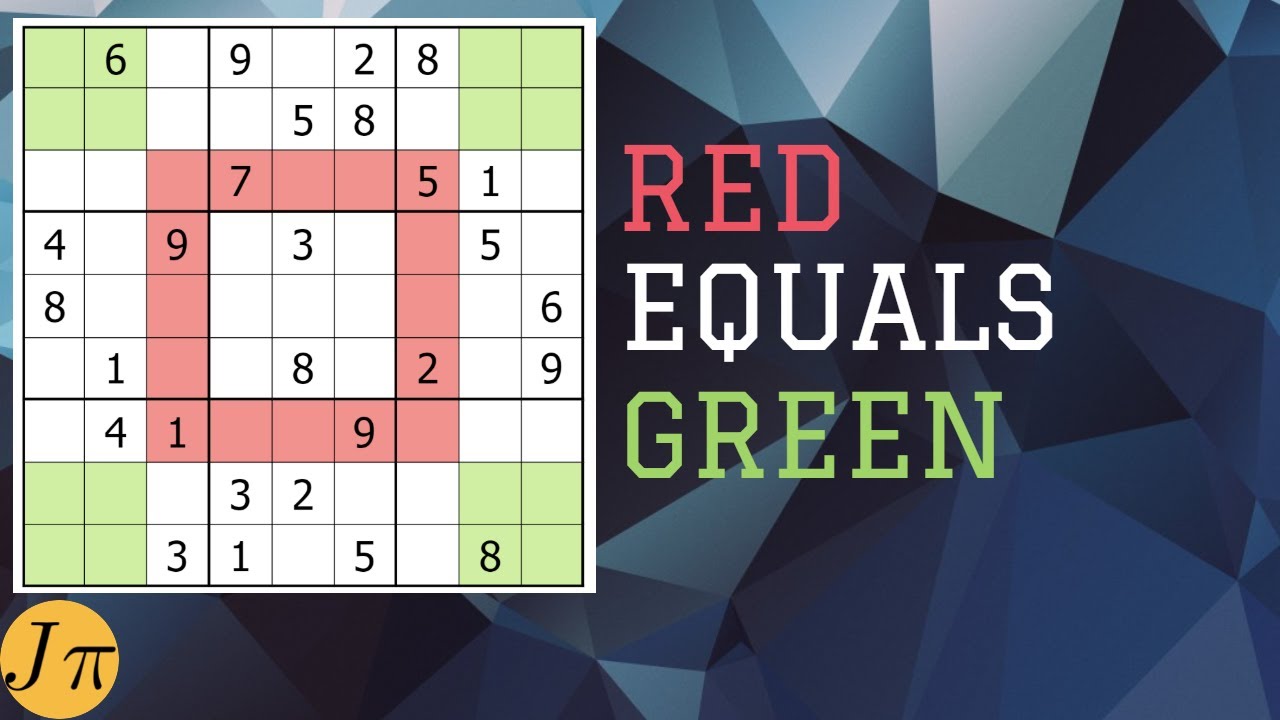
In this video I'll be proving one of my favourite theorems - the Phistomefel Ring Theorem. If you're a fan of sudoku puzzles, or logic puzzles in general, you'll love this one. This theorem states that in any complete sudoku, the 16 digits in the cells in the ring around the central box are equal to the 16 digits found in the 2x2 cells found in each corner of the grid.

Follow me on Instagram: @jpimaths

And, as always, any comments, feedback or suggestions are welcomed!

Thanks.

[matthewmtm]

Finally, a very clear and easily understandable proof. Wonderful! Thanks

[jomo]

Excellent proof and demonstration! I was astounded when I first learned this theorem, it's just so neat that it doesn't quite seem possible it could be true... Well done :)

[emilywilliams]

I think you do this very well. I have watched CTC and listened to both Simon and Mark explain this, and your explanation is equally clear (and possibly a bit briefer). Thanks.

[YellowSkarmory]

Great proof of this theorem! There are plenty of ways to prove it, and this is one that makes a lot of sense.

[astolfo]

By deduction, the right unshaded region + left unshaded region = top unshaded region + bottom unshaded region

[Taitanio]

thanks for the proof, I was wondering how to justify that step on cracking the cryptic's video

[emmanuelkazeera]

Excellent proof of the individual numbers as opposed to just the sum itself

[Trashtiel]

Mindblown by this, thank you for the explanation!

[bullish]

Thanks for this! Perfectly explained, it finally makes sense to me!

[shivpatel]

Nice video, but I think you could have done a better job explaining the rules of croquet.

[roberthanson]

Nice presentation. There is also a very simple geometric/algebraic explanation. It is based on the fact that in this case one can move the rows and columns around with no effect (as the blocks don't matter in this analysis). If we get all the green blocks together in a block in the upper left corner, and the 3x3 empty block in the bottom right corner, it's easy to see that the block forms two four-cell-wide "shadows" that must have (4-n) values each. There's still a 3x3 block with 1 value each, and the n for the green and x in the red. Then just add it up: n + (4-n) + (4-n) + 1 + x = 9. Voila! x = n. QED

[bradparker]

Great presentation. Still grappling with the concept, but that's just me. Hope you're planning more of these...

[p_square]

Red squares : R
Green Squares : G
N(R) : no. of red squares
N(G) : no. of green squares
now if we look at the figure, we can see that N(R)=16 and N(G) = 4*4 = 16 => N(R) = N(G)
is this proof correct? pretty bad proof i guess XD. Your proof was cool!