Solving a rational inequality

Показать описание

bprp fast

Рекомендации по теме

Комментарии

Answer is A
Just bring out the common denominator, combine fractions and look for critical points:
(x-1)/(x+2) > 3/4
(4x-4)/[4(x+2)] > (3x+6)/[4(x+2)]
[(4x - 4) - (3x + 6)]/[4(x+2)] > 0
(x-10)/[4(x+2)] > 0
1/4 is a positive constant, we can divide both sides by 1/4:
(x-10)/(x+2) > 0
Denominator > 0 means that its reciprocal is also > 0:
(x-10)(x+2) > 0
Critical points: -2, 10
Test: x = -3 --> (x-10)(x+2) = 13 (>0), x = 0 --> (x-10)(x+2) = -20 (not > 0), x = 12 --> (x-10)(x+2) = 28 (> 0)
Thus
x < -2 or x > 10

Yu_

Difficult for the cat 🐈 to concewtreate ! 😂😂

Needs food . . . 😂

Lolcoca

A is the answer. We can subtract 3/4 from both sides.
Simplify expression into 1 fraction
We care about:- -2, 0, 10 so we will test -3, -1, 1 and 11 in the expession to >0 and we arrive at A option.

aaryavbhardwaj

Next time, get him to solve some catculus problems 😂

peterchan

Point testing
A) try x=-3 and x=-11
-4÷-1=4>3/4 good
10÷12>.8>3/4 good
B) more specific... Check last condition
x=5
4/7<.6 a number less than .6 can not be greater than .75 bad eliminated
C) eliminated because a holds true and we lose solutions
D) eliminated same as C
E) as per B we have a point where x=\=-2 that doesn't work ELIMINATED
By process of elimination it is A.

KingGisInDaHouse

(x - 1)/(x + 2) - 3/4 > 0
[(x - 1) - 3/4(x + 2)]/(x + 2) > 0
(x - 1 - 3x/4 - 3/2)/(x + 2) > 0
(x/4 - 5/2)/(x + 2) > 0
TWO CASES:
case 1: x/4 - 5/2 > 0 and x + 2 > 0
case 2: x/4 - 5/2 < 0 and x + 2 < 0

C1: x/4 > 5/2, x > 10 and x > -2 so x > 10
C2: x/4 > 5/2, x < 10 and x < -2 so x < -2

The answer is A

anonymouscheesepie

Answer is A (cute cat btw)

We consider 2 cases.

Case 1: x + 2 > 0 ⟺ x > -2
Multiplying both sides by x + 2 gives
x - 1 > ¾(x + 2)
⟺ x - 1 > ¾x + 3/2
⟺ ¼x > 5/2
⟺ x > 20/2 = 10
x > -2 ∧ x > 10 implies x > 10

Case 2: x + 2 < 0 ⟺ x < -2
Multiplying both sides by x + 2 gives
x - 1 < ¾(x + 2)
⟺ x - 1 < ¾x + 3/2
⟺ ¼x < 5/2
⟺ x < 20/2 = 10
x < -2 ∧ x < 10 implies x < -2

Note that x + 2 ≠ 0 since (x - 1)/0 is undefined. Combining case 1 and 2 gives the final answer:
x < -2 ∨ x > 10

cyrusyeung

I wanted to write a comment, but the commenter above already wrote everything for me

Österreich-fm

Answer A first is -10 second -2
-10 < -2

saloxiddinergashev

The answer is C. If we cross multiply, we get:
4(x–1) > 3(x+2)
=> 4x – 4 > 3x + 6
=> 4x – 3x > 6 + 4
=> x > 10.

Rafsanul_Haq_

Solving a rational inequality

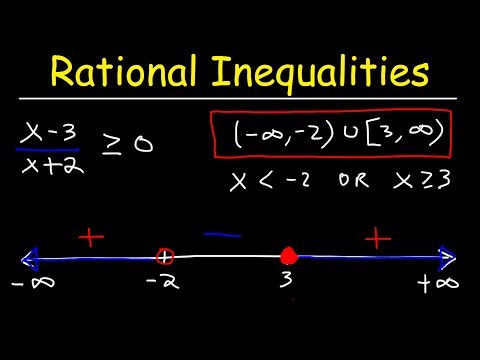
Rational Inequalities

Why solving a rational inequality is tricky!

Solving Rational Inequalities

Rational Inequalities

Solving Rational Inequalities

Leaving Cert Maths - Rational Inequalities - Worked Example

Pre-Calculus - Solving rational inequalities

SOLVING RATIONAL INEQUALITIES || GRADE 11 GENERAL MATHEMATICS Q1

Solving RATIONAL Function Inequalities: 4/(x - 3) less than 1 & 7/(x + 1) greater than 7

Solving a Rational Inequality - Example 1

Solving rational inequalities-Algebraically

Solving Rational Inequalities | General Mathematics

Rational inequalities | Polynomial and rational functions | Algebra II | Khan Academy

How to Solve Rational Inequalities? Rational Equation, Rational Inequality and Rational Function

Learn to Solve and Graph Rational Inequalities | Step-by-Step Explanation

Solving Rational Inequalities Using the Number Line and Test Intervals

How to Solve & Graph Rational Inequalities | Step-by-Step Tutorial

Ex 1: Solving Rational Inequalities

Solving Rational Inequalities (Part 1) - Number Sense 101

Solving Rational Inequalities | TAGALOG-ENGLISH

SOLVING RATIONAL INEQUALITIES | SHS GENERAL MATHEMATICS GRADE 11

Solving and Graphing Polynomial and Rational Inequalities

Solving Rational Inequalities

3.4 Solving Rational INEQUALITIES (full lesson) | MHF4U