filmov
tv
If (cosθ+isinθ) (cos2θ+isin2θ) …………(cosnθ+isinnθ)=1, then the value of θ is

Показать описание
A complex number is a number that can be expressed in the form a + bi, where a and b are real numbers, and i is a solution of the equation x2 = −1, which is called an imaginary number because there is no real number that satisfies this equation.
Iota is a greek letter which is widely used in mathematics to denote the imaginary part of a complex number. Let's say we have an equation: x^2 + 1 = 0 . In this case, the value of x will be the square root of -1, which is fundamentally not possible.
Revision Notes on Complex Numbers
z1 = a+ib and z2 = c+id then z1 = z2 implies that a = c and b = d.
If we have a complex number z where z = a+ib, the conjugate of the complex number is denoted by z* and is equal to a-ib. In fact, for any complex number z, its conjugate is given by z* = Re(z) – Im(z).
Division of complex numbers: The numerator as well as denominator should first be multiplied by the conjugate of the denominator and then simplified.
Argument of a complex number:
1. Argument of a complex number p(z) is defined by the angle which OP makes with the positive direction of x-axis.
2. Argument of z generally refers to the principal argument of z (i.e. the argument lying in (–π, π) unless the context requires otherwise.
3. Hence, the argument of the complex number z = a + ib = r (cos θ + i sin θ) is the value of θ satisfying r cos θ = a and r sin θ = b.
4. The angle θ is given by θ = tan-1 |b/a|.
If OP = |z| and arg (z) = θ, then obviously z = r (cos θ + i sin θ) and is called the polar form of complex number z.
|(z-z1) / (z-z2)| =1 the locus of point representing z is the perpendicular bisector of line joining z1 and z2.
-|z| less Re(z) less |z| and -|z| less Im(z) less |z|
If a and b are real numbers and z1 and z2 are complex numbers then
|az1 + bz2|2 + |bz1 - az2|2 = (a2 + b2) (|z1|2 + (|z2|2)
The distance between the complex numbers z1 and z2 is given by |z1 - z2|.
In parametric form, the equation of line joining z1 and z2 is given by z = tz1 + (1-t)z2.
If A(z1) and B(z2) are two points in the argand plane, then the complex slope μ of the straight line AB is given by μ = (z1 - z2)/ (1 - 2).
Two lines having complex slopes μ1 and μ2 are:
1. Parallel iff μ1 = μ2
2. Perpendicular iff μ1 = - μ2 or μ1 + μ2 = 0
If A(z1), B(z2), C(z3) and D(z4) are four points in the argand plane, then the angle θ between the lines AB and CD is given by θ = arg{(z1 - z2)/ (z3 – z4)}
Some basic properties of complex numbers:
I. ||z1| - |z2|| = |z1+z2| and |z1-z2| = |z1| + |z2| iff origin, z1, and z2 are collinear and origin lies between z1 and z2.
II. |z1 + z2| = |z1| + |z2| and ||z1| - |z2|| = |z1-z2| iff origin, z1 and z2 are collinear and z1 and z2 lie on the same side of origin.
III. The product of nth roots of any complex number z is z(-1)n-1.
IV. amp(zn) = n amp z
V. The least value of |z - a| + |z - b| is |a - b|.
Demoivre's Theorem: The theorem can be stated in two forms:
Case I: If n is any integer, then
(i) (cos θ + i sin θ)n = cos nθ + i sin nθ
(ii) (cos θ1 + i sin θ1) . (cos θ2 + i sin θ2) ......... (cos θn + i sin θn)
= cos (θ1 + θ2 + θ3 .................. + θn) + i sin (θ1 + θ2 + .............. θn)
Case II: For p and q such that q ≠ 0, we have
(cos θ + i sin θ)p/q = cos((2kπ + pq)/q) + isin((2kπ+pq/q) where k = 0,1,2,3,.....,q-1
Demoivre’s formula does not hold for non-integer powers.
Main application of Demoivre’s formula is in finding the nth roots of unity. So, if we write the complex number z in the polar form then,
z = r(cos x + isin x)
Then z1/n = [r (cos x +i sinx )]1/n
= r 1/n [ cos (x+2kπ/n) + i sin (x+2kπ/n)]
Here k is an integer. To get the n different roots of z one only needs to consider values of k from 0 to n – 1.
Continued product of the roots of a complex quantity should be determined using theory of equations.
The modulus of a complex number is given by |z| = √x2+y2.
The only complex number with modulus zero is the number (0, 0).
Some Key Points of Complex Numbers
Complex numbers is an important topic of IIT JEE. Some of the key points of IIT JEE complex numbers are listed below:
As explained, complex numbers are generally expressed as z = a+ib. Hence z is:
Purely real, if b = 0
Imaginary, if b ≠ 0
Purely imaginary, if a = 0
The set R of real numbers is a proper subset of the complex numbers.
Zero is purely real as well as purely imaginary but not imaginary.
i = √(-1), is called the imaginary unit. Also, i2 = -1; i3 = i; i4=1, etc.
√a√b√c = √(abc........)
iff at least one of a,b,c ........ is non-negative.
If j = a + ib, then a-ib is called complex conjugate of j.
Iota is a greek letter which is widely used in mathematics to denote the imaginary part of a complex number. Let's say we have an equation: x^2 + 1 = 0 . In this case, the value of x will be the square root of -1, which is fundamentally not possible.
Revision Notes on Complex Numbers
z1 = a+ib and z2 = c+id then z1 = z2 implies that a = c and b = d.
If we have a complex number z where z = a+ib, the conjugate of the complex number is denoted by z* and is equal to a-ib. In fact, for any complex number z, its conjugate is given by z* = Re(z) – Im(z).
Division of complex numbers: The numerator as well as denominator should first be multiplied by the conjugate of the denominator and then simplified.
Argument of a complex number:
1. Argument of a complex number p(z) is defined by the angle which OP makes with the positive direction of x-axis.
2. Argument of z generally refers to the principal argument of z (i.e. the argument lying in (–π, π) unless the context requires otherwise.
3. Hence, the argument of the complex number z = a + ib = r (cos θ + i sin θ) is the value of θ satisfying r cos θ = a and r sin θ = b.
4. The angle θ is given by θ = tan-1 |b/a|.
If OP = |z| and arg (z) = θ, then obviously z = r (cos θ + i sin θ) and is called the polar form of complex number z.
|(z-z1) / (z-z2)| =1 the locus of point representing z is the perpendicular bisector of line joining z1 and z2.
-|z| less Re(z) less |z| and -|z| less Im(z) less |z|
If a and b are real numbers and z1 and z2 are complex numbers then
|az1 + bz2|2 + |bz1 - az2|2 = (a2 + b2) (|z1|2 + (|z2|2)
The distance between the complex numbers z1 and z2 is given by |z1 - z2|.
In parametric form, the equation of line joining z1 and z2 is given by z = tz1 + (1-t)z2.
If A(z1) and B(z2) are two points in the argand plane, then the complex slope μ of the straight line AB is given by μ = (z1 - z2)/ (1 - 2).
Two lines having complex slopes μ1 and μ2 are:
1. Parallel iff μ1 = μ2
2. Perpendicular iff μ1 = - μ2 or μ1 + μ2 = 0
If A(z1), B(z2), C(z3) and D(z4) are four points in the argand plane, then the angle θ between the lines AB and CD is given by θ = arg{(z1 - z2)/ (z3 – z4)}
Some basic properties of complex numbers:
I. ||z1| - |z2|| = |z1+z2| and |z1-z2| = |z1| + |z2| iff origin, z1, and z2 are collinear and origin lies between z1 and z2.
II. |z1 + z2| = |z1| + |z2| and ||z1| - |z2|| = |z1-z2| iff origin, z1 and z2 are collinear and z1 and z2 lie on the same side of origin.
III. The product of nth roots of any complex number z is z(-1)n-1.
IV. amp(zn) = n amp z
V. The least value of |z - a| + |z - b| is |a - b|.
Demoivre's Theorem: The theorem can be stated in two forms:
Case I: If n is any integer, then
(i) (cos θ + i sin θ)n = cos nθ + i sin nθ
(ii) (cos θ1 + i sin θ1) . (cos θ2 + i sin θ2) ......... (cos θn + i sin θn)
= cos (θ1 + θ2 + θ3 .................. + θn) + i sin (θ1 + θ2 + .............. θn)
Case II: For p and q such that q ≠ 0, we have
(cos θ + i sin θ)p/q = cos((2kπ + pq)/q) + isin((2kπ+pq/q) where k = 0,1,2,3,.....,q-1
Demoivre’s formula does not hold for non-integer powers.
Main application of Demoivre’s formula is in finding the nth roots of unity. So, if we write the complex number z in the polar form then,
z = r(cos x + isin x)
Then z1/n = [r (cos x +i sinx )]1/n
= r 1/n [ cos (x+2kπ/n) + i sin (x+2kπ/n)]
Here k is an integer. To get the n different roots of z one only needs to consider values of k from 0 to n – 1.
Continued product of the roots of a complex quantity should be determined using theory of equations.
The modulus of a complex number is given by |z| = √x2+y2.
The only complex number with modulus zero is the number (0, 0).
Some Key Points of Complex Numbers
Complex numbers is an important topic of IIT JEE. Some of the key points of IIT JEE complex numbers are listed below:
As explained, complex numbers are generally expressed as z = a+ib. Hence z is:
Purely real, if b = 0
Imaginary, if b ≠ 0
Purely imaginary, if a = 0
The set R of real numbers is a proper subset of the complex numbers.
Zero is purely real as well as purely imaginary but not imaginary.
i = √(-1), is called the imaginary unit. Also, i2 = -1; i3 = i; i4=1, etc.
√a√b√c = √(abc........)
iff at least one of a,b,c ........ is non-negative.
If j = a + ib, then a-ib is called complex conjugate of j.
 0:02:36
0:02:36
 0:02:36
0:02:36
 0:02:15
0:02:15
 0:02:40
0:02:40
 0:03:50
0:03:50
 0:05:08
0:05:08
 0:01:59
0:01:59
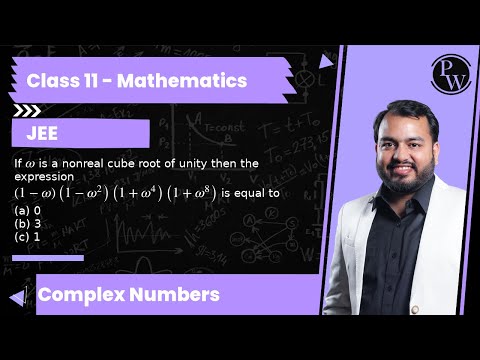 0:02:09
0:02:09
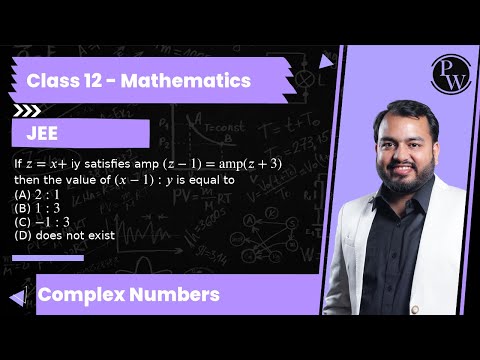 0:04:02
0:04:02
 0:04:29
0:04:29
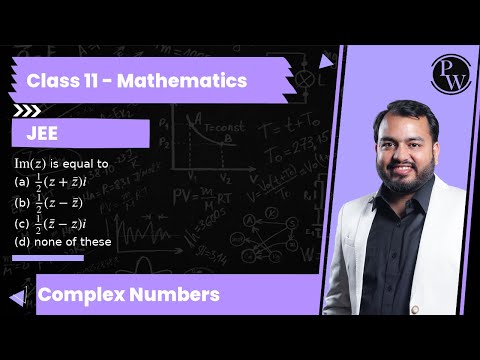 0:01:52
0:01:52
 0:06:02
0:06:02
 0:03:21
0:03:21