filmov
tv
Laplace Transforms for Partial Differential Equations (PDEs)
Показать описание
In this video, I introduce PDEs to the concept of Laplace Transforms through easy and step by step procedure.
Learn how to apply this powerful mathematical tool to solve complex PDEs and gain a deeper understanding of their behavior. Whether you're a student struggling with this topic or a math enthusiast looking to enhance your skills, this video will provide valuable insights and techniques. Join the conversation by commenting below and don't forget to subscribe for more educational content. Master Laplace Transforms for PDEs and unlock a new level of mathematical prowess today!
What is Laplace Transform?
A Laplace Transform is a specific integral transform that, when applied to a differential equation, essentially integrates out one of the independent variables to simplify the equation. Once we have solved this smaller problem, we can use tables to compute the inverse Laplace Transform and find the solution to the original differential equation.
MORE ABOUT PDEs:
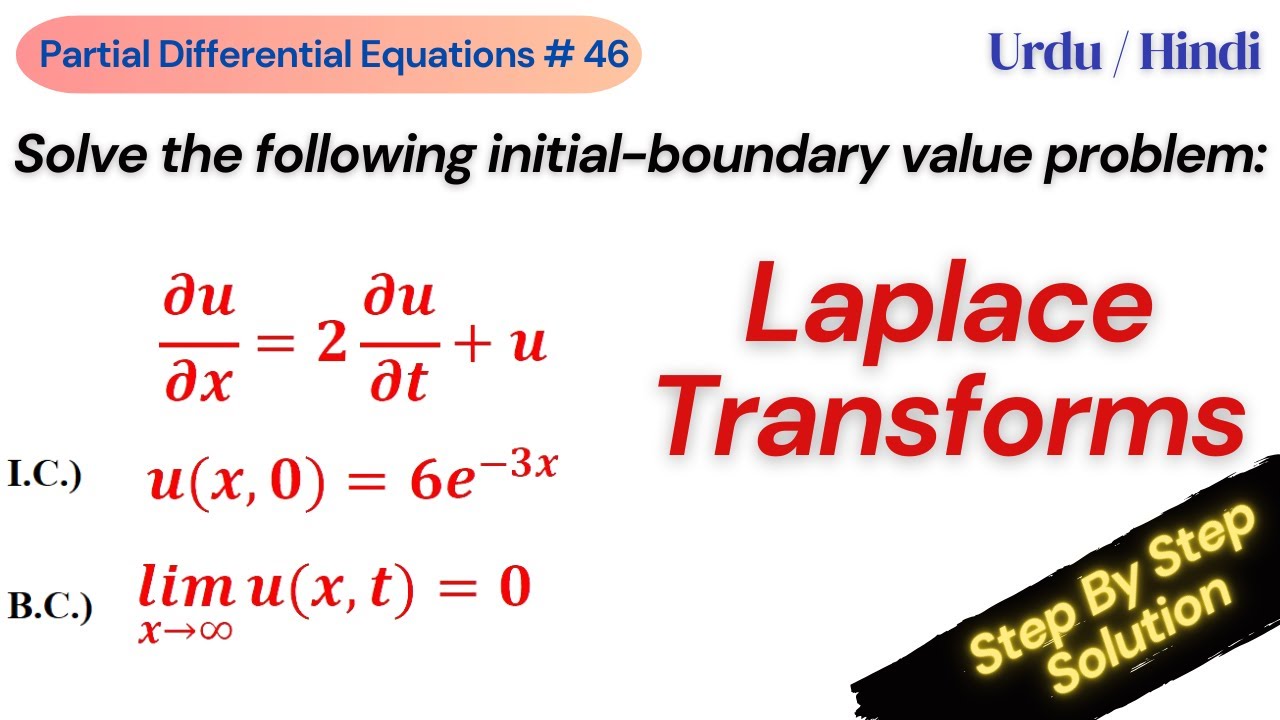
Following an introduction on Laplace Transforms, I apply the approach to a simple example utilizing a one dimensional partial differential equation on a semi-infinite domain. We arrive at our answer after some computing.
laplace transform
differential equations
inverse laplace transform
laplace transform differential equations
partial differential equations
solving differential equations using laplace transforms
differential equations laplace transform
differential equations laplace transform examples
laplace differential equation
systems of linear differential equations laplace
partial differential equation
how to use laplace transform to solve differential equations
Solution of Partial differential equations using laplace transformation
how to solve pde using laplace transform
how to solve pde using laplace transformation
laplace transform
laplace transforms
laplace transformation
Join the conversation by commenting below and don't forget to subscribe for more educational content. Master Laplace Transforms for PDEs and unlock a new level of mathematical prowess today!
My Social Media Ends:
Instagram:
Facebook:
Learn how to apply this powerful mathematical tool to solve complex PDEs and gain a deeper understanding of their behavior. Whether you're a student struggling with this topic or a math enthusiast looking to enhance your skills, this video will provide valuable insights and techniques. Join the conversation by commenting below and don't forget to subscribe for more educational content. Master Laplace Transforms for PDEs and unlock a new level of mathematical prowess today!
What is Laplace Transform?
A Laplace Transform is a specific integral transform that, when applied to a differential equation, essentially integrates out one of the independent variables to simplify the equation. Once we have solved this smaller problem, we can use tables to compute the inverse Laplace Transform and find the solution to the original differential equation.
MORE ABOUT PDEs:
Following an introduction on Laplace Transforms, I apply the approach to a simple example utilizing a one dimensional partial differential equation on a semi-infinite domain. We arrive at our answer after some computing.
laplace transform
differential equations
inverse laplace transform
laplace transform differential equations
partial differential equations
solving differential equations using laplace transforms
differential equations laplace transform
differential equations laplace transform examples
laplace differential equation
systems of linear differential equations laplace
partial differential equation
how to use laplace transform to solve differential equations
Solution of Partial differential equations using laplace transformation
how to solve pde using laplace transform
how to solve pde using laplace transformation
laplace transform
laplace transforms
laplace transformation
Join the conversation by commenting below and don't forget to subscribe for more educational content. Master Laplace Transforms for PDEs and unlock a new level of mathematical prowess today!
My Social Media Ends:
Instagram:
Facebook:
0:11:48
Solving a partial differential equation using laplace transforms
0:12:03
Laplace Transforms for Partial Differential Equations (PDEs)
0:12:32
Laplace Transforms for Partial Differential Equations (PDEs)
0:08:53
Inverse Laplace Transform Example using Partial Fractions
0:00:46
How to solve differential equations
0:09:56
Laplace Transforms: Partial Fractions (Imaginary Roots)
0:40:16
Solving PDEs with the Laplace Transform: The Heat Equation
0:25:04
Solving PDEs with the Laplace Transform: The Wave Equation
0:16:23
Laplace Transform | Application to Partial Differential Equations | GP
0:07:52
solve differential with laplace transform, sect 7.5#3
0:23:30
Solve PDE via Laplace transforms
0:20:34
Laplace Transform | Derivation of Essential Equations
0:17:46
Laplace Transform | Partial Differential Equation | Concept & Example by GP Sir
0:21:48
APPLICATIONS OF LAPLACE TRANSFORMS TO SOLUTIONS OF PARTIAL DIFFERENTIAL EQUATIONS
0:03:30
inverse laplace transform, example#4, with partial fraction
2:56:15
Ultimate Inverse Laplace Transform Study Guide
0:16:12
APPLICATIONS OF LAPLACE TRANSFORMS TO SOLUTIONS OF PARTIAL DIFFERENTIAL EQUATIONS
0:19:27
Using Laplace Transforms to Solve Differential Equations
0:09:30
Using Laplace Transforms to solve Differential Equations ***full example***
0:45:14
Solving Partial Differential Equations (PDEs) using Laplace Transforms
0:09:19
The Inverse Laplace Transform by Partial Fraction Expansion
0:04:34
inverse laplace transform, example#5, with completing the square
0:26:46
Mastering Heat Equations: Laplace Transform Solutions for Finite and Semi-Infinite Bars!
0:56:27
Inverse Laplace Transform by Partial Fraction Decomposition
Комментарии