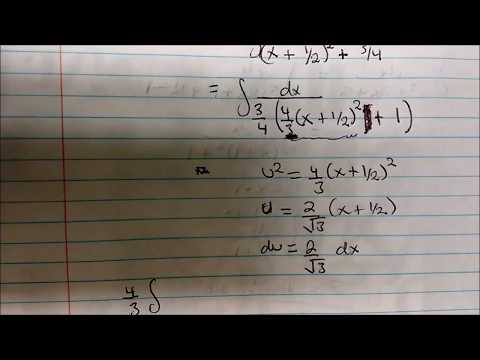filmov
tv
Integral of 1/(ax^2+bx+c) (ALL CASES 💪)
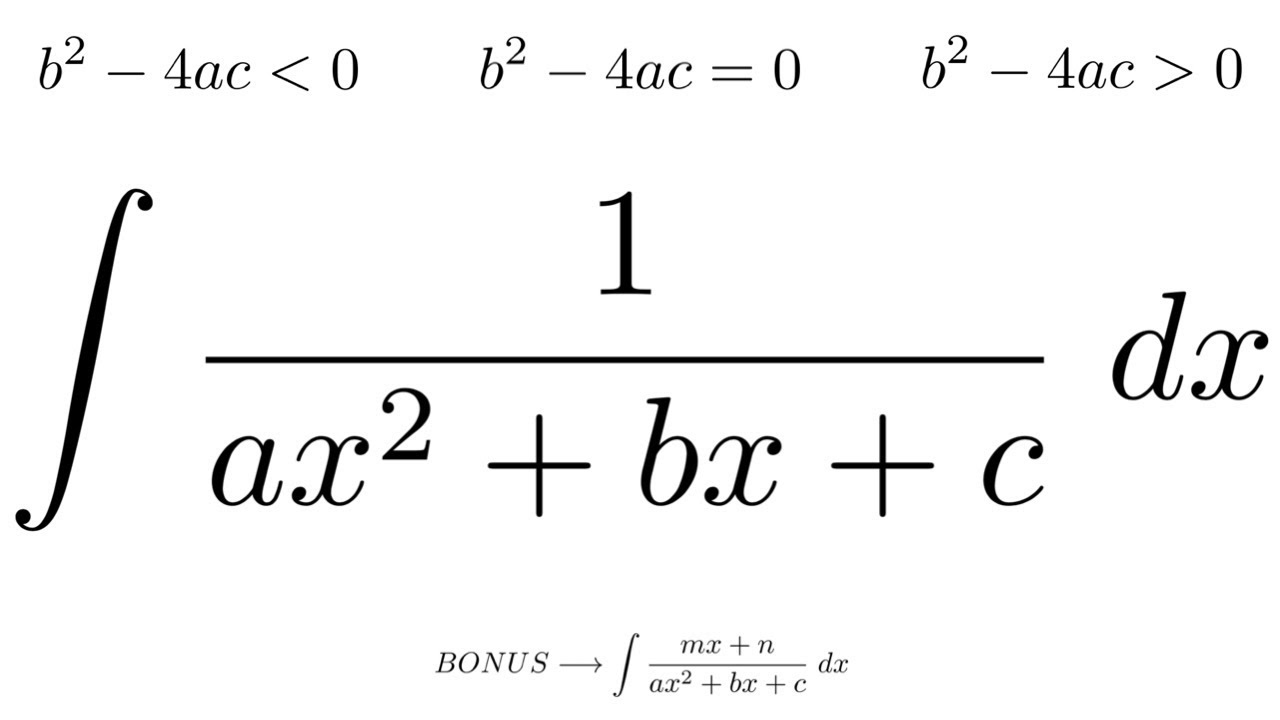
Показать описание
✍🏼 Integral of 1/(ax^2+bx+c) - How to integrate it step by step!
🚶 𝐂𝐚𝐬𝐞𝐬
00:00 Case b^2-4ac = ZERO
04:01 Case b^2-4ac = NEGATIVE
12:12 Case b^2-4ac = POSITIVE
21:27 Integral of (mx+n)/(ax^2+bx+c)
🔍 𝐀𝐫𝐞 𝐲𝐨𝐮 𝐥𝐨𝐨𝐤𝐢𝐧𝐠 𝐟𝐨𝐫 𝐚 𝐩𝐚𝐫𝐭𝐢𝐜𝐮𝐥𝐚𝐫 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥? 𝐅𝐢𝐧𝐝 𝐢𝐭 𝐰𝐢𝐭𝐡 𝐭𝐡𝐞 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥 𝐬𝐞𝐚𝐫𝐜𝐡𝐞𝐫:
🎓 𝐇𝐚𝐯𝐞 𝐲𝐨𝐮 𝐣𝐮𝐬𝐭 𝐥𝐞𝐚𝐫𝐧𝐞𝐝 𝐚𝐧 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐭𝐢𝐨𝐧 𝐦𝐞𝐭𝐡𝐨𝐝? 𝐅𝐢𝐧𝐝 𝐞𝐚𝐬𝐲, 𝐦𝐞𝐝𝐢𝐮𝐦 𝐚𝐧𝐝 𝐡𝐢𝐠𝐡 𝐥𝐞𝐯𝐞𝐥 𝐞𝐱𝐚𝐦𝐩𝐥𝐞𝐬 𝐡𝐞𝐫𝐞:
👋 𝐅𝐨𝐥𝐥𝐨𝐰 @𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥𝐬𝐟𝐨𝐫𝐲𝐨𝐮 𝐟𝐨𝐫 𝐚 𝐝𝐚𝐢𝐥𝐲 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥! 😉
𝐅𝐨𝐥𝐥𝐨𝐰 𝐈𝐧𝐭𝐞𝐠𝐫𝐚𝐥𝐬 𝐅𝐨𝐫𝐘𝐨𝐮
𝐃𝐨𝐧𝐚𝐭𝐞
#integralsforyou #integrals #integration
🚶 𝐂𝐚𝐬𝐞𝐬
00:00 Case b^2-4ac = ZERO
04:01 Case b^2-4ac = NEGATIVE
12:12 Case b^2-4ac = POSITIVE
21:27 Integral of (mx+n)/(ax^2+bx+c)
🔍 𝐀𝐫𝐞 𝐲𝐨𝐮 𝐥𝐨𝐨𝐤𝐢𝐧𝐠 𝐟𝐨𝐫 𝐚 𝐩𝐚𝐫𝐭𝐢𝐜𝐮𝐥𝐚𝐫 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥? 𝐅𝐢𝐧𝐝 𝐢𝐭 𝐰𝐢𝐭𝐡 𝐭𝐡𝐞 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥 𝐬𝐞𝐚𝐫𝐜𝐡𝐞𝐫:
🎓 𝐇𝐚𝐯𝐞 𝐲𝐨𝐮 𝐣𝐮𝐬𝐭 𝐥𝐞𝐚𝐫𝐧𝐞𝐝 𝐚𝐧 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐭𝐢𝐨𝐧 𝐦𝐞𝐭𝐡𝐨𝐝? 𝐅𝐢𝐧𝐝 𝐞𝐚𝐬𝐲, 𝐦𝐞𝐝𝐢𝐮𝐦 𝐚𝐧𝐝 𝐡𝐢𝐠𝐡 𝐥𝐞𝐯𝐞𝐥 𝐞𝐱𝐚𝐦𝐩𝐥𝐞𝐬 𝐡𝐞𝐫𝐞:
👋 𝐅𝐨𝐥𝐥𝐨𝐰 @𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥𝐬𝐟𝐨𝐫𝐲𝐨𝐮 𝐟𝐨𝐫 𝐚 𝐝𝐚𝐢𝐥𝐲 𝐢𝐧𝐭𝐞𝐠𝐫𝐚𝐥! 😉
𝐅𝐨𝐥𝐥𝐨𝐰 𝐈𝐧𝐭𝐞𝐠𝐫𝐚𝐥𝐬 𝐅𝐨𝐫𝐘𝐨𝐮
𝐃𝐨𝐧𝐚𝐭𝐞
#integralsforyou #integrals #integration
Комментарии