For p greater than 0, the vector v2 = 2i + p + 1j is obtained by rotating the vector| JEE Mains 2021

preview_player
Показать описание
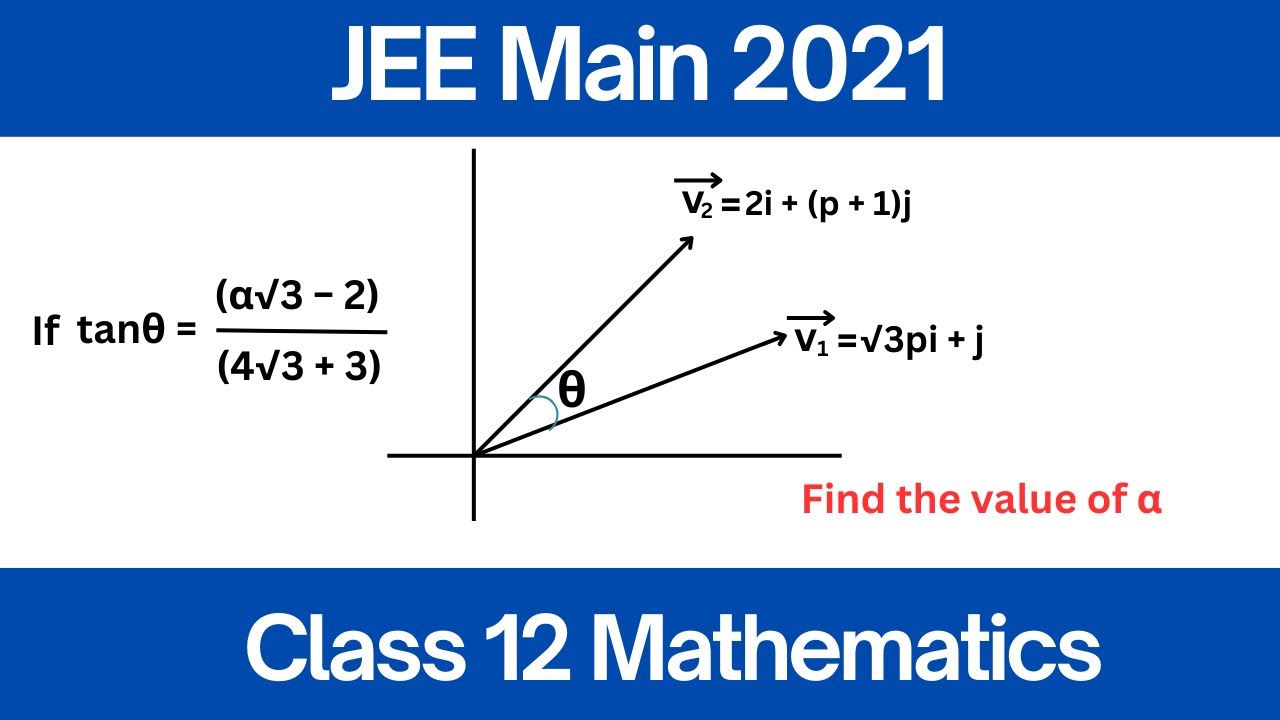
For p greater than 0, the vector v2 = 2i + (p + 1)j is obtained by rotating the vector v1 = √3pi + j by an angle θ about the origin in a counterclockwise direction. If tanθ = (α√3 − 2) / (4√3 + 3), then the value of α is equal to

In this video, we explore a vector rotation problem involving two vectors and an angle of rotation around the origin. This JEE-level problem asks us to determine the value of α based on a given trigonometric ratio of tan(θ). We break down the step-by-step approach to solve for α and provide clear explanations of each step. Watch the full video to enhance your understanding of vector rotation, angles, and trigonometry, key concepts in competitive exams like JEE.

  1. Shiwam's Classes
  2. shiwam'sclasses
  3. then the value of α is equal to
  4. JEE vector rotation
  5. vector problem JEE
  6. trigonometry JEE
Рекомендации по теме
The Lp Norm 0:09:34

The Lp Norm for Vectors and Functions

If p greater 0:06:33

If p greater than 0, then lim(1/n^p)=0

For p greater 0:02:47

For p greater than 0, the vector v2 = 2i + p + 1j is obtained by rotating the vector| JEE Mains 2021

p-Value (Statistics made 0:06:35

p-Value (Statistics made simple)

5 simple unsolvable 0:00:50

5 simple unsolvable equations

If 1 is 0:00:30

If 1 is greater than p is greater than 0 is greater than q is greater than -1, which of the...

P-Value = .000??? 0:02:41

P-Value = .000??? What to do when a p-value of .000 is reported

Question:Is p greater 0:02:51

Question:Is p greater than q?Statement1: p*q is greater than zero | UPSC | CSAT 2023 | Avishek Sinha

solve the definite 0:01:58

solve the definite integral with constant p been greater than 0

Is p greater 0:01:02

Is p greater than q? | p^3 is smaller than 0 | pq^2 is smaller than 0...

Random Variable Probability 0:05:34

Random Variable Probability Distribution P(X greater than=x) = 3P(X less than 2) IB Math

Geometric Series | 0:06:28

Geometric Series | Convergence, Derivation, and Example

Normal Distribution: Find 0:04:51

Normal Distribution: Find Probabilities Given Z-scores Using Table (Left of Z-score)

Proof and Problem 0:06:35

Proof and Problem Solving - Quantifiers Example 03

If q^2 - 0:07:38

If q^2 - 4pr =0, p grater than zero then the domain of the function f(x)=log(p x^3 +(p+q)x^2 +(q+r)

If p, q 0:00:32

If p, q are each greater than 0 but less than 1, which of the | 7338 | Experts' Global GMAT Pre...

Excel Formula: Create 0:04:06

Excel Formula: Create IF greater than or less than Function formula in Excel (Easy)

If p and 0:01:03

If p and q are each greater than 0 but less than 1, which of the following may be true...

Calculus 2 - 0:43:52

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

Nested Quantifiers (Solved 0:07:34

Nested Quantifiers (Solved Example 1)

IllegalArgumentException radius must 0:01:23

IllegalArgumentException radius must be greater than 0 | Do not use %p | Solved

Theory of Computation: 0:07:52

Theory of Computation: PDA Example (a^n b^2n)

Statistical Significance, the 0:01:59

Statistical Significance, the Null Hypothesis and P-Values Defined & Explained in One Minute

For the equation 0:01:46

For the equation 3x2+px+3=0, p greater than zero if one of the roots is square of the other, then p