filmov
tv
Solving and graphing a absolute value inequality with infinite many solutions
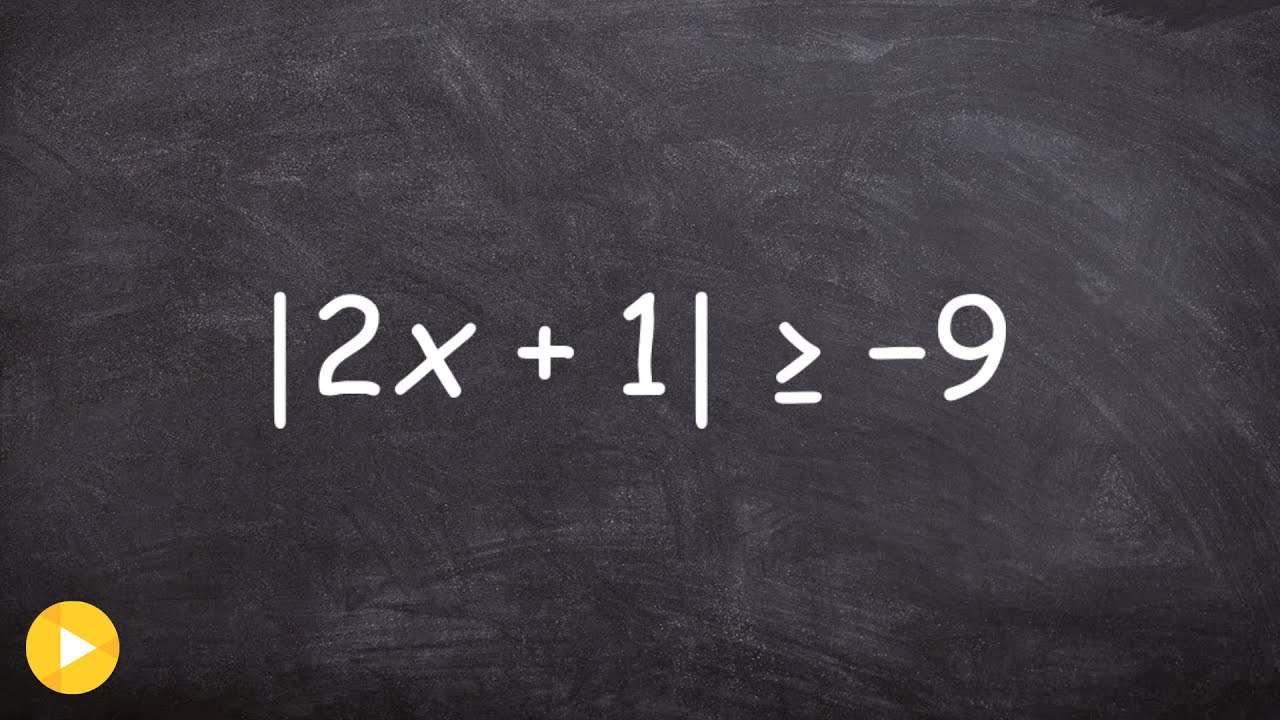
Показать описание
👉 Learn how to solve absolute value inequalities. The absolute value of a number is the positive value of the number. For instance, the absolute value of 2 is 2 and the absolute value of -2 is also 2. To solve an absolute value inequality, we create the two cases of absolute value problems: the positive case and the positive case. For the negative case, we flip the inequality sign. This results in a compound inequality that we then solve accordingly and graph our solution on a number line.
Organized Videos:
✅Solve Absolute Value Inequalities
✅Solve Absolute Value Inequalities | Easy
✅Solve Absolute Value Inequalities | Medium
✅Solve Absolute Value Inequalities | Hard
Connect with me:
#AbsoluteValue #Brianmclogan
Organized Videos:
✅Solve Absolute Value Inequalities
✅Solve Absolute Value Inequalities | Easy
✅Solve Absolute Value Inequalities | Medium
✅Solve Absolute Value Inequalities | Hard
Connect with me:
#AbsoluteValue #Brianmclogan
How To Graph Absolute Value Functions - Domain & Range
How To Solve Absolute Value Equations, Basic Introduction, Algebra
Solving and graphing an absolute value inequality
Graphing the absolute value function with transformations
Absolute Value Inequalities - How To Solve It
17 - Solving & Graphing Absolute Value Inequalities in Algebra, Part 1
Sketching Harder Absolute Value Graphs
Solving and Graphing a Double Absolute Value Inequality
Mechanical Vibrations Lecture 14
How to solve and graph an absolute value inequality with OR
Graphing an absolute value equation with transformations and a vertical stretch
Absolute value inequalities | Linear equations | Algebra I | Khan Academy
Graphs of absolute value functions | Functions and their graphs | Algebra II | Khan Academy
How To Solve Absolute Value Equations
Graphing Absolute Value Functions (y=a|x-h|+k)
Solving and graphing a absolute value inequality with infinite many solutions
Solving and graphing a two step absolute value inequality
Adding Absolute Values- Graphing
Absolute value equations | Linear equations | Algebra I | Khan Academy
Solve and graph an absolute value inequality
Solve and graph an absolute value inequality using AND
how to graph and shade an absolute value inequality by translating parent graph
Sketch Graph of Absolute Quadratic Function
Graphing Absolute Value Functions (3 of 3: Reflections & non-linear curves)
Комментарии
 0:10:54
0:10:54
 0:04:21
0:04:21
 0:06:19
0:06:19
 0:02:47
0:02:47
 0:12:40
0:12:40
 0:23:30
0:23:30
 0:09:09
0:09:09
 0:05:22
0:05:22
 0:50:13
0:50:13
 0:04:39
0:04:39
 0:03:50
0:03:50
 0:13:13
0:13:13
 0:08:34
0:08:34
 0:20:53
0:20:53
 0:06:52
0:06:52
 0:02:28
0:02:28
 0:03:28
0:03:28
 0:07:26
0:07:26
 0:10:41
0:10:41
 0:04:31
0:04:31
 0:03:08
0:03:08
 0:03:48
0:03:48
 0:04:36
0:04:36
 0:09:52
0:09:52