filmov
tv
Solve an equation by factoring large numbers

Показать описание
we find two factors of the product of the constant term (the term with no variable) and the coefficient of the squared variable whose sum gives the linear term. These factors are now placed in separate brackets with x to form the factors of the quadratic equation.
There are other methods that can be used to achive this including the AC, Berry, Box, Grouping, diamond and mental technique. Once we have factored the quadratic equation, we then apply the product property to solve our equation. When solving a quadratic equation we call our solutions the zeros, x-intercept, and roots.
Organized Videos:
✅Solve Quadratic Equations by Factoring
✅Solve Quadratic Equations by Factoring | Learn About
✅Solve Quadratic Equations by Factoring | Square Root Method
✅Solve Quadratic Equations by Factoring | Zero Product Property
✅Solve Quadratic Equations by Factoring | GCF
✅Solve Quadratic Equations by Factoring | x^2+bx+c
✅Solve Quadratic Equations by Factoring | Difference of Two Squares
✅Solve Quadratic Equations by Factoring | Perfect Square
✅Solve Quadratic Equations by Factoring | ax^2+bx+c
Connect with me:
#quadratics #solvingquadratics #brianmclogan
There are other methods that can be used to achive this including the AC, Berry, Box, Grouping, diamond and mental technique. Once we have factored the quadratic equation, we then apply the product property to solve our equation. When solving a quadratic equation we call our solutions the zeros, x-intercept, and roots.
Organized Videos:
✅Solve Quadratic Equations by Factoring
✅Solve Quadratic Equations by Factoring | Learn About
✅Solve Quadratic Equations by Factoring | Square Root Method
✅Solve Quadratic Equations by Factoring | Zero Product Property
✅Solve Quadratic Equations by Factoring | GCF
✅Solve Quadratic Equations by Factoring | x^2+bx+c
✅Solve Quadratic Equations by Factoring | Difference of Two Squares
✅Solve Quadratic Equations by Factoring | Perfect Square
✅Solve Quadratic Equations by Factoring | ax^2+bx+c
Connect with me:
#quadratics #solvingquadratics #brianmclogan
Комментарии
 0:12:29
0:12:29
 0:02:43
0:02:43
 0:09:05
0:09:05
 0:06:31
0:06:31
 0:07:19
0:07:19
 0:11:11
0:11:11
 0:06:22
0:06:22
 0:22:30
0:22:30
 0:09:22
0:09:22
 0:02:40
0:02:40
 0:05:00
0:05:00
 0:00:50
0:00:50
 0:03:47
0:03:47
 0:04:07
0:04:07
 0:09:14
0:09:14
 0:11:20
0:11:20
 0:07:34
0:07:34
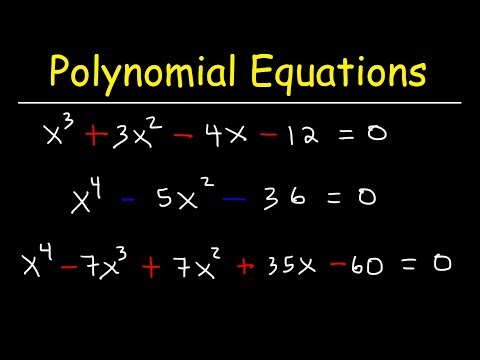 0:14:19
0:14:19
 0:03:25
0:03:25
 0:15:05
0:15:05
 0:04:28
0:04:28
 0:15:44
0:15:44
 0:04:17
0:04:17
 0:06:24
0:06:24