6 Infinite Series You Must Know For Your Calc 2 Class

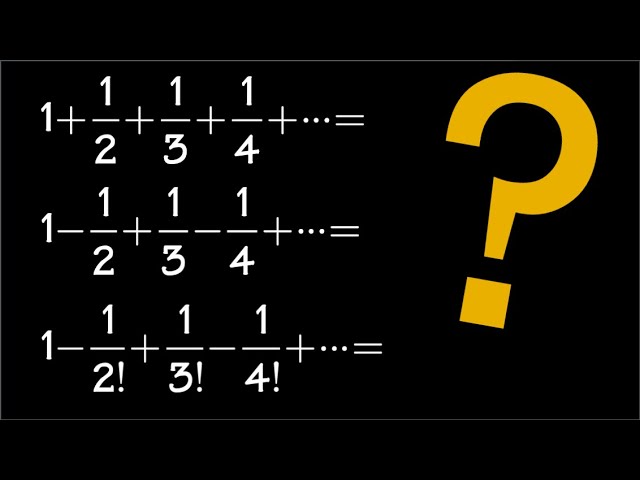
Q1. 1+1/2+1/3+1/4+1/5+... =?
Q2. 1+1/3+1/5+1/7+1/9+... =?
Q3. 1-1/2+1/3-1/4+1/5-... =?
Q4. 1-1/3+1/5-1/7+1/9-... =?
Q5. 1-1/(2!)+1/(3!)-1/(4!)+1/(5!)-... =?
Q6. 1-1/(3!)+1/(5!)-1/(7!)+1/(9!)-... =?

[sivaanand]

The thing which makes me more amazed than seeing you solving math problems... is the way you switch between black pen to red pen within a jiffy.

[dexter]

Natural log series: alternating, all natural numbers
Inverse tangent series: alternating, only odd numbers
Exponential series:
non-alternating, all factorials
Sine series:
alternating, only odd factorials

[rhversity]

Can you run through the AMC or AIME exam papers?

I want to see how a pro does it so i can do better next time

[akshatahuja]

Can you have video on all special integral like gaussian integral and many other names

[CDChester]

lol that ending smile; you knew it was devious AND LIKED

[vishwarajgohil]

For second series, Say the sum of first series be 'S' and that of second be 'T'. So what we have is "S= T + (1/2)T". Therefore it also be divergent

[JaydentheMathGuy]

My Calc Teacher: *watches this video
Also My Calc Teacher: Without solving anything in this video Ik that all of the are divergent
BlackPenRedPen: I'm about to end this man's entire career ;))

[TheWolfboy]

Whatever happened to
m i n u s
o n e
t w e l f t h

[gonzaloayancan]

1 is a small number, therefore, the exercise 6 is equal to 1

[jiaming]

Ooo i didnt think of sin's expansion for the last one, i used Im(e^i) hehe

[ffggddss]

(1) The famous Harmonic Series.
It diverges, because starting with ½, grouping terms in powers of 2, each group sums to > ½, so you have an infinite sum of terms each > ½, which is infinite.
(2) Similarly diverges, by starting with ⅓, grouping terms in powers of 2, each group sums to > ¼, so the sum is infinite.
(3) ln(2), because it's the Taylor Series for ln(1+x), with x = 1.
(4) ¼π, because it's the Taylor Series for tan⁻¹x, with x = 1.
(5) 1 – e⁻¹, because it's 1 minus the Taylor Series for e⁻ˣ, with x = 1.
(6) sin(1), because it's the Taylor Series for sin(x), with x = 1.

Interesting bonus question: We know that (1) behaves logarithmically with n, the number of terms, so that
lim [∑ᵢ₌₁ⁿ(1/i) – ln(n)] = γ, a finite value (the Euler-Mascheroni constant, 0.5772156649...)
n→∞

So what can be done of a similar nature with series (2), the odd harmonic series? Ans:
lim [∑ᵢ₌₁ⁿ(1/[2i–1]) – ln(2√n)] = ½γ (0.2886078325...)
n→∞

Fred

[kutuboxbayzan]

1:div
2:div
3:ln2
4:pi/4
5:1-1/e
6:sin (1)

[douglasstrother]

The "Euler-Mascheroni Constant" (γ) ~ Wolfram
It's pretty crazy that the difference between to diverging series is finite!
Gamma still has a host of unresolved properties, which is pretty remarkable.

[anikbhowmick]

for the 4th problem I used ln(1+x) and put x=i (complex number ) I got the nice answer .in our present syllabus we don't have this series problems but it was really amazing .moving to the complex world made things easier

[bachirblackers]

You are so so so genius . Not because you are are one of the best maths but because your detailed definition and smooth deep analysis . Wawwww . Thanks .

[hesselstokman]

I don't know if u can find an awnser with this but what i was thinking is that serie 3 can also be written as 1/(-(( - n√n)^n)
n√n as in the nth square root of n.

[JohnSmith-sxor]

I feel it’s very interesting that the similar series have quite different answer, such as No.3 and 4. 🤔

[mathmancalc]

Can you go one step further? How about "1+(1/2)!+(1/3)!+(1/4)!+..." and "1-(1/2)!+(1/3)!-(1/4)!+..."? I have never seen this and would like to know what happens.

[WhattheHectogon]

Haven't watched it yet, but off the top of my head, it's divergent, divergent, ln2, pi/4, 1/e, and I dunno the last one :) love your videos! Would really appreciate it if you checked any of my out. Keep it up!


Edit: Darn! 1 - 1/e, I forgot about that leading 1...
Also, I should've seen that was the sine taylor series...live and learn, I guess!

[aleksandervadla]

Can you explain why the harmonic series goes to ln(n) ?