filmov
tv
Mathematical Physics- Special Functions: Orthogonality of Legendre’s Polynomials
Показать описание
This is completely voluntary, these donations can help increase the quality of the videos and the lectures overall. Special Functions: Orthogonality of Legendre’s Polynomials
Integral of the product of Pn(x)and Pm(x)with respect to x between the limits-1 and +1is equal to Kronecker delta. This theorem has been defined and proved.
Integral of the product of Pn(x)and Pm(x)with respect to x between the limits-1 and +1is equal to Kronecker delta. This theorem has been defined and proved.
0:46:20
Mathematical Physics- Special Functions: Orthogonality of Legendre’s Polynomials
0:32:54
Mathematical Physics- Special Functions: Problems on Orthogonality of Legendre’s Polynomials
0:26:41
Mathematical Physics- Special Functions: Hermite Polynomials- Orthogonal Properties
0:18:40
Trig Function Orthogonality
0:11:49
Arfken 7th Edition Section 15.4 Associated Legendre Functions - Orthogonality
0:07:23
How to use Orthogonality Relations in Solving Problems from Special functions | Physics Hub
0:00:15
MATHEMATICAL PHYSICS :- LEGENDRE FUNCTIONS
0:20:53
Mathematical Physics Lecture28: Hermite polynomials from orthogonality condition
0:05:36
Legendre's Special Functions All Important Formulas, Orthogonality,DE, Recurrence Formulas
0:25:51
FROBENIUS METHOD & SPECIAL FUNCTIONS: Orthogonality of Hermite polynomials
0:30:42
FROBENIUS METHOD & SPECIAL FUNCTIONS: Orthogonality of Bessel's functions
0:16:00
Mathematical Physics- Special Functions: Laguerre Polynomials- 1
0:16:40
37. Orthogonality of Legendre Polynomial | Complete Concept and Derivation | Most Important
0:26:30
Mathematical Physics- Special Functions: Legendre’s Polynomials
0:09:03
orthogonality relation of hermite polynomials
0:09:51
The Orthogonality of Hermite Polynomials
0:15:48
Mathematical Physics- Special Functions: Hermite Polynomials- 1
0:16:00
Special function (Bessel,legendre,Hermite)
0:31:02
Mathematical Physics- Special Functions: Problems on Legendre’s polynomials- 1
0:21:19
Mathematical Physics- Special Functions: Hermite Polynomials- 2
0:16:01
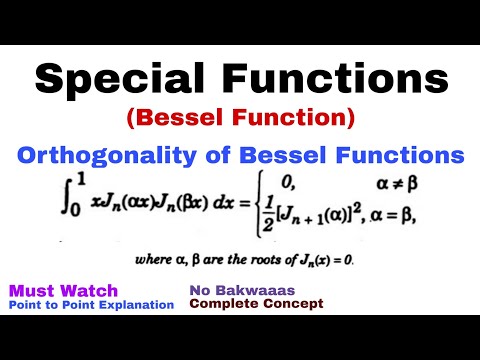
27. Orthogonality of Bessel Functions | Complete Concept | Most Important
0:13:17
Orthogonal properties of legendre's polynomial || mathematical physics|| notes
0:50:15
MCQs | M. Sc. Physics| MMP| Module III: Special Functions-Part I |SPPU
0:00:11
Legendre Polynomials
Комментарии