The Orthogonality of Hermite Polynomials

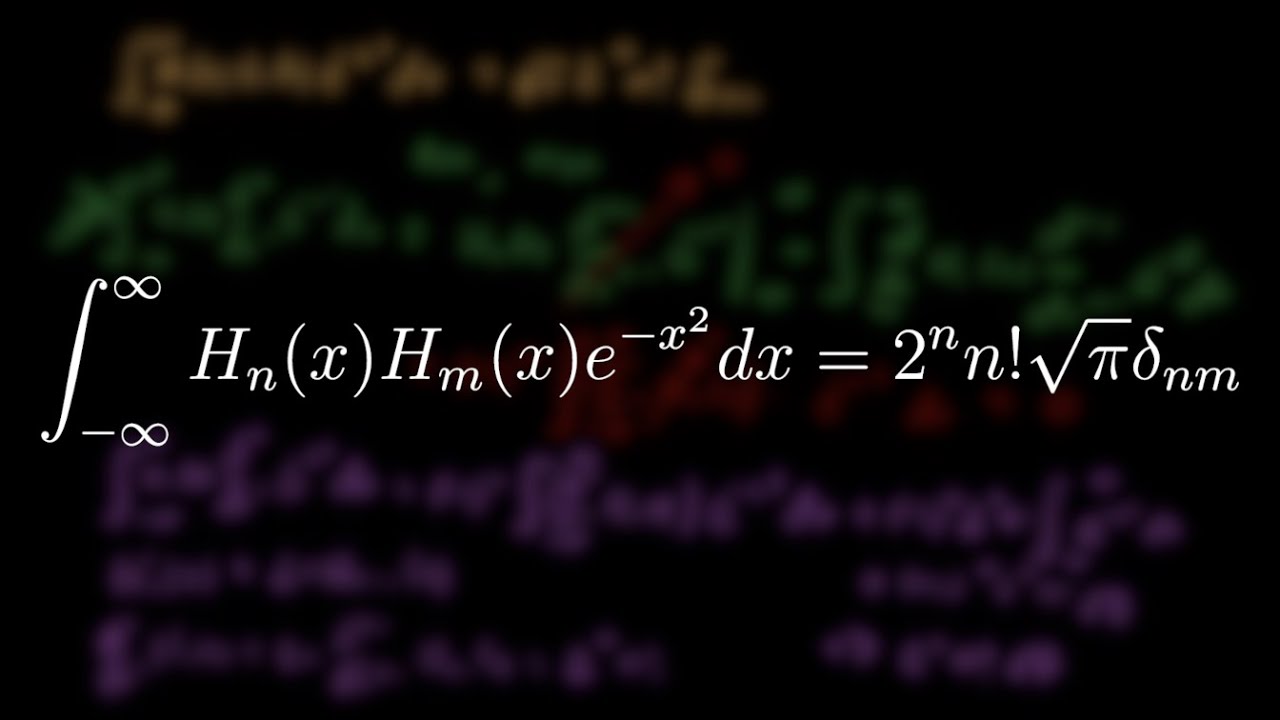
preview_player
Показать описание
In this video I prove that Hermite polynomials are orthogonal with respect to a weighted inner product.

For more videos in this series, visit:
  1. Physics and Math Lectures
  2. Hermite
  3. Hermite Polynomial
  4. Orthogonal
  5. Orthogonality
Рекомендации по теме
The Orthogonality of 0:09:51

The Orthogonality of Hermite Polynomials

Orthogonality between Hermite 0:08:14

Orthogonality between Hermite polynomials

orthogonality relation of 0:09:03

orthogonality relation of hermite polynomials

Orthogonality of Hermite 0:33:30

Orthogonality of Hermite Polynomial

Prove that the 0:24:50

Prove that the Hermite Polynomials are Orthogonal with respect to e^-x^2

The most useful 0:20:26

The most useful polynomials you have never heard of.

ORTHOGONAL PROPERTY OF 0:18:37

ORTHOGONAL PROPERTY OF HERMITE POLYNOMIAL | ORTHOGONALITY OF HERMITE POLYNOMIAL | WITH EXAM NOTES |

Orthogonality of hermite 0:18:09

Orthogonality of hermite polynomial/showing hermite Polynomials are orthogonal/normality condition..

Mathematical Physics- Special 0:26:41

Mathematical Physics- Special Functions: Hermite Polynomials- Orthogonal Properties

Hermite Polynomial Orthogonality 0:06:24

Hermite Polynomial Orthogonality proof

Orthogonal property of 0:27:17

Orthogonal property of hermite polynomials. (MATH)

orthogonality of hermite 0:07:38

orthogonality of hermite polynomials in hindi

Orthogonality of Hermite 0:34:24

Orthogonality of Hermite Polynomials

Introduction to Hermite 0:06:14

Introduction to Hermite Polynomials

Mathematical Physics Lecture28: 0:20:53

Mathematical Physics Lecture28: Hermite polynomials from orthogonality condition

Rodrigues and orthogonal 0:17:21

Rodrigues and orthogonal for hermite

Rodrigues Formula for 0:10:21

Rodrigues Formula for Hermite polynomials and Orthogonality

Orthogonality Relation For 0:11:31

Orthogonality Relation For Hermite Polynomial Hn(x) || Lecture No 17 || Bengali

orthogonal property of 0:00:16

orthogonal property of hermite polynomial

Normalisation of Hermite 0:12:59

Normalisation of Hermite polynomials

mod05lec46 - Hermite 0:22:37

mod05lec46 - Hermite polynomials

Orthogonal prop Hermite 0:05:36

Orthogonal prop Hermite polynomial

Degree Mathematics || 0:13:14

Degree Mathematics || Special Functions || Hermite polynomial -3

Orthogonal property of 0:00:10

Orthogonal property of hermite polynomial notes 🥰🥰

Комментарии
Автор

When I figured this out before dinner yesterday, I thought I was wrong because what if m < m? This video pointed out to me that m and n can switched so I had figured the solution correctly all along.

MisterTutor
Автор

I screamed at my computer at how easy that was. I tried to do it unsuccessfully on my own for a Thank you so much.

FunnCubes
Автор

Great Video and thank you for the clear explanation!

sanderscheel
Автор

At 5:29, why is it just exp(- x^2)? I know the result will still be 0, but not quite following that jump. Thanks

ventrue
Автор

One question, why the evaluation of the first term is 0 because of the gaussian? We don't have only to take into account the infinity but the central part, where the density is concentred

MrMagraden
Автор

you are assuming H_n is poly in the limit, but was that shown?

captainfartolini
Автор

why can you rewrite the hermitian pol as a derivative
?

domenicagarzon